Direction of friction for three bodies stacked one on top of the other

[lorenz0]

Homework Statement

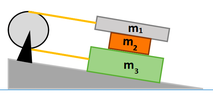
Three boxes of mass $m_1=10\ kg$, $m_2=5\ kg$ and $m_3= 30\ kg$ are arranged as shown in the figure and move by sliding down the inclined plane ($m_3$) and one on the other ($m_1$ and $m_2$).
The dynamic friction coefficient between the case of mass $m_3$ and the inclined plane is $\mu_{d3} =0.15$ while the dynamic friction coefficient between the $m_2$ and the other two boxes is $\mu_d = 0.10$.
Find the acceleration of the boxes and the tension of the rope assuming that the rope is ideal and the pulley of negligible mass. Assume that the plane is inclined by an angle $\alpha= \frac{\pi}{6}$ and stationary with respect to the ground and that the boxes, do not fall over but remain one on top of the other during the observation period.

Relevant Equations

$\sum_{i}\vec{F}_i=m\vec{a}$, $F_{fr}=\mu_d N$

I have drawn three free body diagrams, one for each box and then I applied Newton's Second Law after choosing a reference frame rotated clockwise by $\alpha$, with $x$ pointing south-east and $y$ pointing north-east and I got:

$\begin{cases}m_{1x}: -T+m_1g\sin(\alpha)+F_{fr_{12}}=m_1 (-a)\\ m_{2x}: -F_{fr_{12}}+F_{fr_{23}}+m_2g\sin(\alpha)=m_2 a_2\\ m_{3x}: -T-F_{fr_{23}}-F_{fr_3}+m_3g\sin(\alpha)=m_3 a\\ m_{1y}: N_1-m_1g\cos(\alpha)=0,\ |F_{fr_{12}}|=\mu_d N_1\\ m_{2y}: N_2-N_1-m_2g\cos(\alpha)=0,\ |F_{fr_{23}}|=\mu_d N_2\\ m_{3y}: N_3-N_2-m_3g\cos(\alpha)=0,\ |F_{fr_{3}}|=\mu_{d3}N_3\end{cases}$

where $F_{fr_{ij}}$ denotes the friction force between objects $i$ and $j$, $a_1=a_2=a, T_1=T_3=T$ since the rope is ideal and the pulley of negligible mass.

Now, I checked my solution against that of the text I am learning from and the text says that $F_{fr_{23}}$ on $m_2$ should point to the left and on $m_3$ to the right!

It says (all other equations coinciding with mine) that for $m_2$ and $m_3$ we have:
\begin{cases}
m_{2x}: -F_{fr_{12}}-F_{fr_{23}}+m_2g\sin(\alpha)=m_2 a_2\\ m_{3x}: -T+F_{fr_{23}}-F_{fr_{12}}+m_3g\sin(\alpha)=m_3 a
\end{cases}

How is that possible? As far as I can see as soon as the system is set in motion $m_3$ should go down the inclined plane and so the friction force with $m_2$ should prevent it from sliding, isn't it? If this is not the case then it makes me question the method I have always used up to now to find the direction of the friction: I try to see where the point of contact "is trying to move to" and I know that the friction force points in the opposite direction... is there another (better) way to do this? Thanks.

 

[haruspex]

in your first three equations you have not been consistent wrt signs.
You started off taking each new force and acceleration (except for T) as positive down the slope. In the third, you have taken friction from the ground on m₃ as positive up the slope (ok, but confusing) but more seriously you have both m₁ and m₃ with acceleration a down the slope.

Another problem is your F_f=μN equations. Since you don't yet know which way friction acts, these should be |F_f|=μN.

 

[lorenz0]

in your first three equations you have not been consistent wrt signs.
You started off taking each new force and acceleration (except for T) as positive down the slope. In the third, you have taken friction from the ground on m₃ as positive up the slope (ok, but confusing) but more seriously you have both m₁ and m₃ with acceleration a down the slope.

Thanks, I have corrected that and a couple of other typos.

 

[haruspex]

Thanks, I have corrected that and a couple of other typos.

See my edit.

 

[lorenz0]

See my edit.

The problem is that I thought I had gotten the correct signs for the friction forces between the boxes.

 

[haruspex]

The problem is that I thought I had gotten the correct signs for the friction forces between the boxes.

Even my edit did not quite capture it. Originally you had assumed a₂ was strictly between a and -a. Now you are assuming only that it is not equal to either of those. I should have written |F_f|≤μN.

 

[lorenz0]

Even my edit did not quite capture it. Originally you had assumed a₂ was strictly between a and -a. Now you are assuming only that it is not equal to either of those. I should have written |F_f|≤μN.

Isn't that the condition for static friction? I assumed (and the book agrees with me) that the boxes are sliding relative to one another so I used the formula for dynamic friction which, if I am not mistaken, is (in magnitude) constant and equal to $F_{fr_d}=\mu_d N$.

 

[haruspex]

Isn't that the condition for static friction? I assumed (and the book agrees with me) that the boxes are sliding relative to one another so I used the formula for dynamic friction which, if I am not mistaken, is (in magnitude) constant and equal to $F_{fr_d}=\mu_d N$.

In practice, it is highly likely that the middle mass moves together with one of the other two, but since you are not given a static coefficient we must either assume it is the same or that it is all kinetic.

Consider the case where there is no friction. How would the three move?

 

[lorenz0]

In practice, it is highly likely that the middle mass moves together with one of the other two, but since you are not given a static coefficient we must either assume it is the same or that it is all kinetic.

Consider the case where there is no friction. How would the three move?

Considering only friction between $m_3$ and the inclined plane intuition tells me that m_2 should start sliding downwards with respect to $m_3$ so that's why the friction force on $m_2$ relative to $m_3$ should point to the left and, by Newton's third law, why the friction force on $m_3$ relative to $m_2$ should point downwards.

If I try to set up Newton's third law this way I get:
$\begin{cases} (1): -T+m_1 g\sin(\alpha)=m_1 (-a)\\ (2): m_2g\sin(\alpha)=m_2a_2\\ (3): -T+m_3g\sin(\alpha)-F_{fr_3}=m_3 a\\ (4): N_1-m_1g\cos(\alpha)=0\\ (5): N_2-N_1-m_2g\cos(\alpha)=0\\ (6): N_3-N_2-m_3g\cos(\alpha)=0\\ (7): F_{fr_3}=\mu_dN_3 \end{cases}$

$(8)=(3)-(1): g\sin(\alpha)(m_3-m_1)-F_{fr_3}=(m_3+m_1)a$;
$(9): (4)\to (5)\to (6): N_3=(m_1+m_2+m_3)g\cos(\alpha)$;
$(10): (9)\to (7)\to (8): a=\frac{1}{m_3+m_1}\left( (m_3-m_1)g\sin(\alpha)-\mu_d (m_1+m_2+m_3)g\cos(\alpha)\right)=\frac{9.8}{40}\left( 10-\frac{45\sqrt{3}}{2} \right)\approx 1.50\ \frac{m}{s^2}$

while, from $(2)$, we get $a_2=g\sin(\alpha)=4.9\ \frac{m}{s^2}$.
Now, this result also appears to suggest that $m_2$ will slide downwards to the right and so the friction force between $m_2$ and $m_3$ is as I said in the above paragraph.

I think I understand what the boxes are doing now. Is my reasoning correct? Thanks.

 

[erobz]

Rookie question here. I was under the impression that you don't have to choose the direction of the frictional forces correctly so long as you are consistent in applying Newtons 3rd law between bodies for which ever direction you do choose?

i.e. if you choose $\rightarrow$ for $f_{r_{21}}$ you must choose $\leftarrow$ for $f_{r_{12}}$. In the end you will have the directions revealed to you w.r.t the directions you chose?

 

[haruspex]

Rookie question here. I was under the impression that you don't have to choose the direction of the frictional forces correctly so long as you are consistent in applying Newtons 3rd law between bodies for which ever direction you do choose?

Quite so, but the relationship between normal force and kinetic frictional force is $|F_f|=|N|\mu_k$. Assuming N is chosen positive in its actual direction, that simplifies to $|F_f|=N\mu_k$.

 

[haruspex]

Considering only friction between $m_3$ and the inclined plane intuition tells me that m_2 should start sliding downwards with respect to $m_3$ so that's why the friction force on $m_2$ relative to $m_3$ should point to the left and, by Newton's third law, why the friction force on $m_3$ relative to $m_2$ should point downwards.

If I try to set up Newton's third law this way I get:
$\begin{cases} (1): -T+m_1 g\sin(\alpha)=m_1 (-a)\\ (2): m_2g\sin(\alpha)=m_2a_2\\ (3): -T+m_3g\sin(\alpha)-F_{fr_3}=m_3 a\\ (4): N_1-m_1g\cos(\alpha)=0\\ (5): N_2-N_1-m_2g\cos(\alpha)=0\\ (6): N_3-N_2-m_3g\cos(\alpha)=0\\ (7): F_{fr_3}=\mu_dN_3 \end{cases}$

$(8)=(3)-(1): g\sin(\alpha)(m_3-m_1)-F_{fr_3}=(m_3+m_1)a$;
$(9): (4)\to (5)\to (6): N_3=(m_1+m_2+m_3)g\cos(\alpha)$;
$(10): (9)\to (7)\to (8): a=\frac{1}{m_3+m_1}\left( (m_3-m_1)g\sin(\alpha)-\mu_d (m_1+m_2+m_3)g\cos(\alpha)\right)=\frac{9.8}{40}\left( 10-\frac{45\sqrt{3}}{2} \right)\approx -7.1\ \frac{m}{s^2}$

while, from $(2)$, we get $a_2=g\sin(\alpha)=4.9\ \frac{m}{s^2}$.
Now, this result, while it seems absurd relative to the pair $m_1$, $m_3$ also appears to suggest that $m_2$ will slide downwards to the right and so the friction force between $m_2$ and $m_3$ is as I said in the above paragraph.

I think I understand what the boxes are doing now. Is my reasoning correct? Thanks.

you seem to have dropped $\mu_d$ in your evaluation of (10).
But if it were to turn out that a is negative, it would mean your equation 7 is wrong. Either the system is static or 7 should have the sign flipped (see post #11).

 

[haruspex]

I would approach this by allowing for static friction and assuming it equals the kinetic value (it cannot be less). Then I would consider the ways m2 might move in relation to m1 and m3. A priori, there are five:

1. Moves up faster than m1
2. Moves with m1
3. Moves up less than m1 but down less than m3
4. Moves down with m3
5. Moves down faster than m3.

One can be discounted immediately. Another is very unlikely, requiring a precise combination of parameters (and produces an indeterminate result).
Start with the middle of the remaining possibilities. If it violates one of the friction limits, that should point the way to the correct case.

Edit:
Thinking that through again, it is easy to show that m2 moves down.

 

[lorenz0]

you seem to have dropped $\mu_d$ in your evaluation of (10).
But if it were to turn out that a is negative, it would mean your equation 7 is wrong. Either the system is static or 7 should have the sign flipped (see post #11).

I computed (10) again including $\mu_d$ and I got $a=1.50\ \frac{m}{s^2}$ so it appears that indeed $m_2$ is sliding relative to $m_3$. Thanks again for your help.