Poetria
- 267
- 42
- Homework Statement
- h(x,y)=x*y
x,y, h are measured in meters
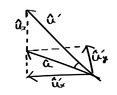
A guy starts walking at the point (2,1) and continues in a straight line to (0,3).
As he starts, he is walking uphills if I understand it correctly.
The slope at the moment he starts is required.
Then a unit vector which is tangent to the level curve of h at height 2 at the point (2,1).
Finally - directional derivative ##D_\vec u h(2,1)##
- Relevant Equations
- $$y'=-\frac {F_x} {F_y}$$
$$h_x=y$$
$$h_y=x$$
Substituting the coordinates of a given point:
$$y'=-\frac {y} {x}$$
$$y'=-\frac {1} {2}$$
A unit vector:
$$\frac {1} {\sqrt{5}, \frac {2} {\sqrt{5}}$$
$$D_\vec u h(2,1) = \frac {1} {\sqrt{5}, \frac {2} {\sqrt{5}} \cdot \vec (1,2)$$
$$D_\vec u h(2,1) = \frac {5} {\sqrt{5}}$$
$$h_y=x$$
Substituting the coordinates of a given point:
$$y'=-\frac {y} {x}$$
$$y'=-\frac {1} {2}$$
A unit vector:
$$\frac {1} {\sqrt{5}, \frac {2} {\sqrt{5}}$$
$$D_\vec u h(2,1) = \frac {1} {\sqrt{5}, \frac {2} {\sqrt{5}} \cdot \vec (1,2)$$
$$D_\vec u h(2,1) = \frac {5} {\sqrt{5}}$$
Last edited: