Discharging a capacitor to to charge multiple capacitors

[Biker]

Homework Statement

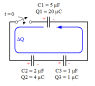
Assume you have C1, C2, C3 capacitors and each one of them have a specific charge. Now imagine you connect them to one circuit (Just as in the picture below). Determine the charge on each capacitor after the movement of charges stop

Homework Equations

Kirchhoff's law

The Attempt at a Solution

So when I started solving these problems, I came out with a good solution for these problems.
Now the basic solution for a charged capacitor to charge another capacitor is to consider those in parallel, But here we just cant.

So my solution has always showed the right answers, I wonder if it does apply here too.
Basically, you show that one of the capacitors (c1) has a voltage bigger than the sum of others thus a current will pass. Now I can say that Q charges will move in the whole period and with the indicated polarities above I can say that C1's charges decreases by Q and C2's charge increase by Q and C3's charges increase by Q

Now by using conservation of energy or Kirchhoff's 2nd law (voltage law) you can actually determine Q
and you can then get the charge of each capacitor after charging.

It gave sensible answer, that gave me a round trip of zero

 

[gneill]

Conservation of energy is not likely to be very useful here. When capacitors redistribute charge there's always a net loss of energy. KVL on the other hand will work quite nicely.

So, did you have a question? Did you make an attempt to solve the given problem that you can show us?

 

[Biker]

Conservation of energy is not likely to be very useful here. When capacitors redistribute charge there's always a net loss of energy. KVL on the other hand will work quite nicely.

So, did you have a question? Did you make an attempt to solve the given problem that you can show us?

I just meant conservation of energy as in KVL
The words above explains the mathematical approach I used to solve the problem, Anyway here is it:
$\frac{Q_1-Q}{C_1} = \frac{Q_2 + Q}{C_2} + \frac{Q_3 + Q}{C_3}$
Isolate Q:
$Q = \frac{((\frac{Q_1}{C_1} - \frac{Q_2}{C_2} - \frac{Q_3}{C_3}) C_1 ~ C_2 ~ C_3)}{C_3C_2 + C_3C_1 + C_1C_2}$

You get charge Q where it represents the amount of charge transferred or moved.
Then you can determine the charges on each capacitor
$Q_1 = 1.941176\text{x} 10^{-5}$
$Q_2 = 4.58826 \text{x} 10^{-6}$
$Q_3 = 1.588235 \text{x} 10^{-6}$

 

[haruspex]

you show that one of the capacitors (c1) has a voltage bigger than the sum of others

You can omit that step. Just define the change in charge as positive in a particular direction. If you guessed wrong, you will get a negative result.

 

[Biker]

You can omit that step. Just define the change in charge as positive in a particular direction. If you guessed wrong, you will get a negative result.

So the solution is correct right? are there other solutions?

And about parallel and series, You can't just solve using that in anyway right? Just ensuring.

 

[gneill]

So the solution is correct right? are there other solutions?

I don't get the same results, although your initial KVL equation looks okay. One or both of us may have mucked up the arithmetic There will be only one solution.

Perhaps you can supply a bit more detail for the calculations of your solution? What value did you find for Q? How did you determine the new capacitor Q's?

And about parallel and series, You can't just solve using that in anyway right? Just ensuring.

I imagine that you could combine capacitors to reduce the circuit, but you'd have to preserve the net charge and potential difference with each reduction. I haven't tried that. In this case since you're looking for the final charge on each of the original capacitors, you wouldn't want to "lose" access any of them by reducing the circuit. There is an alternative though:

You can form an equivalent circuit to determine the amount of charge that moves (your Q). Initially each charged capacitor can be modeled as a fixed potential difference in series with an empty capacitor, the PD being the initial PD across the capacitor due to the initial charge it holds. When you do this for all the capacitors in your circuit you'll notice that since they are series-connected you can sum up the PD's around the loop to obtain the net PD that can drive current, and sum the "empty" capacitors in series to obtain a net capacitance. So, one fixed PD and one capacitor. The charge on that capacitor due to the PD will be the amount of charge that moves in your circuit (your Q).

 

[Biker]

I don't get the same results, although your initial KVL equation looks okay. One or both of us may have mucked up the arithmetic There will be only one solution.

Perhaps you can supply a bit more detail for the calculations of your solution? What value did you find for Q? How did you determine the new capacitor Q's?

I imagine that you could combine capacitors to reduce the circuit, but you'd have to preserve the net charge and potential difference with each reduction. I haven't tried that. In this case since you're looking for the final charge on each of the original capacitors, you wouldn't want to "lose" access any of them by reducing the circuit. There is an alternative though:

You can form an equivalent circuit to determine the amount of charge that moves (your Q). Initially each charged capacitor can be modeled as a fixed potential difference in series with an empty capacitor, the PD being the initial PD across the capacitor due to the initial charge it holds. When you do this for all the capacitors in your circuit you'll notice that since they are series-connected you can sum up the PD's around the loop to obtain the net PD that can drive current, and sum the "empty" capacitors in series to obtain a net capacitance. So, one fixed PD and one capacitor. The charge on that capacitor due to the PD will be the amount of charge that moves in your circuit (your Q).

Rip Arithmetic.

From my equation above
$Q = \frac{1}{1700000} (4-2-1)$
$Q = 5.8823\text{x}10^{-7}$
$Q^{'}_1 = Q_1 - Q$
$Q^{'}_1 = 20\text{x}10^{-6} - 5.8823\text{x}10^{-7}$
$Q^{'}_2 = Q_2 + Q$
$Q^{'}_2 = 4\text{x}10^{-6} + 5.8823\text{x}10^{-7}$
$Q^{'}_3 = Q_3 + Q$
$Q^{'}_3 = 1\text{x}10^{-6} + 5.8823\text{x}10^{-7}$

And the results are the same, Probably I might have made a mistake not sure I checked them again.And for the simplification, Awesome idea! We can prove that to be the case through the equation we got above. Didn't notice that :D

About Parallel and series, Not sure if I can do anything about it. All capacitors have different charges and I can't take them as Parallel because they don't have the same voltage

 

[gneill]

Hmm. According to your circuit and the equations you wrote, shouldn't Q1 lose charge Q, not gain charge Q? And both Q2 and Q3 should increase by Q.

About Parallel and series, Not sure if I can do anything about it. All capacitors have different charges and I can't take them as Parallel because they don't have the same voltage

Well in this case they're all in series anyways, unless you combine two of them in series first. You could reduce C2 and C3 to a single capacitor. Just conserve the net PD of the two on your "new" capacitor by giving it a suitable charge.

 

[Biker]

Hmm. According to your circuit and the equations you wrote, shouldn't Q1 lose charge Q, not gain charge Q? And both Q2 and Q3 should increase by Q.
Well in this case they're all in series anyways, unless you combine two of them in series first. You could reduce C2 and C3 to a single capacitor. Just conserve the net PD of the two on your "new" capacitor by giving it a suitable charge.

Sorry yea fixed it, Just messed the number of each Charge. But still the arithmetic is the same

 

[haruspex]

Sorry yea fixed it, Just messed the number of each Charge. But still the arithmetic is the same

I get the same numbers as you do. I used the same principle but not your equation.