- #1
AntonVrba
- 92
- 0
I would like to invite comment to the near step function below.
[tex]
\Mvariable{step(x)}=\frac{{e^{-\frac{\gamma }{{x^2}}}}}{{\sqrt{1-\frac{{e^{-\frac{\gamma }{{x^2}}}}}{{x^2}}}}}
[/tex]
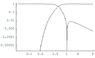
the above function evaluates to nearly 1 for |x|>1 and nearly zero for |x|<0.3
for gamma = 0.4823241136337762 I have attached the plots of step and 1-step
here are some spot values for
x and step(x)
0.05 1.15E-83
0.1 1.84E-21
0.2 6.55E-6
0.3 5.11E-3
0.4 6.11E-2
0.5 2.32E-1
0.6 0.518
0.8 0.932
1.0 1.0068
2.0 1.006
5.0 1.00092
10 1.00022
50 1.0000090
[tex]
\Mvariable{step(x)}=\frac{{e^{-\frac{\gamma }{{x^2}}}}}{{\sqrt{1-\frac{{e^{-\frac{\gamma }{{x^2}}}}}{{x^2}}}}}
[/tex]
the above function evaluates to nearly 1 for |x|>1 and nearly zero for |x|<0.3
for gamma = 0.4823241136337762 I have attached the plots of step and 1-step
here are some spot values for
x and step(x)
0.05 1.15E-83
0.1 1.84E-21
0.2 6.55E-6
0.3 5.11E-3
0.4 6.11E-2
0.5 2.32E-1
0.6 0.518
0.8 0.932
1.0 1.0068
2.0 1.006
5.0 1.00092
10 1.00022
50 1.0000090
Attachments
Last edited: