Disk with rod attached rotating about the center of the disk

[lorenz0]

Homework Statement

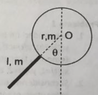
A disk of mass $m$ and radius $r$ is free to rotate around an axis passing through its center O. A rod of mass $m$ and length $l$ is attached radially to the edge of the disk at an angle $\theta$. Find:
1) The moment of inertia with respect to the center O and the position of the center of mass (also with respect to O).
If the system is left free to move starting from rest from the angular position $\theta=\pi/2$, find, for a generic angle $0\leq \theta\leq \frac{\pi}{2}$:
2) The formula for $\frac{d\theta}{dt}$ as a function of $\theta$;
3) The formula for $\frac{d^2\theta}{dt^2}$ as a function of $\theta$;
4) The vector acceleration $\vec{a}_{CM}$ of the center of mass and the vector $\vec{N}$, the reaction force of the axis.

Relevant Equations

$I=\int r_{\perp}^2 dm,\ \vec{r}_{cm}=\frac{\int \vec{r}dm}{M}$

1) Since the rod is uniform, with mass m and length l, it has a linear mass density of $\lambda=\frac{m}{l}$, so $I_{rod_O}=\int_{x=r}^{x=r+l}x^2 \lambda dx=\frac{\lambda}{3}[(r+l)^3-r^3]=\frac{\lambda r^3}{3}[(1+\frac{l}{r})^3-1]=\frac{1}{3}mr^2[3+\frac{3l}{r}+\frac{l^2}{r^2}].$
$I_{O}=I_{disk_O}+I_{rod_O}=\frac{1}{2}mr^2+\frac{1}{3}mr^2[3+\frac{3l}{r}+\frac{l^2}{r^2}]=mr^2[\frac{3}{2}+\frac{l}{r}+\frac{l^2}{3r^2}].$

$\vec{r}_{cm}=\frac{1}{2m}(-(r+\frac{l}{2})\sin(\theta), -(r+\frac{l}{2})\cos(\theta))=-\frac{2r+l}{4}(\sin(\theta),\cos(\theta))$ so $r_{cm}=\frac{2r+l}{4}.$

Now, the problem is that I don't understand how to find $\omega=\frac{d\theta}{dt}$ and $\alpha=\frac{d^2\theta}{dt^2}$ as a function of $\theta$. What I have done is: $\tau_O=I_O\alpha \Leftrightarrow - r_{cm}2mg\sin(\theta)=mr^2[\frac{3}{2}+\frac{l}{r}+\frac{l^2}{3r^2}] \frac{d^2\theta}{dt^2}$ so $\frac{d^2\theta}{dt^2}=-\frac{2r+l}{4r^2[\frac{3}{2}+\frac{l}{r}+\frac{l^2}{3r^2}]}g\sin(\theta)$ but I don't see how to go from this to $\frac{d\theta}{dt}$ (even knowing the initial conditions $\theta(0)=\frac{\pi}{2}, \omega(0)=0$), since there is a $\sin(\theta)$ involved in the integration, where $\theta=\theta(t)$.

So, I would be grateful if someone could help me understand how to find these two quantities, thanks.

------
By knowing $\omega$ and $\alpha$ then I can find $\vec{a}_{cm}=\omega^2 r_{cm}(-\hat{r})+r_{cm}\alpha (-\hat{\theta})=\frac{4mgr_{cm}\cos(\theta)}{I_O} r_{cm} (-\hat{r})+\frac{r^2_{cm} mg\sin(\theta)}{I_O}(-\hat{\theta})$.

 

[TSny]

Now, the problem is that I don't understand how to find $\omega=\frac{d\theta}{dt}$ ... as a function of $\theta$.

There's a conserved quantity that might be helpful here.

 

[lorenz0]

There's a conserved quantity that might be helpful here.

$2mgr_{cm}=\frac{1}{2}I_O\omega^2+2mg(r_{cm}-r_{cm}\cos(\theta))\Leftrightarrow \omega=\sqrt{\frac{4mgr_{cm}\cos(\theta)}{I_O}}=\sqrt{\frac{4mg\frac{2r+l}{4}\cos(\theta)}{mr^2(\frac{3}{2}+\frac{l}{r}+\frac{l^2}{3r^2})}}=\sqrt{\frac{6(2r+l)}{9r^2+6lr+2l^2}g\cos(\theta)}$

 

[TSny]

$mgr_{cm}=\frac{1}{2}I_O\omega^2+mg(r_{cm}-r_{cm}\cos(\theta))$

Looks pretty good. But, does $m$ here represent the total mass or the mass of just the rod or disk?

$\Leftrightarrow \omega=\sqrt{\frac{2mgr_{cm}(1-\cos(\theta))}{I_O}}$ ?

Check your work. I think there's a mistake in solving for $\omega$. What does this equation give for $\omega$ when $\theta = \pi/2$?

 

[lorenz0]

Looks pretty good. But, does $m$ here represent the total mass or the mass of just the rod or disk?Check your work. I think there's a mistake in solving for $\omega$. What does this equation give for $\omega$ when $\theta = \pi/2$?

It should be correct now, thanks.

 

[TSny]

It should be correct now, thanks.

Looks good except for a missing $g$ in the final expression.

 

[lorenz0]

Looks good except for a missing $g$ in the final expression.

Corrected, thanks. Could you please tell me what you think of the way I solved question (4) about the acceleration of the CM and the reaction of the axis?

 

[TSny]

By knowing $\omega$, then I can find $\vec{a}_{cm}=\omega^2 r_{cm}(-\hat{n})+g\sin(\theta)\hat{t}=...$

How did you get $g\sin(\theta)\hat{t}$ for the tangential acceleration of the center of mass? This would imply that when the system is released at $\theta = \pi/2$, the acceleration of the center of mass would be $g \hat{t}$, which is not correct.

 

[lorenz0]

How did you get $g\sin(\theta)\hat{t}$ for the tangential acceleration of the center of mass? This would imply that when the system is released at $\theta = \pi/2$, the acceleration of the center of mass would be $g \hat{t}$, which is not correct.

Just by considering the free body diagram of the CM of the object at an intermediate angle $0\leq\theta\leq\frac{\pi}{2}$: looking back at it, I think it should be $g\sin(\theta) (-\hat{t})$.

 

[TSny]

The motion of the center of mass is determined by the net external force according to $\vec F_{net}^{ext} = M \vec a_{cm} \,$, where $M$ is the total mass of the system. The force of gravity is not the only external force acting on this system.

You should be able to express the tangential acceleration of the center of mass in terms of $r_{cm}$ and $\ddot \theta$.

 

[lorenz0]

The motion of the center of mass is determined by the net external force according to $\vec F_{net}^{ext} = M \vec a_{cm} \,$, where $M$ is the total mass of the system. The force of gravity is not the only external force acting on this system.

You should be able to express the tangential acceleration of the center of mass in terms of $r_{cm}$ and $\ddot \theta$.

$\vec{a}_{CM}=\omega^2 r_{cm} (-\hat{n}) + \alpha r_{cm} (-\hat{t})=\frac{4mgr^2_{cm}cos(\theta)}{I_O}(-\hat{n})+\frac{-r^2_{cm}mg\sin(\theta)}{I_O}(-\hat{t})$

$\vec{N}+2m\vec{g}=2m\vec{a}_{cm}\Leftrightarrow \vec{N}=-2m\vec{g}+2m\vec{a}_{cm}$

 

[TSny]

That looks pretty good to me. I think there might be some small errors due to not distinguishing the mass $m$ of the disk (or rod) and the total mass $M$ of the system. What does the symbol $m$ represent in each occurrence of your expressions above? Check to see if the last term of your expression for $\vec a_{CM}$ should have $2m$ instead of $m$.

I'm also not sure of your choice of direction of $\hat t$. Is it in the direction of increasing $\theta$ or decreasing $\theta$?

 

[kuruman]

Check to see if the last term of your expression for $\vec a_{CM}$ should have $2m$ instead of $m$.

The mass of the disk and the mass of the rod are each equal to $m$ so $2m$ is correct for the reaction force when the acceleration is zero.

 

[TSny]

$\vec{a}_{CM}=\omega^2 r_{cm} (-\hat{n}) + \alpha r_{cm} (-\hat{t})=\frac{4mgr^2_{cm}cos(\theta)}{I_O}(-\hat{n})+\frac{-r^2_{cm}mg\sin(\theta)}{I_O}(-\hat{t})$

$\vec{N}+2m\vec{g}=2m\vec{a}_{cm}\Leftrightarrow \vec{N}=-2m\vec{g}+2m\vec{a}_{cm}$

The second equation now looks correct.

However, I still think you need a factor of 2 in the numerator of the last term in the first equation. If you go back to your first post, you had

$\tau_O=I_O\alpha \Leftrightarrow - r_{cm}2mg\sin(\theta)$

------
$\vec{a}_{cm}=\omega^2 r_{cm}(-\hat{r})+r_{cm}\alpha (-\hat{\theta})=\frac{4mgr_{cm}\cos(\theta)}{I_O} r_{cm} (-\hat{r})+\frac{r^2_{cm} mg\sin(\theta)}{I_O}(-\hat{\theta})$.

The first equation looks correct (with the $2m$ in the last term of the right side). But this factor of 2 seems to be missing in the numerator of the last term of the right side of the second equation above.