Displacement as Function of Time Graph Question

In summary, After receiving feedback and advice, the velocity and acceleration graphs were redrawn based on the original graph A. The velocity graph shows a decrease in slope before peaking, indicating a decrease in speed before hovering. The acceleration graph shows a slight positive slope throughout, indicating a constant acceleration. These graphs could represent a scenario where a helicopter hovers at a certain height before descending. However, labeling of axes and titles should be included for accuracy.
  • #1
physicsnobrain
123
0

Homework Statement


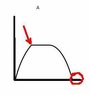
The graph below shows the vertical displacement of an object as a function of time.
(a) Construct a graph of the object’s velocity as a function of time.
(b) Construct a graph of the object’s acceleration as a function of time.
(c) Describe a scenario in which a real-life object might move in this way


The Attempt at a Solution



Ok, Here is a picture. Graph A represents the original graph that we construct B and C from. I am not sure if I drew graph B (velocity as a function of time) and C (acceleration as a function of time) correctly. Did I?
15f4y6r.png


Also for a potential scenario, I can't come up with anything except for maybe a helicopter that goes up to a certain height, then hovers at that height then comes back down. But I'm not sure about the curvatures on that original graph A so I want to make the scenario detailed.

Thanks!
 
Physics news on Phys.org
  • #2
Before I say anything useful I will say that it has been a very long time since I've looked at position (or velocity or acceleration) vs. time graphs so it's possible that I am wrong but: I think your graphs are fine (but you shouldn't trust me entirely).

One thing I will mention is that you should aim to provide titles and label your axes for ease of other readers (ie. us "helpers" or your teacher). Some (many?) teachers will be a stickler for such things and it will be easy to lose marks in a silly way if you don't label your axes carefully.

As for a scenario, I believe the one you have described works well. We could try to be slightly more detailed. On the position vs. time graph, prior to peaking we notice that the slope decreases slightly - this means that the velocity is somewhat decreased.

We can explain this in the following way: A helicopter lifts up off of the ground and moves upwards with some speed. At a certain height the pilot decides to slow his ascent and hover in the air at some height. Then he descends in a similar fashion. (This is basically what you said).

We could make this more elaborate.

Suppose we are on a planet with trees that grow very, very tall. One day, your kitten, Paws, decides to climb one of these trees (she is chasing a delicious looking bird-like creature) and gets stuck very high up. You contact the emergency services and the send a rescue helicopter to save her. The pilot flies his helicopter up and as he approaches the location of Paws he begins to slow down and hover in mid-air when he is near. The passenger, a professional cat rescuer rescues Paws and brings her on board the helicopter and the pilot begins the descent again.
 
  • #3
physicsnobrain said:
The graph below shows the vertical displacement of an object as a function of time.
(a) Construct a graph of the object’s velocity as a function of time.

Hi physicsnobrain. http://img96.imageshack.us/img96/5725/red5e5etimes5e5e45e5e25.gif

Take a ruler and lay it to be tangential at some point on your plot of distance vs time, the steepness of the incline of the ruler here indicates the velocity of the moving object at that moment. The steeper the slope of ruler, the greater the speed.

Slowly move the ruler along the curve, adjusting it so it stays tangential to the curve, and you get a clear picture of how the speed of the object is changing (or not changing, as the case may be). When the ruler's incline is steep, the speed is greater.

Ignore the graphs you have already sketched. Start anew and work out bit by bit how the velocity vs time graph should go.
 
Last edited by a moderator:
  • #4
NascentOxygen said:
Hi physicsnobrain. http://img96.imageshack.us/img96/5725/red5e5etimes5e5e45e5e25.gif

Take a ruler and lay it to be tangential at some point on your plot of distance vs time, the steepness of the incline of the ruler here indicates the velocity of the moving object at that moment. The steeper the slope of ruler, the greater the speed.

Slowly move the ruler along the curve, adjusting it so it stays tangential to the curve, and you get a clear picture of how the speed of the object is changing (or not changing, as the case may be). When the ruler's incline is steep, the speed is greater.

Ignore the graphs you have already sketched. Start anew and work out bit by bit how the velocity vs time graph should go.

Are the graphs I drew not correct? Also, since the velocity time is velocity as a function of time, if I drew it steep that would mean there is acceleration, which I believe there isn't judging from the graph A.
 
Last edited by a moderator:
  • #5
NascentOxygen said:
Hi physicsnobrain. http://img96.imageshack.us/img96/5725/red5e5etimes5e5e45e5e25.gif

Take a ruler and lay it to be tangential at some point on your plot of distance vs time, the steepness of the incline of the ruler here indicates the velocity of the moving object at that moment. The steeper the slope of ruler, the greater the speed.

Slowly move the ruler along the curve, adjusting it so it stays tangential to the curve, and you get a clear picture of how the speed of the object is changing (or not changing, as the case may be). When the ruler's incline is steep, the speed is greater.

Ignore the graphs you have already sketched. Start anew and work out bit by bit how the velocity vs time graph should go.

Ok, after taking your advice this is now the graph I get of velocity as a function of time constructed from the graph A in my first post.
34g7e9w.png


Is it right?
 
Last edited by a moderator:
  • #6
And here is the acceleration as a function of time graph.
msoh07.png


Again, is this right?
 
  • #7
Does anybody have an idea if my two new graphs work given graph A in my original post?
 
  • #8
physicsnobrain said:
Ok, after taking your advice this is now the graph I get of velocity as a function of time constructed from the graph A in my first post.
34g7e9w.png


Is it right?
That is looking better. Though we don't know that those sloping straight lines should actually be straight lines, it is better than your first post's graph (B).

The right hand side of your plot should not end like you show it, though.

EDITED
 
Last edited:
  • #9
NascentOxygen said:
That is looking better. Though we don't know that those sloping straight lines should actually be straight lines, it is better than your first post's graph (B).

The right hand side of your plot should not end like you show it, though.

EDITED

Is this better for velocity as function of time?
2i77gw1.png
 
  • #10
Also, would the acceleration just be a straight line at zero?
 
  • #11
physicsnobrain said:
Is this better for velocity as function of time?
2i77gw1.png
No, that is not better. A period of constant velocity would show on the displacement-time plot as a straight (though sloping) line. There are no such lines on the distance-time plot given for this question. What you had was good enough for now.

You have noticed how the distance-time graph ends with the object sitting at zero distance for some time?

Only when you have the velocity-time plot right is it worth discussing the acceleration.
 
  • #12
NascentOxygen said:
No, that is not better. A period of constant velocity would show on the displacement-time plot as a straight (though sloping) line. There are no such lines on the distance-time plot given for this question. What you had was good enough for now.

You have noticed how the distance-time graph ends with the object sitting at zero distance for some time?

Only when you have the velocity-time plot right is it worth discussing the acceleration.

2mq6p88.png


Ok I drew this velocity as a function of time graph. This is accurate, correct?
 
  • #13
Pay attention to two regions:

attachment.php?attachmentid=61863.jpg
 

Attachments

  • 15f4y6r.jpg
    15f4y6r.jpg
    4 KB · Views: 1,127
  • #14
NascentOxygen said:
Pay attention to two regions:

attachment.php?attachmentid=61863.jpg

That second region you circled shouldn't be there. It was a mistake on my part when i drew the graph in paint. Pretend that the graph ends when it hits the x axis.
 
  • #15
Okay. But how did you decide (on the right hand side of the plot) there is a sudden bend in the line halfway while the object returns to zero level?

The arrow points to a point in time where distance was rapidly changing with time then it abruptly stopped changing. That happens when speed has an abrupt drop to zero.
 
  • #16
Ok, I see the abrupt change. I went back to the graph I drew before and modified the second part.

oj42eb.png


Is this more like it?
 
  • #17
You have the first abrupt change to zero velocity correct, but you are still not showing the second one. When the distance graph finally returns to the t axis, it stays there, so velocity is now zero.
Also, because distance ends up where it started, at zero, the total area under the velocity graph (counting areas below the t axis as negative) should be zero. I.e. the total area above the t axis should be equal to the total area below it.
 
  • #18
physicsnobrain said:
Ok, I see the abrupt change. I went back to the graph I drew before and modified the second part.

oj42eb.png


Is this more like it?
The left hand half of the plot is looking good. Next, that 120 degree bend you show in the right hand half, why did you draw it like that?
 
  • #19
NascentOxygen said:
The left hand half of the plot is looking good. Next, that 120 degree bend you show in the right hand half, why did you draw it like that?

Well because there is no abrupt change in the second half of the graph, it flows.
 
  • #20
physicsnobrain said:
Well because there is no abrupt change in the second half of the graph, it flows.
So why did you show an abrupt change in the way velocity changes where there is no abrupt change in the way displacement is changing?

Anyway, you should get back to the graph you drew here. https://www.physicsforums.com/showpost.php?p=4503814&postcount=5

34g7e9w.png


Now that you cleared up the artifact I had circled, that graph from earlier is correct!

Now you can determine the acceleration vs time graph. (The one in your very first post is not correct.)
 
  • #21
Ok. Now to derive acceleration from this velocity time graph I will find the slope ((vf-vi)/(change in time)) of each segment of the graph.
 
  • #22
Here is what I get for the acceleration as a function of time graph.
155krc7.png
 
  • #23
You used a ruler like I suggested? Aligning it to be tangential to the graph as you slowly slide it from left to right. You won't quite get that graph.

A real life example that fits this trio of graphs would be throwing something like a pillow or a rolled up T-shirt from floor level (awkward, I know) upwards to hit the ceiling.
 
Last edited:
  • #24
physicsnobrain said:
Well because there is no abrupt change in the second half of the graph, it flows.
Are you sure? The graph you posted shows the displacement returning to zero and staying there, while the t axis continues briefly.
 
  • #25
Getting close to finding the correct answer now.
Here is V3.0 of velocity as a function of time:

23svtqt.png
 
  • #26
Still trying to work this out. First the object increases from 0 velocity to max velocity, then it instantly drops to zero velocity, then 0 velocity decreases to max negative velocity then it instantly hits zero.

2lsjjfr.png
 
  • #27
I have now concurred that it is 100% impossible to convert this graph from displacement - time to velocity - time. This is probably my final answer.
 
  • #28
Ok, this is my final attempt. If this is wrong, I give up.

2mw8nl2.png
 
  • #29
and here is the the graph of acceleration as a function of time I am sure of it.

2ytv0ib.png
 
  • #30
Ok after even further time analysis, I believe this to be the velocity as a function of time graph:
29ffdcy.png
 
  • #31
One of your previous responses was closest to the correct answer (#12).

Chet
 
  • #32
Chestermiller said:
One of your previous responses was closest to the correct answer (#12).

Chet

Ok, well then this has to be the right answer.

etxjzd.png
 
  • #33
physicsnobrain said:
Ok, well then this has to be the right answer.

etxjzd.png

This doesn't look anything like the graph in response #12.
 
  • #34
Ok. But at the end it accelerates slow then quickly accelerates. So should it be this?
3536h.png
 
  • #35
Here is what I'm getting for acceleration now:
2a9v485.png
 

Similar threads

Why would this be accelerating positively?
Replies
6
Views
504
Analyzing Motion: Deriving Displacement Graphs from First Principles
Replies
6
Views
1K
Distance-time graph of a ball throw vertically up from a fixed point
Replies
5
Views
1K
Finding acceleration from Velocity vs Position graph
Replies
2
Views
3K
Velocity, Acceleration and Displacement time graphs.
Replies
30
Views
3K
I need opinions on a Projectile Motion problem that I made up
Replies
11
Views
1K
Understanding a Velocity-Time Graph
Replies
14
Views
4K
Velocity-time graph given. Plot acceleration vs time etc.
Replies
1
Views
8K
Finding Displacement based on Velocity Graph
Replies
11
Views
2K
Distance Velocity Graph: What Does the Area Represent?
Replies
2
Views
1K

Hot Threads

Recent Insights

Back
Top