- #1
vel
- 26
- 0
- Homework Statement
- Standing sound waves are produced in a pipe that is 1.80 m long.
1) If the pipe is closed at the left end and open at the right end, determine the locations along the pipe (measured from the left end) of the displacement nodes for the first overtone.
2) If the pipe is closed at the left end and open at the right end, determine the locations along the pipe (measured from the left end) of the displacement nodes for the second overtone.
- Relevant Equations
- (4 / 3) * L = lambda
1st overtone: L / 4, (3 * L) / 4
2nd overtone: L / 6, (3*L) / 6, (5 * L) / 6
(4 / 3) * (1.8) = 2.4 = lambda
1st overtone: 2.4 / 4 = .6; (2.4 * 3) / 4 = 1.8
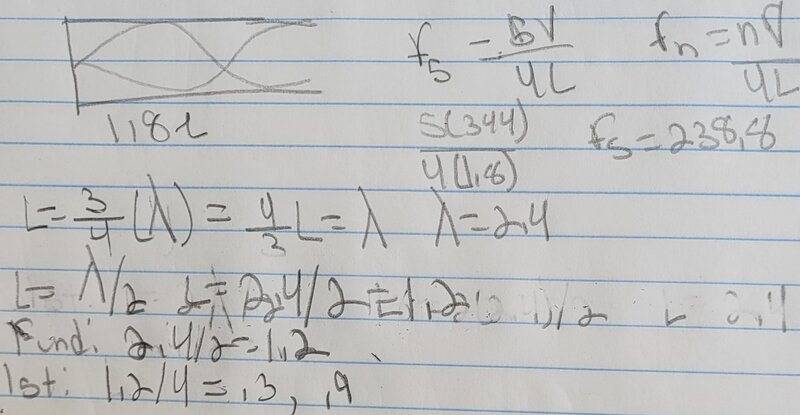
1st overtone: 2.4 / 4 = .6; (2.4 * 3) / 4 = 1.8
Last edited: