- #1
mesa
Gold Member
- 695
- 38
I have been looking at mass spectrometers, in particular the interactions between the Bf ind of a charged particle in motion in a static Bf of the spectrometer.
Figure 1 and 2 shown below are a basic 2 dimensional slice of the problem from the top (fig 1) and from the inside of the radius looking out (fig 2) to establish our F.o.R.
Figure 3 is a view of the charged particle moving towards us at velocity vq- with the 'direction' of the Bf ind shown along 8 symmetrically spaced points.
[URL=[PLAIN]http://s1332.photobucket.com/user/ian5576g/media/crossproductpage1_zps8daf6515.jpg.html][PLAIN]http://i1332.photobucket.com/albums/w606/ian5576g/crossproductpage1_zps8daf6515.jpg
Figure 4 below shows the interaction of the Bf ind with our static Bf along these 8 points and the resultant Force at those points. Figure 5 uses this information to draw a 'representative' magnitude for the Force along different points acting on our moving charged particle in the static Bf. To me this looks like the Force at these points would mediate a rotation about an axis drawn through a charged particle in the direction of 'y' if moving through a static Bf as shown, is this correct?
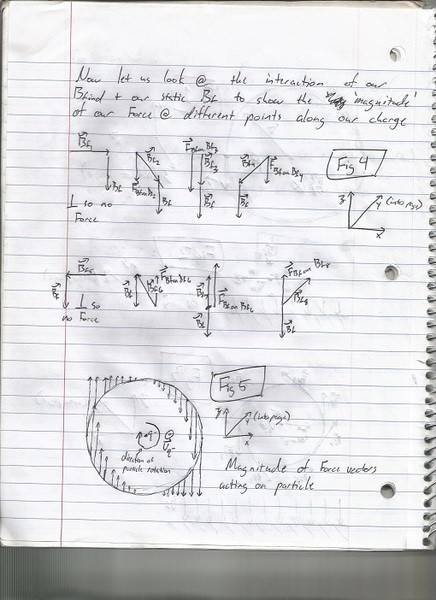
Figure 1 and 2 shown below are a basic 2 dimensional slice of the problem from the top (fig 1) and from the inside of the radius looking out (fig 2) to establish our F.o.R.
Figure 3 is a view of the charged particle moving towards us at velocity vq- with the 'direction' of the Bf ind shown along 8 symmetrically spaced points.
[URL=[PLAIN]http://s1332.photobucket.com/user/ian5576g/media/crossproductpage1_zps8daf6515.jpg.html][PLAIN]http://i1332.photobucket.com/albums/w606/ian5576g/crossproductpage1_zps8daf6515.jpg
Figure 4 below shows the interaction of the Bf ind with our static Bf along these 8 points and the resultant Force at those points. Figure 5 uses this information to draw a 'representative' magnitude for the Force along different points acting on our moving charged particle in the static Bf. To me this looks like the Force at these points would mediate a rotation about an axis drawn through a charged particle in the direction of 'y' if moving through a static Bf as shown, is this correct?
Last edited by a moderator: