greypilgrim
- 579
- 44
Hi.
I had a question about railguns, but I think I can formulate the underlying problem more clearly and concisely, hence I'm opening a different thread.
Consider the following rigid arrangement of three pieces of wire and two parallel capacitor plates:
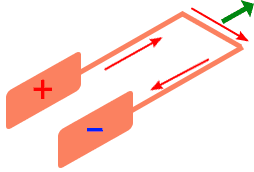 There's an open switch somewhere in the wire and the capacitor is charged using an external voltage source, which is then removed. Then the switch is closed. This will start a current to flow in the direction indicated. The parallel pieces of wire both create a magnetic field that points downwards at every point of the transverse wire (maybe only approximately, since the wire pieces are finite). This causes a Lorentz force in the direction of the green arrow.
There's an open switch somewhere in the wire and the capacitor is charged using an external voltage source, which is then removed. Then the switch is closed. This will start a current to flow in the direction indicated. The parallel pieces of wire both create a magnetic field that points downwards at every point of the transverse wire (maybe only approximately, since the wire pieces are finite). This causes a Lorentz force in the direction of the green arrow.
If I'm not missing anything, all other forces cancel due to the rigidity of the arrangement. This seems to violate conservation of momentum. I can see two ways out here:
I had a question about railguns, but I think I can formulate the underlying problem more clearly and concisely, hence I'm opening a different thread.
Consider the following rigid arrangement of three pieces of wire and two parallel capacitor plates:
If I'm not missing anything, all other forces cancel due to the rigidity of the arrangement. This seems to violate conservation of momentum. I can see two ways out here:
- The loop really start to move and there's opposite momentum carried away by the EM-field.
- This arrangement reminds me suspiciously of the thought experiments that lead to displacement current. Since the electric field between the plates decreases, there is indeed displacement current there and it's directed opposite the current in the transverse wire.
Now, does Lorentz force also act on displacement currents? I have never heard of that, but that could potentially compensate the green force.
Last edited: