rudransh verma
Gold Member
- 1,067
- 96
- Homework Statement
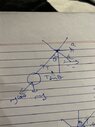
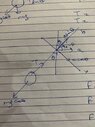
- A small sphere is suspended by a string from the ceiling of car. If the car begins to accelerate with a , the tension generated in string is(##T_0## is tension at rest)
- Relevant Equations
- F=ma
If I draw the fbd then some force will accelerate the car in horizontal direction which I think does not effect the string in vertical direction. So same tension regardless of acceleration.
But we know it will increase. So what will be the correct physics behind it?
But we know it will increase. So what will be the correct physics behind it?