- #1
allisrelative
- 26
- 3
The answer is no and even when decoherence occurs for Wigner's Friend in the lab, quantum coherence remains. Let's start with the paper that illustrates this.
Assisted Macroscopic Quantumness
Wow, I recently read this paper and the results are simply fascinating. Many people think decoherence means coherence is lost to the environment. This shows this isn't the case. Coherence remains even in the face of decoherence. So Wigner's Friend, in this scenario Alice, can be in a decohered state in the lab and Wigner outside of the lab can still measure coherence which supports my recent posts that said you can't confuse decoherence with collapse of the wavefunction. Here's more from the paper.
So Wigner's Friend Alice in the lab is in a decohered state but Wigner outside of the lab can still measure coherence and he can only measure coherence if macroscopic Alice is included as an informationally indispensable part of the quantum system. Without Alice, there's no entanglement measured in a and t.
So even when the system has decohered and coherence appears to be lost, the information about coherence can still be recovered on if macroscopic Alice is included as part of the quantum system. Here's more:
So while decoherence has occurred it doesn't remove coherence from an information-theoretic perspective. So in this case, decoherence is only relative to Wigner's Friend in the lab. Here's more:
So decoherence appears to destroy the entanglement in partition (A|at) but this is only relative to A(Alice) in the lab. This isn't enough to achieve macroscopic classicality because the larger system measured by Wigner outside of the lab includes aA which includes Alice and qubit a remains entangled to qubit t even though a and t aren't entangled.
This goes to what Rovelli says in the Relational interpretation. Wigner O' has information about (aA|t) which is a larger system than (A|at) which appears to Alice as a decohered state. It's not though and you need A to describe (aA|t) as an entangled system.
So decoherence doesn't remove quantumness. So Wigner's Friend in the lab can be in a state of decoherence and appear to see a classical outcome in the lab, Wigner outside of the lab can still measure coherence. This result has practical implications for quantum key distribution.
Assisted Macroscopic Quantumness
CONT.It is commonly expected that quantum theory is universal, in that it describes the world at all scales. Yet, quantum effects at the macroscopic scale continue to elude our experimental observation. This fact is commonly attributed to decoherence processes affecting systems of sufficiently large number of constituent subsystems leading to an effective macro-scale beyond which a quantum description of the whole system becomes superfluous. Here, we show both theoretically and experimentally that the existence of such a scale is unjustifiable from an information-theoretic perspective. We introduce a variant of the Wigner's friend experiment in which a multiparticle quantum system is observed by the friend. The friend undergoes rapid decoherence through her interactions with the environment. In the usual version of this thought experiment, decoherence removes the need for Wigner to treat her as a quantum system.
https://arxiv.org/abs/1711.10498However, for our variant we prove theoretically and observe experimentally that there exist partitions of the subsystems in which the friend is entangled with one of the particles in assistance with the other particle, as observed by Wigner. Importantly, we show that the friend is indispensable for the entanglement to be observed. Hence Wigner is compelled to treat the friend as part of a larger quantum system. By analyzing our scenario in the context of a quantum key distribution protocol, we show that a semi-classical description of the experiment is suboptimal for security analysis, highlighting the significance of the quantum description of the friend.
Wow, I recently read this paper and the results are simply fascinating. Many people think decoherence means coherence is lost to the environment. This shows this isn't the case. Coherence remains even in the face of decoherence. So Wigner's Friend, in this scenario Alice, can be in a decohered state in the lab and Wigner outside of the lab can still measure coherence which supports my recent posts that said you can't confuse decoherence with collapse of the wavefunction. Here's more from the paper.
A commonly conjectured roadblock for macroscopic quantum effects is quantum decoherence related to the size of the considered system [12, 13]. Loosely speaking, according to some (rather intuitive) definitions of macroscopic quantumness wherein the size of the system (and thus the number of its constituent elements) is a decisive parameter [14–21], the larger the system is the harder it is to isolate it from interactions with the environment. Such interactions, in turn, destroy the coherence of the system, hence, no quantum property of a macroscopic, i.e. sufficiently large, system can be observed unless via extremely high-precision measurements [14, 17]. In other words, it is assumed that there exists a “scale” beyond which physical systems become “macroscopic” and can be analyzed without any reference to the quantum formalism.
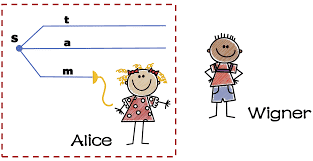
In this contribution we challenge this view by showing in theory and experiment that, with the assistance of a second system, a macroscopic system (in the sense of being made up by a very large number of subsystems) can be proven to be entangled even after complete decoherence. We set the scene as in a Wigner’s friend experiment, with the crucial difference that the observer is not assumed to preserve quantum coherence, and analyze Wigner’s perspective, see Fig. 1. In our variant, Wigner’s friend is in possession of two particles labeled a and t. We find that, even in the presence of decoherence and regardless of its dynamics, the joint subsystem of Wigner’s friend and particle t exhibits entanglement with particle a, while there is no entanglement in any pair of subsystems alone. Consequently, as far as the information content of Wigner’s friend is concerned, she constitutes a quantum system in assistance with particle t regardless of her size. More specifically, Wigner has to use the quantum formalism to describe the entanglement in this particular partition, independent of our choice of interpretation of quantum theory. Hence, there seems to be no escape from the conclusion that Wigner’s friend, despite being a macroscopic observer by any sensible definition, is an informationally indispensable part of the quantum system.
So Wigner's Friend Alice in the lab is in a decohered state but Wigner outside of the lab can still measure coherence and he can only measure coherence if macroscopic Alice is included as an informationally indispensable part of the quantum system. Without Alice, there's no entanglement measured in a and t.
So even when the system has decohered and coherence appears to be lost, the information about coherence can still be recovered on if macroscopic Alice is included as part of the quantum system. Here's more:
We now briefly discuss the crucial differences in the analysis and interpretation of our scenario compared to the conventional Wigner’s friend experiment. There has recently been an increasing interest in the modified Wigner’s friend scenarios, both in theory [26, 27] and experiment [28, 35]. These works are primarily devoted to the disconnect between Wigners perception of reality and that of the friend. In contrast, our aim is to investigate the extent to which the assumption of the existence of a macro-scale beyond which a quantum description becomes redundant is valid.
Here, we have extended the macroscopic system “Alice” of the conventional proposals to an even larger macroscopic system Aa (that is, Alice plus the qubit a). This effectively debilitates the decoherence mechanism’s ability to reach “classicality”, since it only leads to a loss of coherence between Alice and the microscopic subsystems.
So while decoherence has occurred it doesn't remove coherence from an information-theoretic perspective. So in this case, decoherence is only relative to Wigner's Friend in the lab. Here's more:
Technically, while decoherence destroys the entanglement in the partition (A|at), this is insufficient to achieve macroscopic “classicality”, since the macroscopic system aA (which is larger than Alice) remains entangled to the qubit t. At the same time, there is no entanglement in the partition (a|t), implying that the observed entanglement is not merely due to the microscopic subsystems a and t.
At this point one may be tempted to think of Alice as simply holding a piece of (classical) information, which identifies one or the other entangled quantum state of subsystem at. We emphasize that this requires one to refer to the individual subsystem A which, in turn, means working with either the bipartition (A|at) or the tripartition (A|a|t).
So decoherence appears to destroy the entanglement in partition (A|at) but this is only relative to A(Alice) in the lab. This isn't enough to achieve macroscopic classicality because the larger system measured by Wigner outside of the lab includes aA which includes Alice and qubit a remains entangled to qubit t even though a and t aren't entangled.
This goes to what Rovelli says in the Relational interpretation. Wigner O' has information about (aA|t) which is a larger system than (A|at) which appears to Alice as a decohered state. It's not though and you need A to describe (aA|t) as an entangled system.
While this view justifies some kind of classical-quantum description of the whole system across the partitions (A|at) or (A|a|t), it fails to provide such a description for the partition (aA|t), which includes the larger macroscopic system aA of interest as an entity here, because the classical theories required to describe A do not possesses the structure to accommodate entanglement. As such, the entanglement across the partition (aA|t) can only be captured when endowing the macroscopic system aA with a fully quantum description. Indeed, conditioning on Alice’s information is a way to transfer the entanglement from the partition (aA|t) to the partition (a|t). This emphasizes the crucial role Alice’s information plays for the entanglement of the whole system. Consequently, we come to the conclusion that there are micro-macro systems that show quantum features, even in the presence of decoherence.
So decoherence doesn't remove quantumness. So Wigner's Friend in the lab can be in a state of decoherence and appear to see a classical outcome in the lab, Wigner outside of the lab can still measure coherence. This result has practical implications for quantum key distribution.
Last edited: