Does Dropping the Anchor Affect the Barge's Water Displacement?

[brotherbobby]

Homework Statement

An iron anchor with mass $35.0\;\text{kg}$ and density $7860\;\text{kg/m}^3$ lies on the deck of a small barge that has vertical sides and floats in a freshwater river. The area of the bottom of the barge is $8.00\;\text{m}^2$. The anchor is thrown overboard but is suspended above the bottom of the river by a rope; the mass and volume of the rope are small enough to ignore. After the anchor is overboard and the barge has finally stopped bobbing up and down, has the barge risen or sunk down in the water? By what vertical distance?

$\mathbf{\text{Answer = }}\; \boxed{\pmb{5.57\times 10^{-4}\;\text{m}}}$

Relevant Equations

1. $\text{The law of flotation}$ : When a body of mass $m_B$ floats in a liquid, the mass (and weight) of the body is the mass (or weight) of the liquid displaced : $m_B=\Delta m_L$ (and $w_B=\Delta w_L$).
2. Volume of a body : $V_B = \dfrac{m_B}{\rho_B}$, where $\rho_B$ is the density of the body.

(I must confess before I can begin that I found this problem difficult to understand, for reasons I will make clear below. I know it appears simple.)

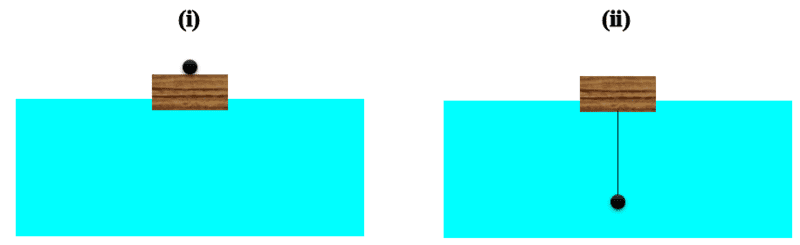Attempt : Let me begin by drawing the problem situation alongside, to the best I understand.

We can see that the in both cases (i) or (ii), the barge and anchor combination is effectively "floating" in water.

Hence, by the law of flotation (see the first of the Relevant Equations above), the mass of water displaced in both occasions : $\Delta m_W = m_B+m_A$ is the same.

If the same amount of water is displaced, the height of water "going up" as a result of the barge-anchor combination also remains the same : $\Delta h_W = \dfrac{V_W}{A}=\dfrac{m_B+m_A}{\rho_W A}$, where $A$ is the cross sectional area of the river!

The height by which the water level "went up" is the same on both occasions.

Hence, by my reasoning, the barge will neither rise nor sink further in water as a result of throwing the anchor overboard. But clearly my answer is incorrect, as per the book.

A help or hint will be welcome.

 

[willem2]

The anchor displaces water after you throw it in.

 

[haruspex]

You seem to be assuming the boat's height above the river bed is the same in both cases, thereby concluding that if the river rises the same amount against the bank it must also rise the same against the hull.

 

[brotherbobby]

The anchor displaces water after you throw it in.

True. Question is does the anchor displace the same mass of water on the two occasions?

My reason for saying "yes" as the answer to the question is that the anchor is "floating" on both occasions. It clearly displaces water equal to its weight (or mass) in case (i). But it does the same in case (ii), despite the fact that it appears that it displaces water equal to its volume when it's hanging in water.

Is my reasoning valid?

 

[kuruman]

True. Question is does the anchor displace the same mass of water on the two occasions?

If you are riding on a boat, do you displace any water? Hint: Are you wet or dry? Same with the anchor shown in the left figure.

 

[haruspex]

True. Question is does the anchor displace the same mass of water on the two occasions?

My reason for saying "yes" as the answer to the question is that the anchor is "floating" on both occasions. It clearly displaces water equal to its weight (or mass) in case (i). But it does the same in case (ii), despite the fact that it appears that it displaces water equal to its volume when it's hanging in water.

Is my reasoning valid?

Think about the force the anchor exerts on the boat in each case.

 

[brotherbobby]

If you are riding on a boat, do you displace any water? Hint: Are you wet or dry? Same with the anchor shown in the left figure.

If I am riding on a boat, the total weight of the boat is more due to my own weight. Since the mass of water displaced is the same as the (total) mass of the boat (law of flotation : $\Delta m_W = m_{\text{boat}+m_{\text{me}}}$), yes I am also displacing water.
Of course I am dry. But that doesn't mean am not displacing water.

Sorry for coming in late. Is my reasoning valid?

 

[brotherbobby]

Think about the force the anchor exerts on the boat in each case.

Yes, good question.

(1) In the first case, the anchor exerts force on the boat equal to its weight. Symbolically : $F_{\text{anchor}\rightarrow \text{boat}} = w_{\text{anchor}}$

(2) In the second case, the anchor is displacing water equal to its weight.
(Or is it equal to its volume? Am confused on this crucial point. As I have argued in my original post #1, the anchor is also "floating" in the second case. Hence, by the law of flotation, it is displacing water equal to its weight, least by my reasoning.)

If that is true, the anchor should be weightless! ($\text{Upthrust} = w_{\text{anchor}}$). Hence the rope should be slack and not taut, but it clearly is taut. Such an anchor would exert no force on the boat.

Please tell me where am mistaken.

 

[kuruman]

Hence, by the law of flotation, it is displacing water equal to its weight, least by my reasoning.)

How does the law of flotation apply in this case? If the rope were longer, the anchor would sink to the bottom. That's how anchors work. Yes, the anchor displaces water but the weight of the displaced water would be equal to the anchor's weight only if the anchor had the same average mass density as the water it displaces. Does it?

 

[haruspex]

Or is it equal to its volume?

Yes. It might help to understand the basis of Archimedes' principle. Imagine removing the immersed object and filling the void with more of the fluid it was displacing. This must be in equilibrium, so the weight of the fluid used to fill the void must equal the upthrust of the rest of the fluid on that shape.

As I have argued in my original post #1, the anchor is also "floating" in the second case.

No, you wrote that the anchor and boat combination is floating, which is true.

 

[brotherbobby]

Sorry for coming in late (work reasons), but thank you all, specially @haruspex and @kuruman for your help. I am the creator of this post (OP as you say). The problem above might look simple to you, but I am as confused today as the day I had written post#1 above. Let me summarise where am stuck.

Problem scene : In image (i) on the right, an anchor (in black) lies on a boat (in brown) as they float. In (ii), the anchor is dropped from the boat into the water and held by the boat by means of a rope. Note the anchor is suspended in water, above the bottom of the river.

Question : Does the boat rise or sink in water as a result of throwing the anchor over [in (ii)]?

Issue : Does the anchor displace water equal to its volume or equal to it weight?

Attempt : My feeling thus far is that in case (ii), the anchor is still floating in water and therefore displaces water equal to its weight. Thanks to suggestions above I can see that the anchor is displacing water equal to its volume : which makes the case the same as that of a person holding the anchor in water. The anchor would have a net weight of $w'_A = w_A-U$, where $U$ is the upthrust on the anchor by water. Hence the man has to apply a force of tension $T=w'_A$ in the rope. The rope would pull the man down, in turn, using Newton's third law with a force equal to $T$. This would be countered by the ground on which the man stands, pushing the man up by the same force and raising the man's weight to $w'_M = w_M+w'_A$.

But here the man is replaced by a boat floating in the river.

The boat will be pulled down by a force of $w'_A$, the reduced weight of the anchor. So the water will have to push the boat up by the same force [case (ii)]. In case (i), the boat is also pulled down by the weight of the anchor $w_A > w'_A$. However, in case (ii), the anchor would displace some water equal to its volume : $\Delta V_W = V_A = \frac{m_A}{\rho_A}$. Let me use the numbers given for the problem (see post #1). The anchor has a mass $m_A = 35$ kg and density $\rho_A = 7860\, \text{kg/m}^3$. Hence the volume of water displaced by the anchor in case (ii) is : $\Delta V_{W_2} = V_A = \frac{35}{7860} = 4.45\times 10^{-3}\,\text{m}^3$. The volume of water displaced by the anchor in case (i) : $\Delta V_{W_1} = \frac{m_A}{\rho_W} = \frac{35}{1000} = 0.035\,\text{m}^3$. Thus $\Delta V_{W_2} < \Delta V_{W_1}$, meaning the boat displaces less water with the anchor thrown overboard, hence the boat is expected to rise. Given the surface area of the boat : $A_B= 8\,\text{m}^2$. Hence the rise of the boat in water : $\Delta h = \frac{\Delta V_{W_1}-\Delta V_{W_2}}{A_B} = \frac{0.035-0.00445}{8} = \boxed{\pmb{3.82\times 10^{-3}\,\text{m}}}$.

I believe I am on the right track, but am yet to get the answer as is given in the text : $\mathbf{\text{Answer = }}\; \boxed{\pmb{5.57\times 10^{-4}\;\text{m}}}$.

Thank you for your interest.

 

[kuruman]

My feeling thus far is that in case (ii), the anchor is still floating in water and therefore displaces water equal to its weight.

Are you saying that if one cuts the rope that holds the anchor, the anchor will stay suspended in water?
How about a free body diagram of the anchor showing all the forces acting on it.

 

[hmmm27]

Thanks to suggestions above I can see that the anchor is displacing water equal to its volume

Go with that.

 

[haruspex]

The boat will be pulled down by a force of $w'_A$, the reduced weight of the anchor. So the water will have to push the boat up by the same force [case (ii)]. In case (i), the boat is also pulled down by the weight of the anchor $w_A > w'_A$. However, in case (ii), the anchor would displace some water equal to its volume : $\Delta V_W = V_A = \frac{m_A}{\rho_A}$. Let me use the numbers given for the problem (see post #1). The anchor has a mass $m_A = 35$ kg and density $\rho_A = 7860\, \text{kg/m}^3$. Hence the volume of water displaced by the anchor in case (ii) is : $\Delta V_{W_2} = V_A = \frac{35}{7860} = 4.45\times 10^{-3}\,\text{m}^3$. The volume of water displaced by the anchor in case (i) : $\Delta V_{W_1} = \frac{m_A}{\rho_W} = \frac{35}{1000} = 0.035\,\text{m}^3$. Thus $\Delta V_{W_2} < \Delta V_{W_1}$, meaning the boat displaces less water with the anchor thrown overboard, hence the boat is expected to rise. Given the surface area of the boat : $A_B= 8\,\text{m}^2$. Hence the rise of the boat in water : $\Delta h = \frac{\Delta V_{W_1}-\Delta V_{W_2}}{A_B} = \frac{0.035-0.00445}{8} = \boxed{\pmb{3.82\times 10^{-3}\,\text{m}}}$.

I believe I am on the right track, but am yet to get the answer as is given in the text : $\mathbf{\text{Answer = }}\; \boxed{\pmb{5.57\times 10^{-4}\;\text{m}}}$.

Thank you for your interest.

In (ii), you have confused the volume the boat no longer displaces in supporting the anchor with the volume it still displaces in supporting the anchor.

 

[brotherbobby]

Sorry for coming in late (work reasons), but thank you all, specially @haruspex and @kuruman for your help. I am the creator of this post (OP as you say). The problem above might look simple to you, but I am as confused today as the day I had written post#1 above. Let me summarise where am stuck.

View attachment 290674Problem scene : In image (i) on the right, an anchor (in black) lies on a boat (in brown) as they float. In (ii), the anchor is dropped from the boat into the water and held by the boat by means of a rope. Note the anchor is suspended in water, above the bottom of the river.

Question : Does the boat rise or sink in water as a result of throwing the anchor over [in (ii)]?

Issue : Does the anchor displace water equal to its volume or equal to it weight?

Attempt : My feeling this far is that in case (ii), the anchor is still floating in water and therefore displaces water equal to its weight. Thanks to suggestions above I can see that the anchor is displacing water equal to its volume : which makes the case the same as that of a person holding the anchor in water. The anchor would have a net weight of $w'_A = w_A-U$, where $U$ is the upthrust on the anchor by water. Hence the man has to apply a force of tension $T=w'_A$ in the rope. The rope would pull the man down, in turn, using Newton's third law with a force equal to $T$. This would be countered by the ground on which the man stands, pushing the man up by the same force and raising the man's weight to $w'_M = w_M+w'_A$.

But here the man is replaced by a boat floating in the river.

The boat will be pulled down by a force of $w'_A$, the reduced weight of the anchor. So the water will have to push the boat up by the same force [case (ii)]. In case (i), the boat is also pulled down by the weight of the anchor $w_A > w'_A$. However, in case (ii), the anchor would displace some water equal to its volume : $\Delta V_W = V_A = \frac{w_A}{\rho_A g}$. Let me use the numbers given for the problem (see post #1).

Are you saying that if one cuts the rope that holds the anchor, the anchor will stay suspended in water?
How about a free body diagram of the anchor showing all the forces acting on it.

No, of course am not. Thank you for the clarification. Clearly, the anchor has a density greater than water, so if not suspended by the boat as a "help", the anchor would drop to the river or lake bed.
However, it is precisely being held on to as a suspension by the boat that gave me reasons to think that the anchor is also floating in water like the boat.

 

[kuruman]

In one picture the anchor is on the boat, in the second picture the anchor is suspended underwater by a rope attached to the boat. Neglecting the mass and volume of the rope, is the force exerted by the anchor on the boat the same or different and why? Answer this question, and you will understand the role of the anchor in all this.

 

[haruspex]

Sorry for coming in late (work reasons), but thank you all, specially @haruspex and @kuruman for your help.

Did you understand post #14?

 

[brotherbobby]

Did you understand post #14?

Yes. The anchor is no longer on the boat in case (ii). So the boat only displaces water equal to its weight. The anchor in this case (ii) displaces water equal to its volume.
But I don't see how to include this to change my calculations in post #11 above. I subtract the volume of water displaced by the anchor in case (ii) from the same in case (i). That volume of displaced water, $\Delta V_{W_1} > \Delta V_{W_2}$. I divide this difference by the cross sectional area of the boat, $V_B$.
Nowhere have I taken the volume actually displaced by the boat.

Please advise. Thank you for your interest.

 

[brotherbobby]

In one picture the anchor is on the boat, in the second picture the anchor is suspended underwater by a rope attached to the boat. Neglecting the mass and volume of the rope, is the force exerted by the anchor on the boat the same or different and why? Answer this question, and you will understand the role of the anchor in all this.

Yes, crucial question. Thank you for asking.

(1) In case 1, the anchor exerts a force on the boat equal to its weight : $F_{{(A \rightarrow B)}_1} = w_A$.
(2) In case 2, the anchor exerts a force on the boat equal to its reduced weight : $F_{{(A \rightarrow B)}_2} = w'_A = w_A - U$, where $U$ is the upthrust of the water on the anchor.

Hence, $F_{{(A \rightarrow B)}_2} < F_{{(A \rightarrow B)}_1}$, difference being equal to the upthrust on the anchor : $U=\rho_W V_A g$. This reduced force on the boat makes the boat displace less water and helps it rise.

Thank you for the question.

 

[kuruman]

Good. In other words, when the anchor is on the boat, the boat displaces enough water to support the weight of the boat and the full weight of the anchor. When the anchor is suspended under the boat, the boat displaces enough water to support the weight of the boat and the reduced weight of the anchor. Can you translate that into a change in displaced volume and hence a change in height?

 

[haruspex]

Yes. The anchor is no longer on the boat in case (ii). So the boat only displaces water equal to its weight. The anchor in this case (ii) displaces water equal to its volume.
But I don't see how to include this to change my calculations in post #11 above. I subtract the volume of water displaced by the anchor in case (ii) from the same in case (i). That volume of displaced water, $\Delta V_{W_1} > \Delta V_{W_2}$. I divide this difference by the cross sectional area of the boat, $V_B$.
Nowhere have I taken the volume actually displaced by the boat.

Please advise. Thank you for your interest.

In post #11 you correctly calculated that the wet anchor displaces $4.45E-3m^3$ of water. That reduces the volume of water the boat needs to displace by that amount. Dividing by the boat's area gives the answer.
But, instead, you first subtracted the $4.45E-3m^3$ from the volume of water displacement needed to make the anchor float (i.e., the volume of water the boat was displacing in (i) on behalf of the anchor). So what you calculated was how much further the boat would rise if the anchor were let go completely.