PainterGuy
- 938
- 73
Hi,
I'd request you to keep it simple so it's accessible by a layman.
Question 1:
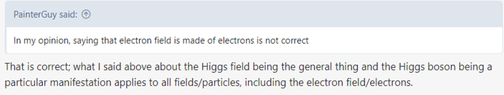
Is Higgs field made up of Higgs bosons? Higgs field has a positive value everywhere. Other fields such as electron field, hover around zero though virtual particles come into existence and decay almost instantly. Only when enough energy is added to the electron field, a real electron comes into being. In my opinion, saying that electron field is made of electrons is not correct; electron field can give rise to electrons when it is excited with enough energy. So, is Higgs field really made up of Higgs bosons?
I also read that Higgs field is a scalar field and the way to detect it was to add enough energy to create a Higgs boson which in turn verifies the existence of Higgs field since every field has an associated particle with it.
Question 2:
Also many a time it is said that Higgs field is responsible for the mass of particles. In my opinion a little correct pop-sci version would be that Higgs field is only responsible for giving mass to the elementary particles such as as electrons and quarks, and their mass slowed them down to form protons, neutrons and finally atoms. On the other hand, protons, neutrons, and atoms get only a fraction of their mass from their intrinsic mass which is a result of Higgs field, and a bigger chunk of their mass results from trapped energy. For example, each proton is made up of three quarks which possesses lots of potential energy and kinetic energy, and energy is also mass.
Thanks for the help and your time, in advance!
Helpful links:
1: /watch?v=kixAljyfdqU (add www.youtube.com in front)
2: /watch?v=Ztc6QPNUqls (add www.youtube.com in front)
3: /watch?v=2kUFs6_DBrM (add www.youtube.com in front)
4: https://www.fnal.gov/pub/science/inquiring/questions/higgs_boson.html
I'd request you to keep it simple so it's accessible by a layman.
Question 1:
Is Higgs field made up of Higgs bosons? Higgs field has a positive value everywhere. Other fields such as electron field, hover around zero though virtual particles come into existence and decay almost instantly. Only when enough energy is added to the electron field, a real electron comes into being. In my opinion, saying that electron field is made of electrons is not correct; electron field can give rise to electrons when it is excited with enough energy. So, is Higgs field really made up of Higgs bosons?
I also read that Higgs field is a scalar field and the way to detect it was to add enough energy to create a Higgs boson which in turn verifies the existence of Higgs field since every field has an associated particle with it.
Question 2:
Also many a time it is said that Higgs field is responsible for the mass of particles. In my opinion a little correct pop-sci version would be that Higgs field is only responsible for giving mass to the elementary particles such as as electrons and quarks, and their mass slowed them down to form protons, neutrons and finally atoms. On the other hand, protons, neutrons, and atoms get only a fraction of their mass from their intrinsic mass which is a result of Higgs field, and a bigger chunk of their mass results from trapped energy. For example, each proton is made up of three quarks which possesses lots of potential energy and kinetic energy, and energy is also mass.
Thanks for the help and your time, in advance!
Helpful links:
1: /watch?v=kixAljyfdqU (add www.youtube.com in front)
2: /watch?v=Ztc6QPNUqls (add www.youtube.com in front)
3: /watch?v=2kUFs6_DBrM (add www.youtube.com in front)
4: https://www.fnal.gov/pub/science/inquiring/questions/higgs_boson.html