Doppler Analysis of Twin Experiment: Valid Argument?

[robphy]

Here's a spacetime diagram that displays physically what is going on,
as well as displays every quantity to support the calculations by @Dale and @PeterDonis.
You can count everything and form the necessary ratios,
which will agree with formulas used in various approaches to explain what is going on.

The first diagram shows regular transmissions by the inertial-twin
and how they are received by the non-inertial twin.

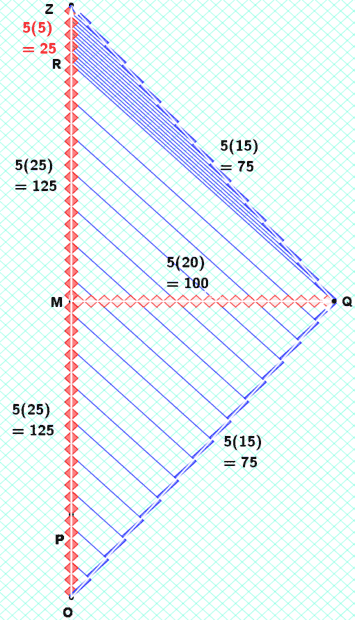
The second shows regular transmissions (with same proper period) by the non-inertial twin
and how they are received by the inertial twin.

(Each "diamond" represents 5 hours of proper time.)

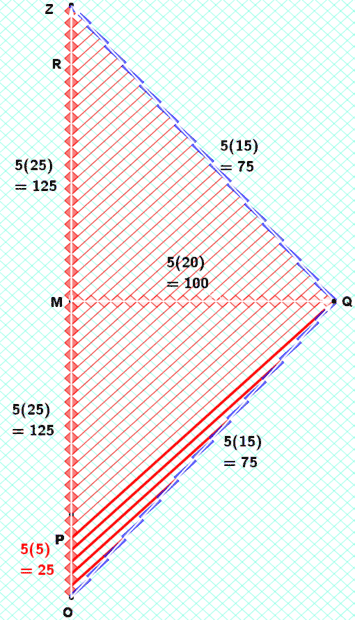
The above uses $v_{separating}=(4/5)c$ and $v_{approaching}=(-4/5)c$.

When separating ,
the ratio of reception-period to transmission-period is $\frac{OQ}{OP}=\frac{75}{25}=3$
using the transmissions by OP,
and
the ratio of reception-period to transmission-period is $\frac{OR}{OQ}=\frac{225}{75}=3$
using the transmissions by OQ. (So, these are lower-frequency receptions.)

When approaching,
the ratio of reception-period to transmission-period is $\frac{QZ}{PZ}=\frac{75}{225}=\frac{1}{3}$
using the transmissions by PZ,
and
the ratio of reception-period to transmission-period is $\frac{RZ}{QZ}=\frac{25}{75}=\frac{1}{3}$
using the transmissions by QZ. (So, these are higher-frequency receptions.)

---

The diamonds are traced out by light-signals in a light-clock.
All diamonds have edges parallel to the rotated graph paper (since the eigenvectors of the boost are along the light cones).
The blue diamonds have the same area as the red diamonds (since the determinant of the boost (the product of the eigenvalues) equals 1).
In fact, the blue diamonds are obtained from the red diamonds
by stretching along one lightlike direction by the Doppler factor $k=3$
and shrinking in the other lightlike direction by the same factor.
The timelike diagonal is along the worldline of the lightclock.
(The spacelike diagonal is simultaneous according to that clock.)

 

[Kairos]

Thanks for all your very helpful remarks! Of course the flashes are by definition emitted every hour in proper times. My above results using only apparent values were supposed to compare the perceived durations, obtained by cross-reading the watches. I completely agree that to switch to proper durations, the number of flashes for the receiver corresponds to the number of hours for the sender. I see my omission of the square root.

 

[robphy]

Possibly entertaining:
@ t=01h10m15s in Einstein’s Universe (Calder)
(the video that got me interested in relativity)

This passage discusses the clock effect with reference to temporal effects with doppler , followed by optical effects associated with doppler.