Doppler effect derivation for moving observer and stationary source

[member 731016]

Homework Statement

Please see below

Relevant Equations

Please see below

For this,

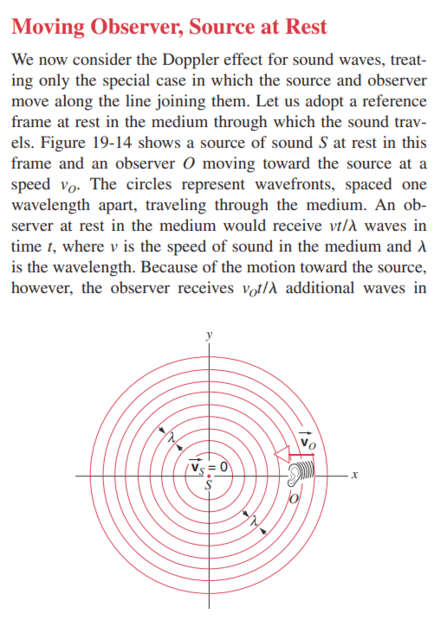
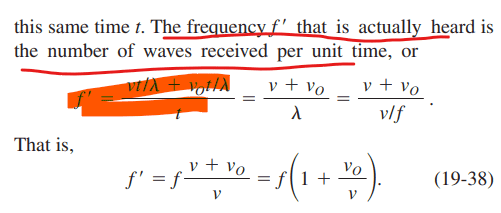
Does someone please know where they got that $f'$ is number of waves fronts received per unit time from? Also could we write the equation highlighted as $f' = \frac{n\lambda}{t}$ where $n$ is the number of wavefronts in a time $t$?

I derived that from $\frac{vt}{\lambda} = n$ and $v = f\lambda$

Many thanks!

 

[Lnewqban]

You have two velocities to consider:
- Propagation of the waves.
- Observer O.

While the observer approaches the non-moving source of sound waves, both velocities have opposite directions, producing a net velocity (which can be converted to a frequency).

While the observer moves away from the source, both velocities have the same direction, producing a net velocity (which can also be converted to another frequency).

Approaching frequency > Distancing frequency

 

[member 731016]

You have two velocities to consider:
- Propagation of the waves.
- Observer O.

While the observer approaches the non-moving source of sound waves, both velocities have opposite directions, producing a net velocity (which can be converted to a frequency).

While the observer moves away from the source, both velocities have the same direction, producing a net velocity (which can also be converted to another frequency).

Approaching frequency > Distancing frequency

Thank you for your reply @Lnewqban!

Good idea to think about it as a resultant velocity (I guess relative to the air)? The textbook says assumes that the body air is the reference frame.

Many thanks!

 

[haruspex]

Does someone please know where they got that f′ is number of waves fronts received per unit time from?

It's definitional. The frequency observed by a receiver is the rate at which whole cycles are received.

could we write the equation highlighted as $f' = \frac{n\lambda}{t}$ where $n$ is the number of wavefronts in a time $t$?

I derived that from $\frac{vt}{\lambda} = n$ and $v = f\lambda$

No, you can’t write it like that for the excellent reason that it is dimensionally inconsistent. The LHS has dimension $T^{-1}$, while the RHS has dimension $LT^{-1}$. You could not have derived it correctly from those other two equations because they are consistent.

 

[Lnewqban]

Please, see:
http://hyperphysics.phy-astr.gsu.edu/hbase/wavrel.html

http://hyperphysics.phy-astr.gsu.edu/hbase/Sound/dopp.html#c1

 

[member 731016]

It's definitional. The frequency observed by a receiver is the rate at which whole cycles are received.

No, you can’t write it like that for the excellent reason that it is dimensionally inconsistent. The LHS has dimension $T^{-1}$, while the RHS has dimension $LT^{-1}$. You could not have derived it correctly from those other two equations because they are consistent.

Thank you for your replies @haruspex and @Lnewqban !

Yeah, I can't find where they the textbook where they define frequency as number of wavelengths per unit time $f' = \frac{n}{t}$ where $n$ is the number of wave fronts in a time internal $t$. I can only find definition $f = \frac{1}{T}$ where the wave speed v is eliminated from $v = f\lambda$ and $v = \frac{\lambda}{T}$ to get the result.

However, using our definition, $f' = \frac{n}{T}$ and comparing with the equation highlighted I get:
$n = \frac{vt}{\lambda} + \frac{v_Ot}{\lambda}$.

I think $n = \frac{vt}{\lambda} + \frac{v_Ot}{\lambda}$ could be rewritten more succinctly as,

$n = \frac{n_1\lambda}{\lambda} + \frac{n_2\lambda}{\lambda}$
$n = n_1 + n_2$

Where, $n_1$ is a positive integer multiple of wavelengths to pass an stationary observer fixed to the frame of the medium and $n_2$ is a positive integer multiple of wavelengths to pass a the observer while they are moving towards the source.

I also tried rewriting it another way,

$n = \frac{d}{\lambda} + \frac{d_O}{\lambda}$

which is also dimensionally consistent and I'm still thinking about

Many thanks!

 

[haruspex]

$n = n_1 + n_2$

Where, $n_1$ is a positive integer multiple of wavelengths to pass an stationary observer fixed to the frame of the medium and $n_2$ is a positive integer multiple of wavelengths to pass a the observer while they are moving towards the source.

Yes, if you mean "… $n_2$ is the positive integer multiple of wavelengths the observer would pass while moving towards the source if the waves were stationary"

 

[member 731016]

Yes, if you mean "… $n_2$ is the positive integer multiple of wavelengths the observer would pass while moving towards the source if the waves were stationary"

Thank you for catching my mistake there @haruspex!