Doubt in a step while deriving Bertrand theorem

[Kashmir]

Goldstein 2nd ed.

In its Appendix is given the derivation of Bertrands Theorem.

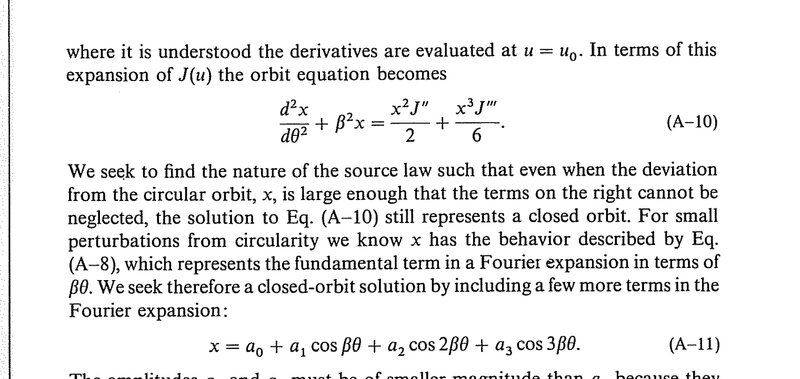Here $x=u-u_0$ is the deviation from circularity and $J(u)=-\frac{m}{l^{2}} \frac{d}{d u} V\left(\frac{1}{u}\right)=-\frac{m}{l^{2} u^{2}} f\left(\frac{1}{u}\right)$

If the R.H.S of A-10 was zero, the solution was then $a \cos(β\theta)$. However if there are terms on the RHS as given in equation A-10 the author writes the solution as a Fourier sum involving only cosine terms .
Now how does the author know that we should use a Fourier expansion of $x$ using only cosine terms with argument $β\theta$?

 

[anuttarasammyak]

Do you have a condition that RHS of A-10 is even function of $\theta$ ?

 

[Kashmir]

Do you have a condition that RHS of A-10 is even function of $\theta$ ?

We started from the orbit equation under a conservative central force written as
$\frac{d^{2} u}{d \theta^{2}}+u=J(u)$
where
$J(u)=-\frac{m}{l^{2}} \frac{d}{d u} V\left(\frac{1}{u}\right)=-\frac{m}{l^{2} u^{2}} f\left(\frac{1}{u}\right)$ which i believe if $u(x)$is its solution then $u(-x)$ is as well.
So maybe the eveness of solution comes from here. I'm not sure.