Doubt In Explanation of Proof of Chain Rule

[mopit_011]

Homework Statement

N/A

Relevant Equations

N/A

In Chapter 3 of Thomas’s Calculus, they give the following proof of the Chain Rule. After the proof, the text says that this proof doesn’t apply when the function g(x) oscillates rapidly near the origin and therefore leads delta u to be 0 even when delta x is not equal to 0. Doesn’t this proof not apply to any function that isn’t one-to-one them as delta u will be 0 when delta x is not equal to 0 in a one-to-one function?

 

[anuttarasammyak]

Could you give us an example of rapidly oscillating function g(x) you said ?

 

[mopit_011]

An example could be a function such as y = sin (1/x).

 

[anuttarasammyak]

Let g(x)= sin 1/x then
$$\delta u= \sin \frac{1}{x+\delta x}-\sin \frac{1}{x}$$
Then show me your choice of f(u) also, please.

 

[mopit_011]

We could let f(u) be a function like u^2.

 

[FactChecker]

An example could be a function such as y = sin (1/x).

This function is poorly behaved near 0 and undefined at 0. It does not have a derivative at 0 and the chain rule will not apply. The chain rule only applies when the functions have derivatives at the points of interest. (see https://en.wikipedia.org/wiki/Chain_rule#Statement)
It is possible to make examples where there is a sequence of points clustering toward the point of interest having $\Delta u=0$ and still having a derivative at the point of interest.

 

[andrewkirk]

Homework Statement:: N/A
Relevant Equations:: N/A

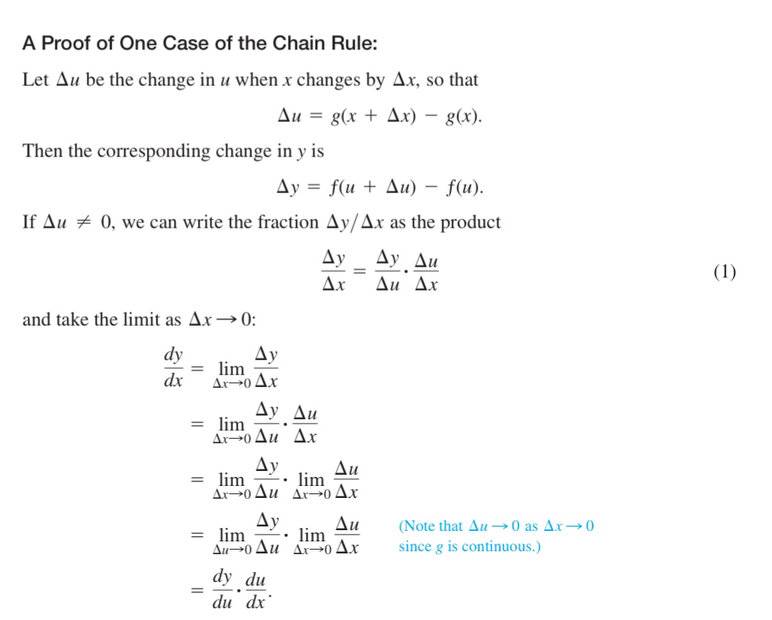
Doesn’t this proof not apply to any function that isn’t one-to-one them as delta u will be 0 when delta x is not equal to 0 in a one-to-one function?

To address the actual question asked, as shown above:
The theorem can apply to most non-one-to-one functions because, provided the function $g$ is differentiable at x and has nonzero derivative there, we can always find an open interval around x in which $g$ is one-to-one. Since we are only aiming to find the derivative of the composed function at x, we can apply the theorem within that open interval to get the result.
For a function that is not differentiable at x, such as g(z) = sin 1/(z - x), we may not be able to find such an open interval where it is one-to-one.
So the key property is being differentiable. Being one-to-one doesn't matter. Accordingly, it's also unnecessary to have a nonzero derivative, as the composed function, if differentiable, will have zero derivative at $x$ if $g$ does,
By the way, $g(z) = z^3 \sin \frac1z$ is a function that is not one-to-one on any interval including 0, but is differentiable at 0, with derivative 0.

 

[FactChecker]

The OP does not say what specific case the proof is for. Suppose that a function has a sequence of points with equal values that cluster toward the point of interest. If it has a derivative at the point of interest, then the derivative must be zero. In that case, the Chain Rule is true in a practically trivial way. That situation might not be included in the case shown.

 

[anuttarasammyak]

Ref #2-#4
$$\triangle u = \sin \frac{1}{x+\triangle x}-\sin \frac{1}{x}$$
$$=\sin [\frac{1}{x} (1- \triangle x / x )]-\sin \frac{1}{x} +o(\triangle x^2)$$
$$=\sin \frac{1}{x} \cos \triangle x / x^2 - \cos \frac{1}{x} sin \triangle x / x^2 -\sin \frac{1}{x} +o(\triangle x^2)$$
$$=( - \frac{1}{x^2}\cos \frac{1}{x})\ \triangle x +o(\triangle x^2)$$
and
$$\triangle y = 2u\triangle u + (\triangle u)^2$$
It seems compatible to the proof.

[EDIT]
y=sin 1/x is a good example of applying chain rule, i.e.
$$\frac{dy}{dx}=\frac{dy}{d(1/x)} \frac{d (1/x)}{dx} = cos(1/x) (- x^{-2})$$
We have got the same result as above easily.

 

[FactChecker]

The part of the proof shown stipulates that $\Delta u \ne 0$. So the case where $g$ is not one-to-one must be handled some other place.