Draw Angles & Find Values in Unit Circle

[mathmari]

Hey! :giggle:

Make a drawing for each of the values of the angle below indicating the angle at the unit circle (in other words: $\text{exp} (i \phi )$) and its sine, show cosine, tangent and cotangent.

Give these four values explicitly in every case (you are allowed to use elementary geometry and the Pythagorean theorem).

$$\phi=\frac{\pi}{6}, \ \ \phi=\frac{\pi}{4}, \ \ \phi=\frac{2\pi}{3}, \ \ \phi=\frac{5\pi}{6}, \ \ \phi=-\frac{2\pi}{3}, \ \ \phi=-\frac{\pi}{3}$$

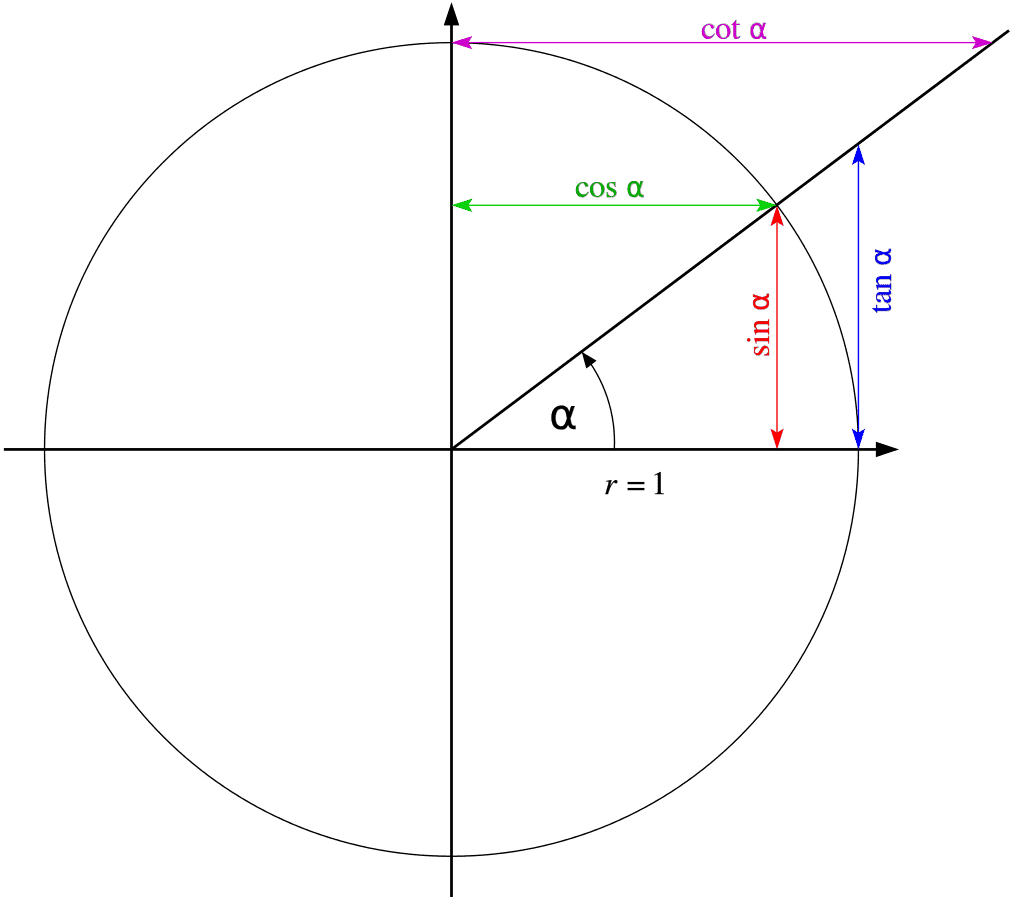
So at a unit circle we draw an angle $\phi$ and then we get a drawing like the following, right? But what does it mean to give these four values explicitly? :unsure:

 

[I like Serena]

So at a unit circle we draw an angle $\phi$ and then we get a drawing like the following, right? But what does it mean to give these four values explicitly? :unsure:

Hey mathmari!

Nice picture! (Sun)
I believe we're supposed to make a separate drawing for each of the angles, and give the corresponding values, such as $\sin\frac\pi 6=\frac 12$.

 

[mathmari]

I believe we're supposed to make a separate drawing for each of the angles, and give the corresponding values, such as $\sin\frac\pi 6=\frac 12$.

I have found an online interactive tool to show all the trigonometric functions : https://www.matheretter.de/rechner/trigonometrie

But this shows only one function at a time, do you maybe know if these is a similar tool that shows all trigonometric function in one picture?

:unsure:

 

[I like Serena]

I have found an online interactive tool to show all the trigonometric functions : https://www.matheretter.de/rechner/trigonometrie

But this shows only one function at a time, do you maybe know if these is a similar tool that shows all trigonometric function in one picture?

Have you considered Desmos, Geogebra, or TikZ?

 

[mathmari]

Have you considered Desmos, Geogebra, or TikZ?

I tried now Desmos :

:unsure:

 

[I like Serena]

I found this TikZ example on stack exchange:
https://tex.stackexchange.com/quest...-and-tangent-to-calculate-coordinates-in-tikz
\begin{tikzpicture}
\usetikzlibrary{angles,quotes}
\tikzset{My Grid/.style={help lines,color=blue!50}}

\draw[My Grid] (-4,-4) grid (4,4);
\draw (-5,0) node[ left ] {$(-5,0)$} -- (5,0) node[ right ] {$(5,0)$};
\draw (0,-5) node[ below ] {$(0,-5)$} -- (0,5) node[ above ] {$(0,5)$};
\draw (0,0) circle [ radius=3cm ];

\coordinate(O)at(0,0);
\draw[red, very thick] (30:3cm)coordinate(A)
--({3*cos(30)},0)coordinate(B);

\draw [very thick,orange] (3,0) -- (3,{3*tan(30)})coordinate(C);
\pic[fill=green!50!black,
angle radius=0.75cm,
angle eccentricity=1.2,
"\(\alpha\)"] {angle=B--O--A};
\draw (O)--(C);
\end{tikzpicture}

\begin{tikzpicture}
\usetikzlibrary{angles,quotes}
\tikzset{My Grid/.style={help lines,color=blue!50}}

  \draw[My Grid] (-4,-4) grid (4,4);
  \draw (-5,0) node[ left ] {$(-5,0)$} -- (5,0) node[ right ] {$(5,0)$};
  \draw (0,-5) node[ below ] {$(0,-5)$} -- (0,5) node[ above ] {$(0,5)$};
  \draw (0,0)  circle [ radius=3cm ];

  \coordinate(O)at(0,0);
  \draw[red, very thick] (30:3cm)coordinate(A)
                         --({3*cos(30)},0)coordinate(B);

  \draw [very thick,orange] (3,0) -- (3,{3*tan(30)})coordinate(C);
  \pic[fill=green!50!black,
       angle radius=0.75cm,
       angle eccentricity=1.2,
       "\(\alpha\)"] {angle=B--O--A};
   \draw (O)--(C);
\end{tikzpicture}

We can edit it with the TikZ Live Editor:
https://tikzimages.mathhelpboards.com/tikz/tikzlive.html