Dropping a mass on a slope, entering a loop and stopping.

[Jairo Rodriguez]

Hello, today our teacher told us that on tomorrow's test there is going to be a problem where you drop a mass on a slope which connects into a loop. The point of the problem is to calculate exactly how tall the slope must be for the mass to complete exactly one course through the loop.

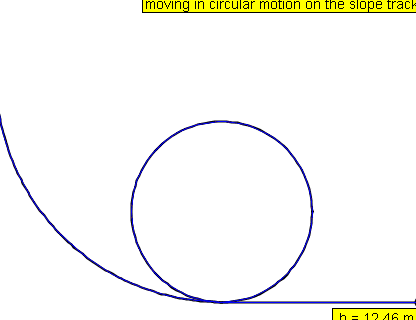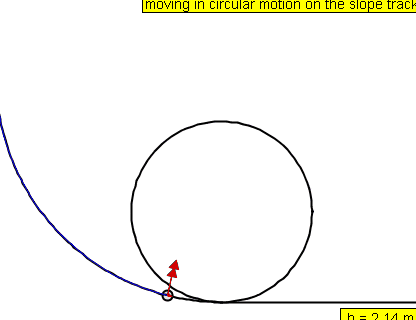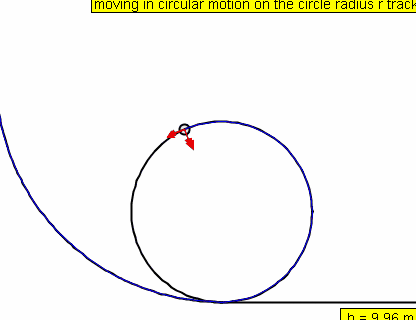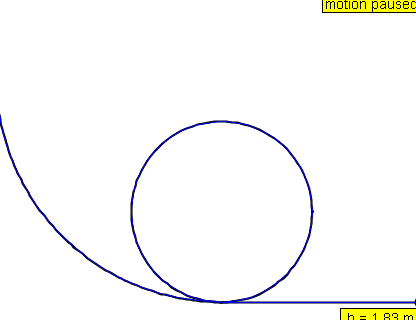
(https://upload.wikimedia.org/wikipedia/commons/1/10/CoasterH%3D2.5r.gif, I think it looks better from there.)
I hope this helps to visualize. The idea is that I drop the object on h height, and then it travels down the slope which is frictionless, then enters the loop which has friction, μ_k=0,2. I have to express algebraically the height required for the mass to stop after it has completed exactly 1 turn. The turn starts when the mass "starts going up" the loop.

I have tried doing this, I even asked another teacher (an assistant) how to do it, and he was unable to. I initially started with the usual stuff, U₁ = K₂, U₁ at the start of the slope, K₂ when the loop starts. Then I started calculating the K₃ + U₃ on the topmost part of the loop, but then I realized I have to take into account centripetal forces, also the normal force which it's different for every point on the loop, so I think I have to dive into calculus. I haven't done any calculus in my life, but if that's the only way to the answer, I am willing to do the necessary reading to at least understand the explanation.

That's all the info we get. If the post is badly redacted, I am sorry, spanish is my first language. If I wasn't clear on some point, let me know, I will try to fix it or explain myself as soon as possible. First time on this forum. Sorry again.
Thanks.

 

[Qwertywerty]

I have tried doing this, I even asked another teacher (an assistant) how to do it, and he was unable to. I initially started with the usual stuff, U1 = K2, U1 at the start of the slope, K2 when the loop starts.

First of, use the work - energy theorem, not ΔU = ΔK - this is only defined for conservative forces.
Second, see which forces act on the body. Next, which of them do work. You also want to make the normal at the top just zero.No calculus will be involved in your calculations.

Hope this helps.