MarkFL
Gold Member
MHB
- 13,284
- 12
Here are the questions:
I have posted a link there to this topic so the OP can see my work.
Calculus Review help?
I have a test coming up and these were a few problems I'm having trouble with covering related rates. Help on these problems would be incredibly appreciated.
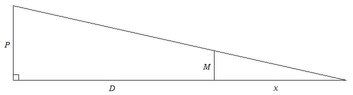
1. A street light is at the top of a 10.000 ft. tall pole. A man 5.000 ft tall walks away from the pole with a speed of 5.000 feet/sec along a straight path. How fast is the tip of his shadow moving when he is 31.000 feet from the pole?
2.Water is leaking out of an inverted conical tank at a rate of 8800.000 cubic centimeters per min at the same time that water is being pumped into the tank at a constant rate. The tank has height 7.000 meters and the diameter at the top is 4.000 meters. If the water level is rising at a rate of 29.000 centimeters per minute when the height of the water is 1.500 meters, find the rate at which water is being pumped into the tank in cubic centimeters per minute.
3. Gravel is being dumped from a conveyor belt at a rate of 50 cubic feet per minute. It forms a pile in the shape of a right circular cone whose base diameter and height are always equal to each other. How fast is the height of the pile increasing when the pile is 13 feet high? Recall that the volume of a right circular cone with height h and radius of the base r is given by V = [ 1/3]πr2h.
(I got the answer of 0.09417452253 but was incorrect)
4. A plane that is flying horizontally at an altitude of 6 kilometers and a speed of 570 kilometers per hour passes directly over a radar station. How fast is the distance between the plane and the radar station increasing when the distance between the two is 19 kilometers
5. A spherical snowball melts in such a way that its surface area decreases at a rate of 1.4 cm2/min. Find the rate at which its diameter is decreasing when the diameter is 9 cm.
(Note: The surface area of a sphere 4πr2.)
(I got the answer of 0.01733020491 but was incorrect)
6.Two cars start moving from the same point. One travels east at 35 miles per hour and the other travels north at 70 miles per hour. How fast is the distance between them increasing 35 minutes after they start.
7.A trough is 9 feet long and has ends that are isosceles triangles that are 1 foot high and 4 feet wide. If the trough is being filled at a rate of 10 cubic feet per minute, how fast is the height of the water increaseing when the height is 8 inches?
8.According to Boyle's Law, when a sample of gas is compressed at a constant temperature, the pressure and volumn satisfy the equation P V = C, where C is a constant. Assume that, at a certain instant, a sample has a volume of 750 cm3, is a pressure of 160 kPa, and the pressure is increasing at a rate of 18 kPa/min. At what rate is the volume decreasing at this instant?
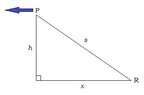
9.A lighthouse is located on a small island 2 km away from the nearest point P on a straight shoreline. Its light makes 5 revolutions per minute. How fast is the beam of light moving along the shoreline when it is 1.4 kilometers from P?
I have posted a link there to this topic so the OP can see my work.