Dynamics Question: Solving for x and N in a Rotating Disc System with Springs

[DaRotot]

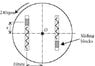
The flat disc rotates around the vertical axis through O a 240rpm (8pi rad/s). each block of 05kg starts of from the spring equilibrium poisition of x = 0.025m. Each spring has stiffness of 400 N/m. Determin the value x and the normal force N of the slot wall on the block. Neglect friction and mass of the springs.
So far I have.
$$\sum$$F(t) = 0 = Tsin$$\theta$$ - Ncos $$\theta$$
$$\sum$$F(n) = Tcos$$\theta$$ + Nsin$$\theta$$ = m.r.$$\omega^2$$
$$\sum$$F(y) = N = ma(y) = m.a.sin $$\theta$$
$$\sum$$F(n) = T = k(x -0.025)
r = $$\sqrt {0.08^2 + x^2}$$
My brain has pretty much rotted out on this occassion. Thanks

 

[KataKoniK]

for your help
Thank you for your post. I will be happy to help you with your problem.

Firstly, let's clarify the given information. From the problem, we know that the disc is rotating around the vertical axis at a speed of 240 rpm (8pi rad/s). We also know that each block has a mass of 0.5kg and starts from an equilibrium position of x=0.025m. The springs have a stiffness of 400 N/m and we are asked to determine the value of x and the normal force N of the slot wall on the block. We are also told to neglect friction and the mass of the springs.

To solve this problem, we can use Newton's laws of motion. Let's consider the forces acting on the block in the horizontal (x) direction. We have the tension force T acting on the block and the normal force N acting in the opposite direction. We can write the equation of motion as:

\sumF(x) = ma(x) = m \frac{d^2x}{dt^2} = T sin\theta - N cos\theta

Next, let's consider the forces acting on the block in the vertical (y) direction. We have the tension force T acting downwards and the normal force N acting upwards. We can write the equation of motion as:

\sumF(y) = ma(y) = m \frac{d^2y}{dt^2} = N - mg

Since the block is moving in a circular motion, we can also use the centripetal force equation to relate the tension force T to the normal force N:

T = m \frac{v^2}{r} = m r \omega^2

Substituting this into the equation of motion in the vertical direction, we get:

m \frac{d^2y}{dt^2} = N - mg = m r \omega^2

We can also use the equation for the spring force, T = k(x-0.025), and substitute it into the equation for the horizontal motion. This gives us:

m \frac{d^2x}{dt^2} = k(x-0.025) sin\theta - N cos\theta

Combining these equations, we get a system of equations that can be solved for x and N:

m \frac{d^2x}{dt^

 

[piercas]

Based on the given information, it appears that you are trying to solve for the value of x and the normal force N in a rotating disc system with springs. The equations you have written so far seem to be on the right track, but there are a few things that need to be clarified before a complete solution can be provided.

Firstly, it is important to define the coordinate system and the angles being used in the equations. In this case, it seems that the vertical axis through point O is being used as the reference axis, and the angle theta refers to the angle between the tension force T and the vertical axis. It would also be helpful to specify the direction of rotation of the disc, as this can affect the signs of the forces in the equations.

Secondly, since the disc is rotating at a constant rate of 240rpm (8pi rad/s), it is important to include the centripetal force in the equations. This force acts towards the center of rotation and is given by the equation Fc = mrω^2, where m is the mass of the block, r is the distance from the center of rotation to the block, and ω is the angular velocity. This force should be included in the \sumF(n) equation.

Thirdly, it is not clear what the variable T represents in the equations. It could refer to the tension force in the springs, but it is not specified how many springs are present in the system. It would be helpful to specify the number of springs and their locations in the system.

Once these clarifications are made, the system of equations can be solved to determine the values of x and N. It may also be helpful to draw a free body diagram to visualize the forces acting on the block and ensure that all forces are accounted for in the equations.

I hope this helps in your problem-solving process. Remember to always be clear and specific in defining your variables and coordinate system, and to double-check your equations to ensure that all forces are included. Good luck!