EC and DC circuits, using matrices

In summary, the professor wants you to find the currents through each resistor in the circuit using matrices. You can use Kickhhoff's rule to find the currents, but you don't know how to do it using matrices, so any help would be greatly appreciated.
  • #1
congo
3
0
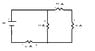
Can someone help me solve this problem using matrices and not Kirchhoff's rules. My professor posted this as a challenge question and I would like to know how to solve such a problem. Please help! I tried looking up how to solve this type of problem using matrices but to no luck. I don't know where to even begin with this question. Here's what the circuit looks like. Any help is greatly appreciated. Thank You!

I did this question using kirchhoff's rule and I would like to know how to solve this using matrices. Please guide me on how use matrices to get to the answers.
 

Attachments

  • Kichoff's circuit.jpg
    Kichoff's circuit.jpg
    10.9 KB · Views: 475
Physics news on Phys.org
  • #2
Do you mean to ask how to solve a system of equations using matrices? Post the exact challenge of your professor, or are you talking about mesh analysis more so than the math to solve the equations?
 
  • #3
Sorry for not being clear. He wants me to find the current through each resisters using matrices format. I found the currents using Kickhhoff's rule, but I do not know how to do it using matrices, any help would be greatly appreciated.
Here's the answers I found using kichhoff's rule.

I1= 0.92A
I2= 0.65A
I3= 0.27A

Thank you
 
  • #4
congo said:
Sorry for not being clear. He wants me to find the current through each resisters using matrices format. I found the currents using Kickhhoff's rule, but I do not know how to do it using matrices, any help would be greatly appreciated.
Here's the answers I found using kichhoff's rule.

I1= 0.92A
I2= 0.65A
I3= 0.27A

Thank you

Welcome to the PF.

You can use the matrix method to solve simultaneous equations. Your professor wants you to write the simultaneous equations for that circuit, and solve those simultaneous equations using the matrix method:

http://en.wikipedia.org/wiki/Simultaneous_equations

You can use either the KCL or KVL method to write the two equations, and then solve using matrices.
 
  • #5
Sorry, I don't know what's KCL or KVL. My professor never mentioned anything with KCL or KVL. Can you clarify a little about KCL and KVL please?
 
  • #6
congo said:
Sorry, I don't know what's KCL or KVL. My professor never mentioned anything with KCL or KVL. Can you clarify a little about KCL and KVL please?

Happy to. KCL = Kirchoff's Current Law (node equations). KVL =n Kirchoff's Voltage Law (Mesh loop equations).

You can use wikipedia.org for more info on those circuit analysis techniques if needed. If you have further questions, post here, but show your work and your analysis of the wikipedia pages.
 

FAQ: EC and DC circuits, using matrices

What is the difference between an EC and DC circuit?

An EC (electronic circuit) is a circuit that uses electronic components such as resistors, capacitors, and transistors to control the flow of electric current. A DC (direct current) circuit is a circuit that uses a constant, unidirectional flow of electric current. The main difference between the two is the type of current used in each circuit.

How do matrices relate to EC and DC circuits?

Matrices are used to represent and solve systems of linear equations in EC and DC circuits. Each component in a circuit can be represented as a node in a matrix, and the relationships between these components can be expressed using mathematical equations. Matrices are also used to analyze the behavior of circuits and determine various properties such as voltage, current, and power.

What are the advantages of using matrices in circuit analysis?

Using matrices in circuit analysis allows for a systematic and efficient approach to solving complex circuits. Matrices can also be used to model and analyze non-linear circuits, which cannot be solved using traditional methods. Additionally, using matrices can help identify the most important components in a circuit and optimize its performance.

Can matrices be used to analyze both AC and DC circuits?

Yes, matrices can be used to analyze both AC and DC circuits. In AC circuits, the matrices are complex and include both real and imaginary components, while in DC circuits, the matrices are typically real and only include resistances, voltages, and currents. In both cases, matrices can be used to solve and analyze the circuit.

Are there any limitations to using matrices in circuit analysis?

While matrices are a powerful tool for circuit analysis, they do have some limitations. Matrices cannot account for non-linearities, such as temperature-dependent components, in a circuit. Additionally, the size and complexity of the matrices can become very large and difficult to solve for more complex circuits. In these cases, other methods such as numerical analysis may be more suitable.

Similar threads

Find the inductance of an inductor in a circuit
Replies
18
Views
2K
Steady state circuit
Replies
7
Views
309
Relation between Mutual information and Expectation Values
Replies
2
Views
583
Gamma matrices in higher (even) dimensions
Replies
1
Views
1K
How to use Kirchhoff's voltage law on this circuit?
Replies
16
Views
1K
An electric circuit problem involving 5 current variables
Replies
28
Views
2K
Solve LC and LRC Circuits Kirchhoff's Rule Problem
Replies
3
Views
1K
How Do You Calculate Capacitance in a DC Circuit with a Fully Charged Capacitor?
Replies
3
Views
1K
Determining Inductance L in an LC Circuit
Replies
5
Views
844
Why a 20 Ohm lightbulb won't light up in a closed circuit?
Replies
7
Views
1K

Hot Threads

Recent Insights

Back
Top