FranzDiCoccio
- 350
- 43
- Homework Statement
- a partially polarized light is shone on a polarizer/analyzer system. Write the percentage polarization as a function of the maximum and minimum intensity emerging from the analyzer as the polarizer is rotated.
- Relevant Equations
- Malus law+intensity of unpolarized light emerging from a polarizer.
This is problem 62 in Cutnell & Johnson's Physics (9th edition):
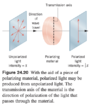
Suppose that the light falling on the polarizer in the figure is partially polarized (average intensity \bar S_P) and partially upolarized (average intensity \bar S_U). The total incident intensity is \bar S_P+ \bar S_U and the percentage polarization is 100 \bar S_P/(\bar S_P+ \bar S_U). When the polarizer is rotated in such a situation, the intensity reaching the photocell varies between a minimum value of \bar S_{\min} and a maximum value of \bar S_{\max}.
Show that the percentage polarization can be expressed as 100 (\bar S_{\max}-\bar S_{\min})/(\bar S_{\max}+\bar S_{\min}).
I might not be understanding what the problem is asking, but I'd say that the proposed solution cannot be true.
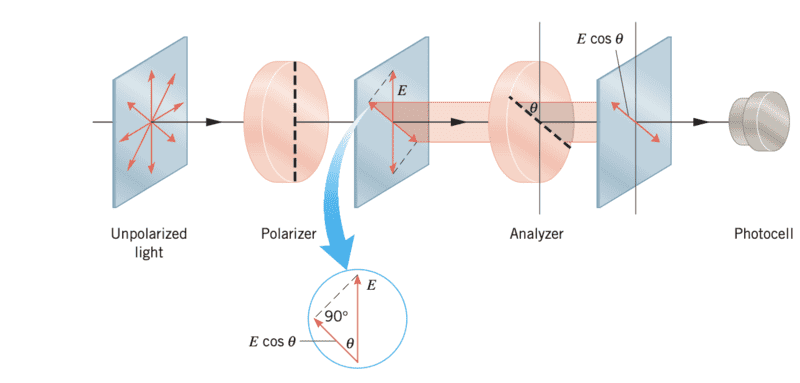
The minimum intensity reaching the photocell as the polarizer is rotated is clearly zero, isnt'it? This correspond to the polarizer axis perpendicular to the analyzer axis. Whatever goes through the polarizer emerges polarized along its axis. When it subsequently hits the perpendicular analyzer it is completely blocked.
The maximum intensity happens when the polarizer axis is parallel to the electric field in the incident polarized component. In this situation the whole polarized component is transmitted, along with half of the unpolarized component. Whatever \bar S_{\max} is, the solution proposed by the problem gives 100%, which does not make sense.
If I look at the intensity emerging from the polarizer only (and therefore not hitting the photocell yet) I get
\bar S_{\max} = \frac{1}{2} \bar S_U + \bar S_P \qquad \bar S_{\min} = \frac{1}{2} \bar S_U
In this case, I get the expected result, because \bar S_{\max}+\bar S_{\min} =\bar S_P + \bar S_U and \bar S_{\max}-\bar S_{\min} =\bar S_P.
Another possibility is that the polarizer and the analyzer are rotated so that the angle between their transmission axes remains the same.
In this case
\bar S_{\max} = (\frac{1}{2} \bar S_U + \bar S_P) \cos^2 \theta \qquad \bar S_{\min} = \frac{1}{2} \bar S_U \cos^2 \theta
and the proposed solution also applies.
Maybe this is because I'm not a native English speaker, but it seems to me that the text of the problem states that the polarizer is rotated, while it should say that both the polarizer and the analyzer are rotated by the same angle, so that the angle between their axes stays constant.
Does this make sense?
Suppose that the light falling on the polarizer in the figure is partially polarized (average intensity \bar S_P) and partially upolarized (average intensity \bar S_U). The total incident intensity is \bar S_P+ \bar S_U and the percentage polarization is 100 \bar S_P/(\bar S_P+ \bar S_U). When the polarizer is rotated in such a situation, the intensity reaching the photocell varies between a minimum value of \bar S_{\min} and a maximum value of \bar S_{\max}.
Show that the percentage polarization can be expressed as 100 (\bar S_{\max}-\bar S_{\min})/(\bar S_{\max}+\bar S_{\min}).
I might not be understanding what the problem is asking, but I'd say that the proposed solution cannot be true.
The minimum intensity reaching the photocell as the polarizer is rotated is clearly zero, isnt'it? This correspond to the polarizer axis perpendicular to the analyzer axis. Whatever goes through the polarizer emerges polarized along its axis. When it subsequently hits the perpendicular analyzer it is completely blocked.
The maximum intensity happens when the polarizer axis is parallel to the electric field in the incident polarized component. In this situation the whole polarized component is transmitted, along with half of the unpolarized component. Whatever \bar S_{\max} is, the solution proposed by the problem gives 100%, which does not make sense.
If I look at the intensity emerging from the polarizer only (and therefore not hitting the photocell yet) I get
\bar S_{\max} = \frac{1}{2} \bar S_U + \bar S_P \qquad \bar S_{\min} = \frac{1}{2} \bar S_U
In this case, I get the expected result, because \bar S_{\max}+\bar S_{\min} =\bar S_P + \bar S_U and \bar S_{\max}-\bar S_{\min} =\bar S_P.
Another possibility is that the polarizer and the analyzer are rotated so that the angle between their transmission axes remains the same.
In this case
\bar S_{\max} = (\frac{1}{2} \bar S_U + \bar S_P) \cos^2 \theta \qquad \bar S_{\min} = \frac{1}{2} \bar S_U \cos^2 \theta
and the proposed solution also applies.
Maybe this is because I'm not a native English speaker, but it seems to me that the text of the problem states that the polarizer is rotated, while it should say that both the polarizer and the analyzer are rotated by the same angle, so that the angle between their axes stays constant.
Does this make sense?