milkism
- 118
- 15
- Homework Statement
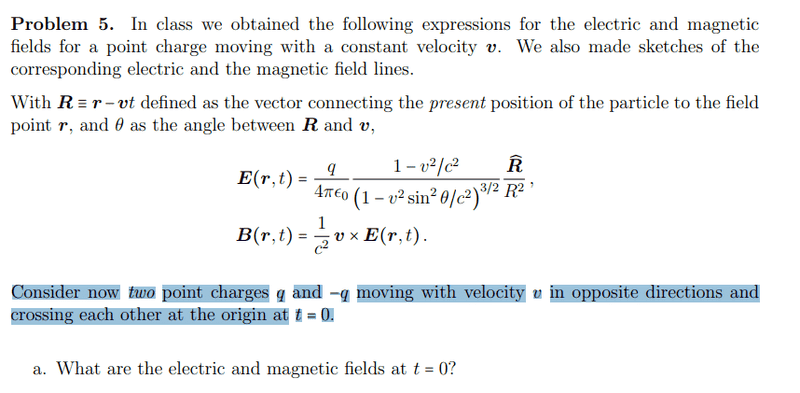
- Say two point charges q and −q moving with velocity v in opposite directions and
crossing each other at the origin at t = 0.
- Relevant Equations
- Electric and magnetic field lines of moving charges.
Part a)
My solution:

Big R basically becomes r, and the electric and magnetic field lines are doubled because of superposition principle. Am I right?
My solution:
Big R basically becomes r, and the electric and magnetic field lines are doubled because of superposition principle. Am I right?
