- #1
Saitama
- 4,243
- 93
Homework Statement
A hollow insulating sphere of radius R is charged uniformly to a charge of Q. There is a small hole on this sphere. What is the electric field strength at the centre of this hole?
Homework Equations
The Attempt at a Solution
I think I have to use Gauss's law here but what should be the Gaussian surface here? I am thinking of a small cylinder around the hole. Correct?
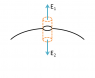
Please see the attachment.
##E_1## is the electric field just above the hole (outside) and ##E_2## is the electric field inside.
From Gauss's Law, ##E_1S-E_2S=0 \Rightarrow E_1=E_2##.
Am I going in the right direction? What should be the next step?