Electric field due to a charged infinite conducting plate

[vcsharp2003]

Homework Statement

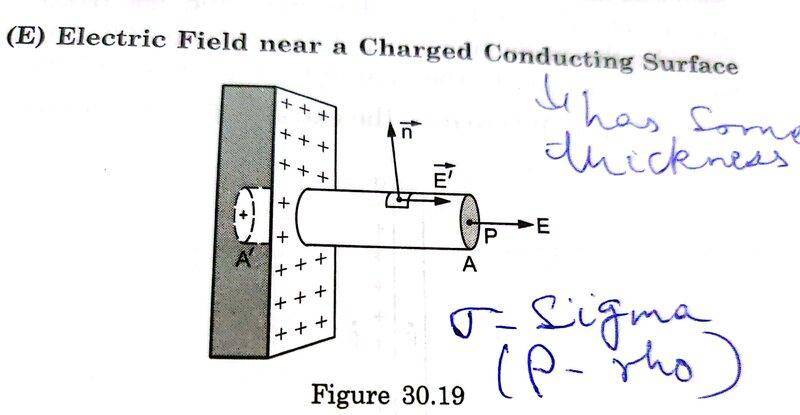
The book derives a formula for a point close to the charged conductor and goes on to say that this formula applies to any charged conductor without sharp points. This derived formula is $E = \frac {\sigma} {\epsilon_o}$ where $\sigma$ is the uniform charge density on the surface of conductor. Why is book assuming the formula is only for near points?

Relevant Equations

$E = \frac {\sigma} {\epsilon_o}$

As shown in figure below, the electric field E will be normal to the cylinder's cross sectional A
even for distant points since the charge is distributed evenly all over the charged surface and also the surface is very large resulting in a symmetry. So the derived formula should also apply to distant points.

I am not sure if my reasoning is correct.

 

[PhDeezNutz]

I agree with your reasoning.

Can you provide an exact quote/context from your book?

I don’t think your book is saying “this is only valid right outside the sheet”

I think your book is implicitly saying “here’s a general expression valid everywhere and since we are dealing with boundary conditions (or electrostatic pressure) we deal with the near field (which is the same as the far field)”

Correct me if I’m wrong.

 

[vcsharp2003]

I don’t think your book is saying “this is only valid right outside the sheet”

You're right in that the book is not explicitly saying this formula is for near points only. But, the figure given by the book for this derivation mentions its title as "Electric field near a charged conductor", so it leads one to believe that its for near points. The diagram from book is as given in my question. I think the book should have explicitly stated that the formula applies for near as well as distant points for a flat conductor.

However, it seems correct to say that this formula derived for a flat conductor would also apply to near points only ( and not distant points) for a conductor of arbitrary shape.

 

[PhDeezNutz]

However, it seems correct to say that this formula derived for a flat conductor would also apply to near points only ( and not distant points) for a conductor of arbitrary shape.

I think me and you agree. Your book should not have used an infinite conducting plane as a general example when talking exclusively about the near field.

 

[Steve4Physics]

I'd like to add to what has already been said.

$E = \frac {\sigma} {\epsilon_o}$ works for an infinite, flat, charged conducting surface. It follows directly from Gauss’s law and symmetry. It is true for both ‘near’ and ‘far’ points.

I think the book is referring to charged conducting surfaces of any shape. The above formula can still be used – but only for the near fields.

Imagine a non-flat surface with no sharp points/corners/edges. If we are close enough to the surface, the surface appears flat.

This is a result of geometry and scale. E.g. the Earth is spherical, but when we are close to the surface, the surface around us looks like a flat (Euclidean) plane (ignoring mountains/valleys/etc. of course).

Can you see why we can use $E = \frac {\sigma} {\epsilon_o}$ for the near field of any conducting surface, irrespective of the surface’s shape, but not for the far field?

(As an aside, note that $\sigma$ will vary with the curvature of the conducting surface. So in general, the magnitude of a conductor’s near field will vary over different parts of its surface, depending on the shape of the surface.)

 

[vcsharp2003]

Can you see why we can use E=σϵo for the near field of any conducting surface, irrespective of the surface’s shape, but not for the far field?

Yes, I think so. Because the electric field $\vec E$ at point P is normal to the area A. Area A is cross sectional of gaussian surface in the question diagram. This can only be true if the area A is very close to the conductor surface and parallel to the very small area on conductor surface (electric field on the conductor surface is always perpendicular to the surface).

At far points, the electric field i.e. electric lines of force will probably be not perpendicular to a small area about the far point since lines of force will most likely diverge or curve as we go further from the charged conductor.

 

[vcsharp2003]

(As an aside, note that σ will vary with the curvature of the conducting surface. So in general, the magnitude of a conductor’s near field will vary over different parts of its surface, depending on the shape of the surface.)

Will areas on conductor surface having less curvature have a lower charge density $\sigma$?

 

[vela]

However, it seems correct to say that this formula derived for a flat conductor would also apply to near points only ( and not distant points) for a conductor of arbitrary shape.

Have you ever thought about what it means for a point to be "near" or "distant" from a conductor? Is a point 1 mm above a surface near the surface? It is if the conductor is a sphere the size of the Earth, but it's not if the sphere has a diameter of 0.1 mm. Nearness depends on a relevant length scale of the situation. For a conducting sphere of radius $R$ with charge $Q$ and a point a distance $h$ from the surface, we can say
$$E = \frac{1}{4\pi \epsilon_0}\frac{Q}{(R+h)^2} = \frac{Q/4\pi R^2}{\epsilon_0} \frac{1}{[1+(h/R)]^2} = \frac{\sigma}{\epsilon_0}[1+(h/R)]^{-2}.$$ If $h/R \ll 1$, then it's a good approximation to say $E = \sigma/\epsilon_0$. So "near" means $h \ll R$, and the relevant length scale is the sphere's radius.

For a flat sheet of charge, the relevant distance is how far away the edge of the sheet is (where fringing becomes nonnegligible). In the case of an infinite plane of charge, the distance to the (non-existent) edge is infinite, so all points are in this sense near the plane, so $E = \sigma/2\epsilon_0$ holds everywhere.

 

[Steve4Physics]

Yes, I think so. Because the electric field $\vec E$ at point P is normal to the area A. Area A is cross sectional of gaussian surface in the question diagram. This can only be true if the area A is very close to the conductor surface and parallel to the very small area on conductor surface (electric field on the conductor surface is always perpendicular to the surface).

I’d add this (which is a result of the symmetry of the charge about a normal when near enough to the surface).

Close enough to the surface, the overall field is dominated by the field from the local, flat, uniformly distributed charge. This field is normal to the surface. The field from the non-local (distant) charges is negligible. As a result, close enough to the surface, the field is normal to the surface - there is no tangential component.

Will areas on conductor surface having less curvature have a lower charge density $\sigma$?

Yes. Assuming by 'less curvature' you mean 'larger radius of curvature'.

That leads to an interesting result. The surface of a conductor is an equipotential. But consider a piece of metal with a sharp point. The surface at the point has a small radius of curvature (=large curvature). As a result, the surface charge density and hence the electric field are high at the point. A spark (discharge) is most likely to start from the point.