- #1
JamMaster
- 3
- 0
Hello. I have a problem calculating the electric field from spherical charge distribution. The exercise is:
1. Homework Statement
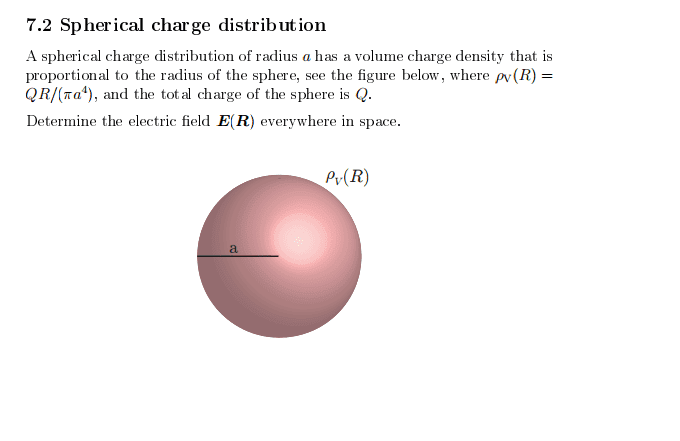
To solve the problem for $$ 0\le R < a$$ i tried 2 ways:
$$
\vec{E} = \frac{\vec{a_R}}{4\pi\epsilon_0}\int_v\frac{1}
{R^2}\rho dv
$$
and the second way
$$
V = \frac{1}{4\pi\epsilon_0}\int_v\frac{1}{R}\rho dv
$$
and
$$
\vec{E} = -\vec{\nabla}V
$$
3. The Attempt at a Solution
Unfortunately, neither of the attempts worked.
Using first method, substituting the charge density from the text and using spherical coordinates to calculate the integral i got
$$
\vec{E} = \frac{Q\vec{a_R}}{4\pi\epsilon_0a^4}\int_v\frac{1}
{R}R^2\sin{\theta}dRd\theta d\phi = \frac{QR^2}{2\pi\epsilon_0a^4}\vec{a_R}
$$
Which is wrong. The correct answer is very close though, where there is a 4 instead of 2 in the answer above. No matter how much i looked i couldn't find where i missed the constant.
Using the second method, i got
$$
V = \frac{Q}{4\pi^2\epsilon_0a^4}\int_vR^2\sin{\theta}dRd\theta d\phi = \frac{QR^3}{3\pi\epsilon_0a^4}
$$
Which gives the electric field
$$
\vec{E} = -\vec{a_R} \frac{\partial{V}}{\partial{R}} = -\frac{QR^2}{\pi\epsilon_0a^4}\vec{a_R}
$$
The above answer is even more wrong than the previous one.
I would really appreciate if someone could tell me what i have done wrong.
1. Homework Statement
Homework Equations
To solve the problem for $$ 0\le R < a$$ i tried 2 ways:
$$
\vec{E} = \frac{\vec{a_R}}{4\pi\epsilon_0}\int_v\frac{1}
{R^2}\rho dv
$$
and the second way
$$
V = \frac{1}{4\pi\epsilon_0}\int_v\frac{1}{R}\rho dv
$$
and
$$
\vec{E} = -\vec{\nabla}V
$$
3. The Attempt at a Solution
Unfortunately, neither of the attempts worked.
Using first method, substituting the charge density from the text and using spherical coordinates to calculate the integral i got
$$
\vec{E} = \frac{Q\vec{a_R}}{4\pi\epsilon_0a^4}\int_v\frac{1}
{R}R^2\sin{\theta}dRd\theta d\phi = \frac{QR^2}{2\pi\epsilon_0a^4}\vec{a_R}
$$
Which is wrong. The correct answer is very close though, where there is a 4 instead of 2 in the answer above. No matter how much i looked i couldn't find where i missed the constant.
Using the second method, i got
$$
V = \frac{Q}{4\pi^2\epsilon_0a^4}\int_vR^2\sin{\theta}dRd\theta d\phi = \frac{QR^3}{3\pi\epsilon_0a^4}
$$
Which gives the electric field
$$
\vec{E} = -\vec{a_R} \frac{\partial{V}}{\partial{R}} = -\frac{QR^2}{\pi\epsilon_0a^4}\vec{a_R}
$$
The above answer is even more wrong than the previous one.
I would really appreciate if someone could tell me what i have done wrong.
