How Does Gauss' Law Apply to Electric Fields in a Hollow Sphere?

[underground]

Hey I am currently studying for my final and I am stuck on a question i have the solution but I am not sure what he did, could someone explain

A hollow sphere of the inner radius R1 and outer radius R2 is uniformly charges with total charge Q. Calculate the electruc fiels in the three regions shown in the cross-section view below 1) r<R1, 2) R1 < r <R2, and 3) r >R2

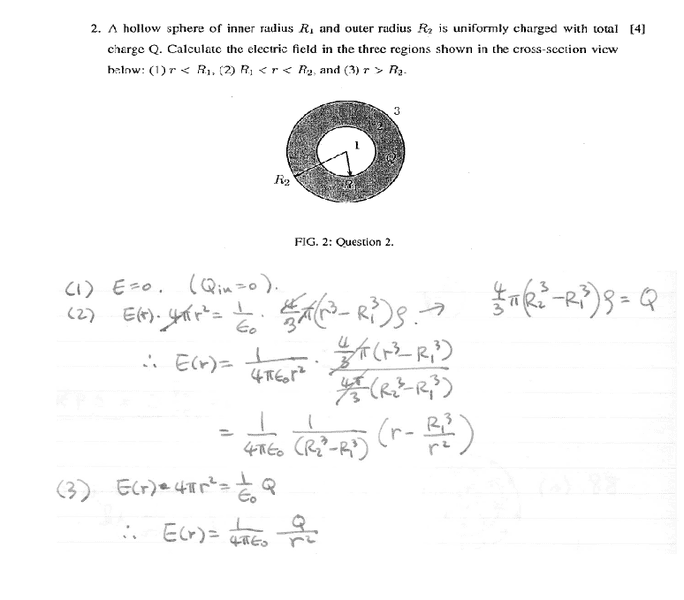
What i don't get is part two, i know he is using gauess law Q inclosed/e = E(r)*A
but i don't know how he got Q and reduced it

 

[tiny-tim]

welcome to pf!

hi underground! welcome to pf!

A hollow sphere of the inner radius R1 and outer radius R2 is uniformly charges with total charge Q.
…
What i don't get is part two, … i don't know how he got Q and reduced it

the total charge is Q, so the charge density is Q/volume = Q/{4π/3(R₂³ - R₁³)},

so the charge inside radius r is the density times the volume within radius r, ie 4π/3(r³ - R₁³)

 

[underground]

Thanks for the respond I am getting there but can you explain one thing

I get what your saying but i don't see where the Q overall charge disappeard

This is my logic of the question and the Q which is overall charge remained in the equation

 

[tiny-tim]

suppose R₁ was 0 (ie, a solid sphere) …

then the charge within radius r would be Q(r/R₂)³, wouldn't it?

this is similar … the charge is Q times (volume/total-volume) ​

 

[underground]

Thanks so much for your help :)