Electric flux through a surface

[haha1234]

Homework Statement

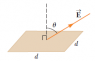
Find the electric flux through the plane surface shown in the figure below if θ = 64.2°, E = 375 N/C, and d = 4.70 cm. The electric field is uniform over the entire area of the surface.

Homework Equations

The Attempt at a Solution

By using the equation:EAcosθ
375x0.047²cos64.2=0.167
I've found that answer is 0.167N.m²/C,but it is not correct.
What is the correct answer?

 

[jtbell]

Please show us exactly how you calculated your answer.

 

[haha1234]

Please show us exactly how you calculated your answer.

I'v edited the content of my thread.Tell me of there are any things are need to be added.

 

[Saitama]

I'v edited the content of my thread.Tell me of there are any things are need to be added.

You have done the calculations incorrectly, what did you input in your calculator?

 

[haha1234]

You have done the calculations incorrectly, what did you input in your calculator?

375x0.047²cos64.2=0.167

 

[Saitama]

375x0.047²cos64.2=0.167

I don't seem to be getting 0.167 with Wolfram Alpha.

 

[haha1234]

I don't seem to be getting 0.167 with Wolfram Alpha.

But I cannot get he other answer.

 

[Tanya Sharma]

E=375
d² = .002209
Cos64.2° = .435231

Multiply the three quantities and you should get something like .3605

 

[Tanya Sharma]

But I cannot get he other answer.

because the tool is interpreting the angle 64.2 in radians .

 

[haha1234]

E=375
d² = .002209
Cos64.2° = .435231

Multiply the three quantities and you should get something like .3605

Thanks. I finally found that I have changed the mode of my calculator into radian,so I cannot find the correct answer.