Electric Potential of point outside cylinder

[jkthejetplane]

Homework Statement:

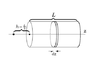
So i am supposed to set up an integral with s (radial) and z to show the electric potential at a point. Part b wants you to calculate the integral in s direction and write in terms of dV/dz

Relevant Equations:

V = 1/4piEps Integral (rho/r)dTau

Edit: Below is my work but i believe i have chosen the wrong values of the separation vector in the s direction. Any ideas as to what it should be?

 

[Delta2]

A complete statement of the problem would help us help you. What is the geometry of the charge density of the problem? Is it a thin cylinder? I guess not cause you write in your notes that there is no symmetry present. Please post a complete statement of the problem if possible.

 

[jkthejetplane]

A complete statement of the problem would help us help you. What is the geometry of the charge density of the problem? Is it a thin cylinder? I guess not cause you write in your notes that there is no symmetry present. Please post a complete statement of the problem if possible.

I edited it to put the whole original problem. thanks

 

[haruspex]

$(h-z)^2+(R-s)^2$? How are you defining s?

 

[jkthejetplane]

$(h-z)^2+(R-s)^2$? How are you defining s?

What do you mean? s is radial component in cylindrical

 

[haruspex]

What do you mean? s is radial component in cylindrical

So what is the distance from (s, Φ, z) to (0, 0, h)?

 

[jkthejetplane]

So what is the distance from (s, Φ, z) to (0, 0, h)?

I believe that's my main question above if i am understanding you correctly. I am not sure how to define the separation vector. Right not i have it as (h-z)zhat + (r-s)shat but i am unsure on the s direction component...

 

[Delta2]

I think the correct distance is $$|\vec{r}-\vec{r'}|=\sqrt{(h-z)^2+s^2}$$. If you do a simple figure it is straightforward pythagorean theorem.

 

[haruspex]

I believe that's my main question above if i am understanding you correctly. I am not sure how to define the separation vector. Right not i have it as (h-z)zhat + (r-s)shat but i am unsure on the s direction component...

By symmetry, Φ doesn't matter, so it reduces to Cartesian: (s, z) to (0, h).