Electric Potential problem, 4 equal charges, different signs, weird shape.

1 reply · 3K views · last reply
BATBLady
Messages
17
Reaction score
0

Homework Statement


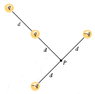
The drawing shows four point charges. The value of q is 1.96 µC, and the distance d is 0.93 m. Find the total potential at the location P. Assume that the potential of a point charge is zero at infinity.
Image of problem is attached.

Homework Equations



V=kq/r

The Attempt at a Solution



Our attempts at solving this problem included just adding the various vectors, canceling out the negative vectors since they pull in the opposite direction and just using the positive vectors, but neither works. Help? Problem due Tuesday by 11:30pm for homework. Thanks
 
Attachments
  • Diagram of four point charges along a bent line: q at top left, q at distance d from the middle charge, -q at right and -q at bottom, each separated by d and meeting at point P.
    physics poblem.gif
    2.2 KB · Views: 518

Discussion

Hi BATBLady! :wink:

Show us your full calculations, and then we can see what went wrong, and we'll know how to help! :smile:

(btw, potential is a scalar, not a vector)