- #1
NicolaiTheDane
- 100
- 10
I have been looking everywhere for a clear explanation for the following: How do I know which way to integrate the electrical field, when I'm looking for the potential between to points?
Example:
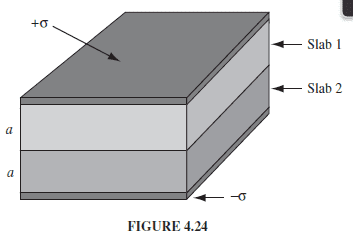
I have to find the potential between the top conducting plate and the bottom conducting plate. Assume that i got an expression for the field in both i linear dielectric slabs. My book says:
$$V = \int_\mathcal{O}^r \vec{E} \cdot d\vec{l}$$
However how do I translate that. Let's forget anything about the actually expression for ##\vec E##. Obviously we are going from ##0## til ##2*a## or the other way around. What dictates the path? I know what the answer to the assignment I'm doing is, but I do not see why my result (which is the same but negative) is wrong. It has something to do with the minus in front and the reference points. Can someone please clarify, because this has been driving me nuts for weeks now.
(The right answer comes from integrating from ##0## to ##2*a##)
Example:
I have to find the potential between the top conducting plate and the bottom conducting plate. Assume that i got an expression for the field in both i linear dielectric slabs. My book says:
$$V = \int_\mathcal{O}^r \vec{E} \cdot d\vec{l}$$
However how do I translate that. Let's forget anything about the actually expression for ##\vec E##. Obviously we are going from ##0## til ##2*a## or the other way around. What dictates the path? I know what the answer to the assignment I'm doing is, but I do not see why my result (which is the same but negative) is wrong. It has something to do with the minus in front and the reference points. Can someone please clarify, because this has been driving me nuts for weeks now.
(The right answer comes from integrating from ##0## to ##2*a##)
