Electromagnetic plane waves from a current sheet

[Luqman Saleem]

I have an infinite sheet (in lossless, homogeneous medium) of time-harmonic current in $yz$-plane at $x=−d$. The current density on this sheet is given by
$$\mathbf{J}=\hat{z}J_0\delta(x+d)$$
$δ(x+d)$ is delta function. Moreover, there is a perfect electric conductor (PEC) half space at $(0<x<∞,−∞<x,y<∞)$ as shown below

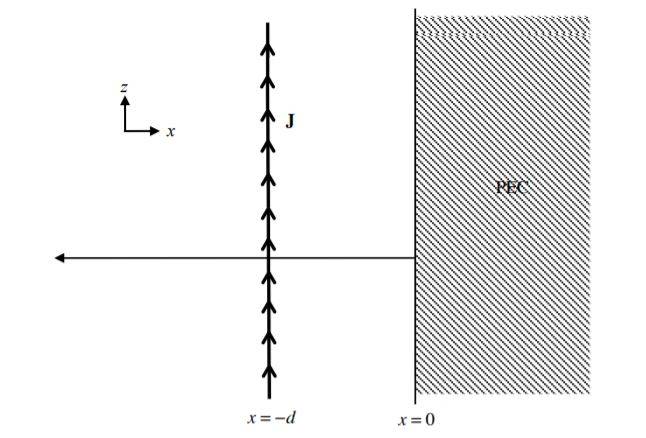
Questions are:

1. I want to find fields everywhere in the region
2. Electric current density induced on the PEC surface at $x=0$

How can I solve this problem? I can solve it if there was not PEC. Wave will be reflected from PEC, right? This reflected wave will add to the wave going toward PEC, right? Will this reflected wave also affect the wave waves in region $x<−d$?

 

[jasonRF]

Have you learned about the method of images?