Hall
- 351
- 87
- Homework Statement
- We tend to forget the electrons in almost of all of nuclear physics. We only consider them when we have to equate the masses.
- Relevant Equations
- ##n + ^{235}U \to ^{236}U^{*}##
In this thread, @haruspex presented a very deliberate point about the role of electrons in a nuclear fission reaction (he might have said or meant something else but I will present my version of it). The problem that we have before us can be stated, as candidly as my linguistic faculty of mind allows, as
How and when the electrons of the parent nucleus will be divided among the daughter nuclei in a nuclear fission?
[Well that parent, division and daughter things remind of legacy and will issue, The Dear Departed: A Comedy in One Act]
Before answering that question directly, I would like to present a related discussion.
What happens to two electrons when an isolated Radium atom decays into a Radon atom and an alpha-particle?
Radium atom was neutral, but alpha-particle is positively-charged with Radon atom being neutral, so, this seems to be going starigh against the law of conservation of charge. One of two possible ways of amending this breach of conservation law is by arguing that when alpha-particle was released from the parent nucleus the daughter nucleus left with less nucleons, i.e. lower Coulombic attraction on the orbiting electrons, and hence some outer electrons may get free (but this argument doesn't very well explain why only two electrons got free, more than two could also get free). And the second possible explanation can be: the off-shoot of the alpha-particle caused some electrons, in the electron cloud of the parent atom, to interact with it and hence moved to a higher energy state and finally got free.
But what is the end-result of these two electrons? They may get isolated (and far from each other), the chances of alpha-particle getting converted into Helium atom by taking in these two electrons are low because the speed of alpha-particle is quite high and would remain so (as we're considering an isolated system).
The second important, but auxiliary, question that we must discuss is: Why do neutrons don't collide with electrons when they are bombarded on a heavy nuclei?
Neutrons and electrons cannot be considered as classical particles, like some very small solid balls that collide and move classically, these particles are like clouds that can pass through each other (their cloud like behavior is a picture deduced from that the fact that these particles have uncertainties in their positions). So, neutrons simply passes through electrons, like a specter, and hits the nucleus.
Now, we can move to the fission of Uranium-235. When an atom of Uranium-235 is bombarded with a neutron, it absorbs the neutron snd undergoes oscillation. After some time, the shape of Uranium-236 nucleus become like this
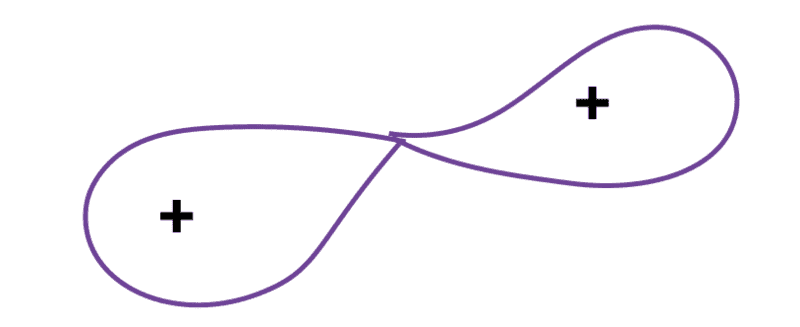
(Believe me, my fellow users, I tried my best. I know it is looking very much like many other things which it is not meant to demonstrate, but anyways meaning lies in the eye/s of beholder)
and Coulombic repulsion causes the split of nucleus. This model of fission has been taken from Meitner's and Frisch's original paper, there they have compared the unstable nucleus with the liquid drop and its subsequent deformations.
My point is, at the stage of nuclear fission which is depicted above, the electron cloud will adjust itself so as to render each nuclei neutral after separation; very similar to covalent bonds and homolytic cleavage (when atoms of same species are involved) in chemistry.
@haruspex original point was about contribution of electrical repulsion of the product nuclei in the their kinetic energies, and if presence of electrons would have some influence on this contribution or not. I would like to put forth this explanation:
At this stage:
the electron cloud is surrounding the whole deformed structure and proper distribution of electrons is yet to happen (maybe infinitesimally later), hence Coulombic repulsion would come into play and cause the separation, but once separated the nuclei would be neutral and hence Coulombic repulsion dies out, thereby having no effect on kinetic energies of the daughter nuclei. The kinetic energies of the daughter nuclei (for explanation purpose, assuming no other product than them) will add upto ##\Delta E = \Delta m c^2##.[NOTE: I shall be a little slower with my replies, for some other reason]
How and when the electrons of the parent nucleus will be divided among the daughter nuclei in a nuclear fission?
[Well that parent, division and daughter things remind of legacy and will issue, The Dear Departed: A Comedy in One Act]
Before answering that question directly, I would like to present a related discussion.
What happens to two electrons when an isolated Radium atom decays into a Radon atom and an alpha-particle?
Radium atom was neutral, but alpha-particle is positively-charged with Radon atom being neutral, so, this seems to be going starigh against the law of conservation of charge. One of two possible ways of amending this breach of conservation law is by arguing that when alpha-particle was released from the parent nucleus the daughter nucleus left with less nucleons, i.e. lower Coulombic attraction on the orbiting electrons, and hence some outer electrons may get free (but this argument doesn't very well explain why only two electrons got free, more than two could also get free). And the second possible explanation can be: the off-shoot of the alpha-particle caused some electrons, in the electron cloud of the parent atom, to interact with it and hence moved to a higher energy state and finally got free.
But what is the end-result of these two electrons? They may get isolated (and far from each other), the chances of alpha-particle getting converted into Helium atom by taking in these two electrons are low because the speed of alpha-particle is quite high and would remain so (as we're considering an isolated system).
The second important, but auxiliary, question that we must discuss is: Why do neutrons don't collide with electrons when they are bombarded on a heavy nuclei?
Neutrons and electrons cannot be considered as classical particles, like some very small solid balls that collide and move classically, these particles are like clouds that can pass through each other (their cloud like behavior is a picture deduced from that the fact that these particles have uncertainties in their positions). So, neutrons simply passes through electrons, like a specter, and hits the nucleus.
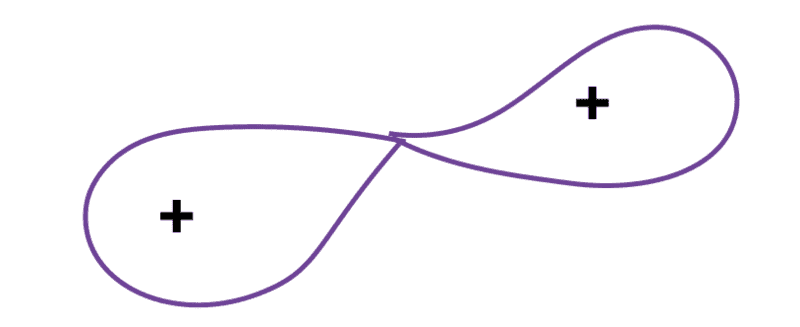
Now, we can move to the fission of Uranium-235. When an atom of Uranium-235 is bombarded with a neutron, it absorbs the neutron snd undergoes oscillation. After some time, the shape of Uranium-236 nucleus become like this
(Believe me, my fellow users, I tried my best. I know it is looking very much like many other things which it is not meant to demonstrate, but anyways meaning lies in the eye/s of beholder)
and Coulombic repulsion causes the split of nucleus. This model of fission has been taken from Meitner's and Frisch's original paper, there they have compared the unstable nucleus with the liquid drop and its subsequent deformations.
My point is, at the stage of nuclear fission which is depicted above, the electron cloud will adjust itself so as to render each nuclei neutral after separation; very similar to covalent bonds and homolytic cleavage (when atoms of same species are involved) in chemistry.
@haruspex original point was about contribution of electrical repulsion of the product nuclei in the their kinetic energies, and if presence of electrons would have some influence on this contribution or not. I would like to put forth this explanation:
At this stage:
the electron cloud is surrounding the whole deformed structure and proper distribution of electrons is yet to happen (maybe infinitesimally later), hence Coulombic repulsion would come into play and cause the separation, but once separated the nuclei would be neutral and hence Coulombic repulsion dies out, thereby having no effect on kinetic energies of the daughter nuclei. The kinetic energies of the daughter nuclei (for explanation purpose, assuming no other product than them) will add upto ##\Delta E = \Delta m c^2##.[NOTE: I shall be a little slower with my replies, for some other reason]
Last edited: