Electrostatic Force and Field between two charges

[psy]

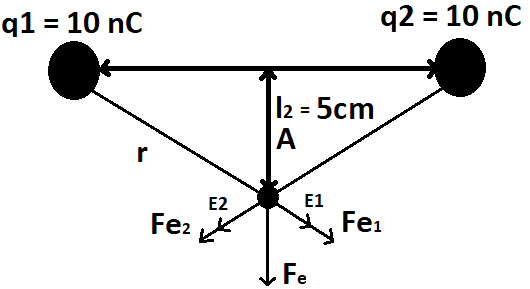
Two positive point charges with a charge of 10nC each are 10cm apart. What is the electric field strength at point A? How is it directed? Which force acts on a charge of 100nC at point A?

F = k * q1 * q2 / r^2
E = F/q
r^2 = (l1/2)^2 + (l2)^2 => r = 0,707m

Electric field strength :

E1 = E2 = 8.99 x 10^9 Nm^2/C^2 * 10*10^-9C / (0,707m)^2 = 179,854 N/C So i "pulled apart" the Electric field strength in x and y components.

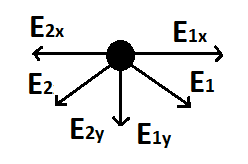
E1y = E1 * cos(45°)= 127,176 N/C
E2y = E2 * cos(45°)=127,176 N/C
E1x = E1 * sin(45°) = 127, 176 N/C
E2x = E2* sin(45°) = 127, 176 N/C

As the Field strength components have the same Amount, yet oppose direction they substract themselvs to Zero, E1y-E2y = 0 ; E1x-E2x = 0. So the Overall Field strength in Point A is Zero.

At the second part i suppose to calculate the Electric Force, if at Point A is a Charge q3= 100nC.
Fe1 = k * q1 * q3 / r^2 = 1,7985*10^-5 N
Fe2 = k * q1 * q3 / r^2 = 1,7985*10^-5 N.

The Overall force Fe^2 = Fe1^2 + Fe2 ^2 => Fe = 2,54 * 10^-5.

Is this the right way to calculate it? I am open for every Suggestion.

Kind Regards

 

[Chandra Prayaga]

As the Field strength components have the same Amount, yet oppose direction they substract themselvs to Zero, E1y-E2y = 0 ; E1x-E2x = 0. So the Overall Field strength in Point A is Zero.

I can see that E_1x and E_2x are opposite and add to zero. Why do the y components add to zero? There is a problem in how you are treating the components. The components of a vector are algebraic quantities. E_1x and E_2x do not have the same sign.
For the second part, the two forces on the point charge at the point A are vectors. They should be added as vectors. You added their magnitudes. Your diagram is showing the forces correctly, but you did ot follow it up with the correct method of vector addition.

 

[psy]

Hello

So i have made the following correction:

r= 0,0707m

E1 = E2 = 17985,4 N/C

E1y = E1 * cos(-45°)= 12717,6 N/C
E2y = E2 * cos(45°)=12717,6 N/C
E1x = E1 * sin(-45°) = -12717,6 N/C
E2x = E2* sin(45°) = 12717,6 N/C

Ey = E1y+E2y = 25435.2 N/C
Ex = E1x+ E2x = -12717,6 N/C+12717,6 N/C = 0.

So the Electric field strength is E = Ey + Ex = 25435.2 N/C + 0 = 25435.2 N/C

In the second part, for calculating the force in case there is a 100nC Point Charge at the given spot :

F= q*E =100*10^-9C * 25435.2 N/C = 25,43* 10^-3 N.

Is this the correct way?

Kind Regards

 

[Chandra Prayaga]

You should really read up about how to add and subtract vectors from your textbook. For any vector V (bold font)
V ≠ V_x + V_y
The left hand side is a vector, with a direction. The components on the right hand side are not vectors. They are algebraic quantities. You must use either unit vectors, or specify both magnitude and direction to write a vector.

 

[psy]

IEI = sqrt( Ey^2 + Ex^2) = 25435.2 N/C ?

 

[Chandra Prayaga]

And the direction. Same with the force.