Electrostatics homework problem help

[Robin288]

Summary:: I been stuck on this problem from past 4 months. I am completely done. I am getting no idea. Even my professor couldn't have helped me. Can anyone please help me?

 

[ergospherical]

In your studies have you come across how to use Gauss' law and Ampère's law to find electric and magnetic fields in highly symmetric geometries, like this one?

 

[berkeman]

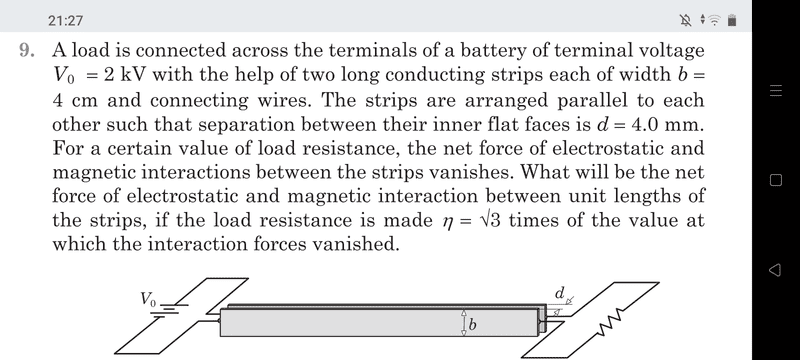
Summary:: I been stuck on this problem from past 4 months. I am completely done. I am getting no idea. Even my professor couldn't have helped me. Can anyone please help me?

View attachment 295945

There are several things going on in that problem. Please list what you think are the "Relevant Equations" so that we can discuss it more. You must always show us your work before we can offer tutorial help here at PF.

 

[Robin288]

Yeah but I have not much practice any starting hint would be much appreciated?

In your studies have you come across how to use Gauss' law and Ampère's law to find electric and magnetic fields in highly symmetric geometries, like this one?

Orah

 

[ergospherical]

Why don't you first try to put a value on the electric field strength between the plates, under the assumption that it is uniform in the gap? And once you've done that, try and think of a suitable Ampèrian loop to find the magnetic field strength between the plates (once again under the assumption that it is uniform in the gap, and zero elsewhere). These justifications are okay in the regime $d \ll b$.

 

[berkeman]

Yeah but I have not much practice any starting hint would be much appreciated?

I did give you a hint -- write the relevant equations please. What class is this for? What have you covered in class so far that seems like it might be applicable?

 

[Steve4Physics]

Yeah but I have not much practice any starting hint would be much appreciated?

Orah

From extensive research I have just discovered that 'Orah' is Serbo-Croation for 'walnut'.

 

[berkeman]

From extensive research I have just discovered that 'Orah' is Serbo-Croation for 'walnut'.

Hmm, but it's also related to a school resource website, so this could be a spam setup. Let's see what the OP has to say next...

 

[Fred Wright]

1. No electrostatic forces.
2. For no magnetic force load resistance $=\infty$.
3. $\sqrt{3}\infty=\infty$

 

[Steve4Physics]

1. No electrostatic forces.

I don’t want to give too much away before the OP has made a contribution. But it's worth reflecting on the correctness of the above point 1.

{Typo's corrected.]

 

[haruspex]

Yeah but I have not much practice any starting hint would be much appreciated?

Orah

Think of the pair of strips as a capacitor. What relevant equations then apply?

 

[Alex Schaller]

Summary:: I been stuck on this problem from past 4 months. I am completely done. I am getting no idea. Even my professor couldn't have helped me. Can anyone please help me?

View attachment 295945

The circuit resembles an EMF connected in parallel with a capacitor, which is then connected also in parallel with a resistor.

The voltage on the capacitor is equal to the applied EMF voltage.
The capacitor resembles a parallel plate capacitor. Its capacitance (per unit length) is readily available on any textbook (depends on the geometry of the plates and on the dielectric material between the plates, which in this case is air).

So far we've got voltage and capacitance per unit length on the capacitor, from there we can find out the charge per unit length (check any textbook for the appropriate formula).
The electric field E between the plates can be found provided we know the voltage and distance between the plates (which we already know).

So we have the electric field E between the plates, and the charge per unit length.
It would be straightforward to believe that by multiplying the electric field E by the charge per unit length we would get the force per unit length acting on the capacitor's plates; but we would be lead astray because the electric field that we had found corresponds to the electric field inside the capacitor (in the gap), whereas the force on any plate is equal to the charge on that plate multiplied by the electric field exerted by the other plate (the field has to be external to the charge, not generated by the same charge - otherwise it would be seen as trying to lift yourself by pulling up your shoelaces) . It is not difficult to check that the electric field of one of the plates is equal to half of the electric field which is in the gap between the plates.

So far we can find the electric force per unit length acting on any of the capacitor plates (as per Newton's third law they are equal).
What do you think so far?

Do you think you can handle to find the remaining magnetic force per unit length (it should correspond to a same magnitude than the electric force per unit length provided the net force is to vanish, wouldn't you agree)?

If you can catch up, you could deduce the current I from the magnetic force per unit lenght, and from there the resistance R. What follows should be a piece of cake.
Regards,
Alex

 

[kuruman]

A direct way of finding the force is to use $F=-\dfrac{\partial U_e}{\partial z}$ where $U_e=\frac{1}{2}CV^2$ is the energy stored in the capacitor and $z$ is the plate separation.