- #1
guyvsdcsniper
- 264
- 37
- Homework Statement
- The goal of this problem is to find the total electrostatic energy stored in a uniformly charge
sphere of radius R and total charge Q. Note that the charge is uniformly distributed throughout the whole volume –this is not a shell.
Express your answer in terms of Q, R, and constants of nature. There are many different ways to do this, you might want to use two different methods so you can check your result.
- Relevant Equations
- W= ϵ /2 ∫ E²dt
I solved this problem on my own using the Energy formula. When I compared my answer to online answers (attached) as well as the griffiths solution manual, I noticed they also include the Electric field outside the sphere into their calculations. I did not and only use the Electric Field inside.
Am I wrong for just considering the Electric Field inside the sphere? The problem explicitly states "find the total electrostatic energy stored IN a uniformly charge sphere". I don't see how E outside is relevant to the question.
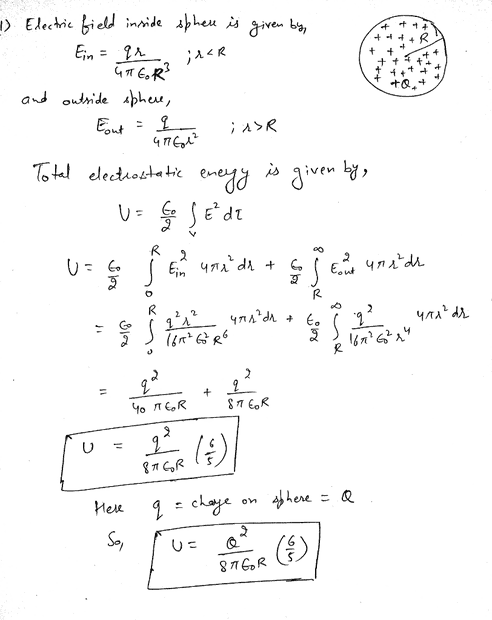
Am I wrong for just considering the Electric Field inside the sphere? The problem explicitly states "find the total electrostatic energy stored IN a uniformly charge sphere". I don't see how E outside is relevant to the question.