Ensemble vs. time averages and Ashcroft and Mermin Problem 1.1

[EE18]

Homework Statement

Please see the attached photo.

Relevant Equations

See below.

The question is as seen below:

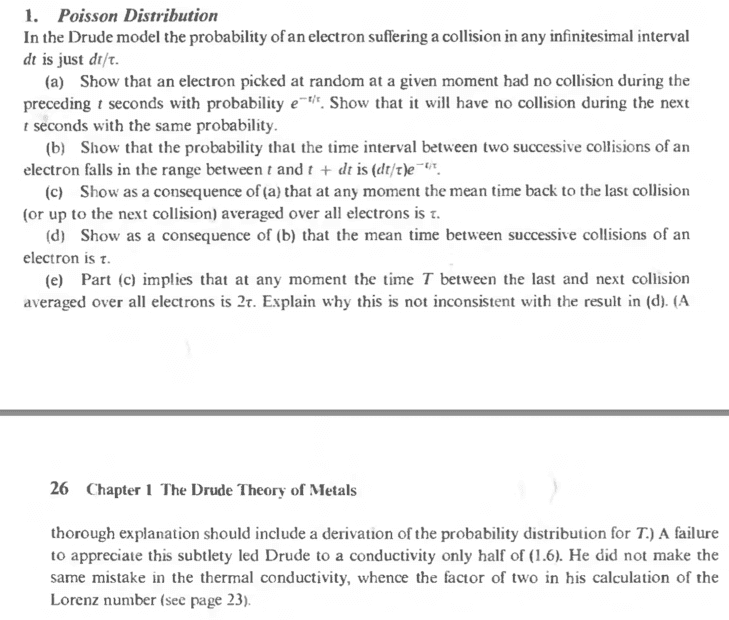
My attempt (note that my questions are in bold below) is below. Please note that I am self-studying AM:

(a) By the independence of any interval $dt$ of time and time symmetry, we expect these two answers are the same (Is there any way to make this rigorous?). Divide the interval $[0,t]$ into $N$ intervals. Then the probability of no collision in $[0,t]$ is equal to the probability that there is no collision in each and every interval $[t_i,t_i+t/N]$. Since each interval is statistically independent, we compute this probability (which we call $P_{nc}(t)$) as the product of the probability of no collision in each interval, each of which is of length $t/N$ (i.e. these are i.i.d. intervals):
$$
P_{nc}(t) \equiv \lim_{n \to \infty} \left(1 - \frac{t}{N\tau}\right)^N = e^{-t/\tau}.
$$
We emphasize again that this is the probability that a given electron has not collided over the previous time $t$, which is the same probability as that given electron not colliding over the future time $t$.

(b)
The probability of the event that the time interval between collisions of an electron is $t$ can be argued (It's not clear to me why this should be the same as the probability that a given electron -- not necessarily just collided -- has time $t$ between two of its collisions.) to be the same as the probability of an electron which has just collided colliding again between $t$ and $t+dt$. But this latter event is precisely the event $A$ that the electron does not collide in $[0,t]$ and $B$ also does collide in $[t,t+dt)$. $P(A)$ is known from a), while $P(B) = dt/\tau$ from Drude Axiom 4. Thus, by the independence of different time intervals, we have $P(A\&B) = P(A)P(B) = P_{nc}(t)dt/\tau = e^{-t/\tau}dt/\tau.$

For (c), (d), and (e), I am at a bit of a loss. I have read somewhere that I cannot seem to find now that perhaps this has something to do with the difference between an ensemble vs. time average, but it seems to me like what's really going on is that there is a different random variable under investigation in (c) and (d).

 

[TSny]

My attempt (note that my questions are in bold below) is below. Please note that I am self-studying AM:

(a) By the independence of any interval $dt$ of time and time symmetry, we expect these two answers are the same (Is there any way to make this rigorous?). Divide the interval $[0,t]$ into $N$ intervals. Then the probability of no collision in $[0,t]$ is equal to the probability that there is no collision in each and every interval $[t_i,t_i+t/N]$. Since each interval is statistically independent, we compute this probability (which we call $P_{nc}(t)$) as the product of the probability of no collision in each interval, each of which is of length $t/N$ (i.e. these are i.i.d. intervals):
$$
P_{nc}(t) \equiv \lim_{n \to \infty} \left(1 - \frac{t}{N\tau}\right)^N = e^{-t/\tau}.
$$
We emphasize again that this is the probability that a given electron has not collided over the previous time $t$, which is the same probability as that given electron not colliding over the future time $t$.

This looks good to me.

The reason we expect the same result for the preceding $t$ seconds and for the next $t$ seconds is that the calculations for the two cases are essentially identical. For the preceding $t$ seconds, you would work with the time interval $[-t, 0]$; for the future $t$ seconds, you work with the interval $[0, t]$. Either way, you can break the interval into N subintervals and proceed as you did. So, unless I’m overlooking something, it should be clear that the probability of no collision during the preceding $t$ seconds should equal the probability of no collision during the next $t$ seconds.

(b)
The probability of the event that the time interval between collisions of an electron is $t$ can be argued (It's not clear to me why this should be the same as the probability that a given electron -- not necessarily just collided -- has time $t$ between two of its collisions.) to be the same as the probability of an electron which has just collided colliding again between $t$ and $t+dt$. But this latter event is precisely the event $A$ that the electron does not collide in $[0,t]$ and $B$ also does collide in $[t,t+dt)$. $P(A)$ is known from a), while $P(B) = dt/\tau$ from Drude Axiom 4. Thus, by the independence of different time intervals, we have $P(A\&B) = P(A)P(B) = P_{nc}(t)dt/\tau = e^{-t/\tau}dt/\tau.$

Again, this looks good. I’m not sure I understand your bold-faced concern. For part (b), I don’t see why you are concerned with an electron "not necessarily just collided”.

For (c), (d), and (e), I am at a bit of a loss.

For part (c) you are picking an arbitrary instant of time which we can call the “present time”. Then, for each electron, there is a time interval $t$ from the present time to the electron’s next collision. The values of $t$ for the different electrons will be distributed with some probability distribution $P(t)$ such that $P(t)dt$ is the probability that the time interval from the present time to the next collision lies between $t$ and $t+dt$. Using part (a) and using reasoning similar to that used in part (b), you can find an explicit expression for $P(t)$. Then you can use $P(t)$ to find $\langle t \rangle$, where $\langle t \rangle$ is the time interval between the present time and the next collision averaged over all the electrons.

Similarly, you can find the average time back to the previous collision.