Epsilon-Delta Limit Definition

[JamesGold]

I know, I know, this topic has already been beat to death, but I'm still having a hard time understanding it despite having already read several forum threads and educational articles.

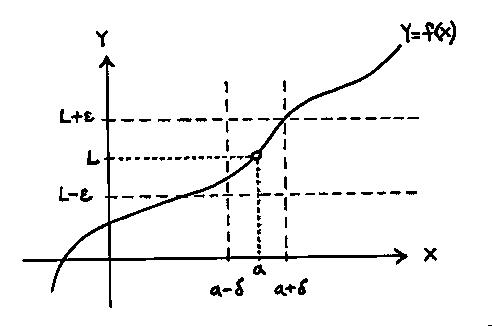
Intuitively, the definition is stating that no matter how narrow we choose to make the "epsilon band" surrounding f(x) = L, there will always be a "delta band" surrounding x = a that contains the x-values that correspond to all the f(x) values contained within the epsilon band. What this is effectively saying is that the function curve is defined no matter how close it gets to x = a. Am I at least partly correct?

Then, when we get down to the nitty gritty rigorous definition and define delta in terms of epsilon, is that the equivalent of proving that the limit exists? Is the fact that there exists a relationship between delta and epsilon that satisfies the definition proof enough that the limit exists?

I'm searching for a bridge that connects the intuitive definition with the rigorous definition but having I'm having a difficult time finding one. Basically, what I'm asking is: How exactly does the epsilon-delta ordeal prove that the limit exists?

 

[I like Serena]

Welcome to PF, JamesGold!

You have it exactly right.

How do you know the limit exists? Just because what you mention is exactly the definition of a limit.

 

[JamesGold]

Great. Thank you! I had a little more time to mull it over and I think I've got it now.

 

[JamesGold]

Why does my calculus textbook have me first pick a value for epsilon and then determine delta from that? Shouldn't it be the other way around? x is the independent variable, after all.

"No matter how close x gets to a there will always be a corresponding f(x)-value" sounds more intuitive than "no matter how close f(x) gets to L there will always be a corresponding x-value". You need the x first to determine the f(x)!

 

[I like Serena]

Why does my calculus textbook have me first pick a value for epsilon and then determine delta from that? Shouldn't it be the other way around? x is the independent variable, after all.

The point is that you can get arbitrarily close to the limit L as long as you get close enough to a.

Suppose you could find an epsilon such that you can not get that close to L, no matter what you picked for x!

 

[JamesGold]

The point is that you can get arbitrarily close to the limit L as long as you get close enough to a.

Right, yes. I just think it might be more intuitive to think of it as "as x approaches a, f(x) approaches L" as opposed to the reverse, "as f(x) approaches L, x approaches a". It follows that we should define delta before we do epsilon. But it works either way, right? If we say the limit of 2x as x approaches 2 is 4, then epsilon = 2(delta) and delta = epsilon / 2; it doesn't matter which one we define first.

Suppose you could find an epsilon such that you can not get that close to L, no matter what you picked for x!

I'm sorry, I'm having a hard time understanding this. Do you think you could rephrase it?

 

[Fredrik]

Is the fact that there exists a relationship between delta and epsilon that satisfies the definition proof enough that the limit exists?

I'm searching for a bridge that connects the intuitive definition with the rigorous definition but having I'm having a difficult time finding one. Basically, what I'm asking is: How exactly does the epsilon-delta ordeal prove that the limit exists?

This seems to indicate some sort of misunderstanding. The "epsilon-delta ordeal" is the definition of what we really mean when we say that the limit exists. If we define the word "pmmmf" to mean "x=2", it doesn't make sense to ask how that definition proves that x=2. It doesn't. It just explains what people who use the word "pmmmf" really mean when they say it.

Maybe these comments that I posted in another thread will be helpful.

Let's consider a particularly simple sequence to illustrate the idea behind the definition of a limit of a sequence: 1/2, 1/3, 1/4,... The terms in this sequence get smaller and smaller. You might think that it's obvious that it goes to 0, or that it's obvious that a smart math guy can prove that it goes to 0, but it's not. It's impossible to even attempt a proof until we have defined what it means for something to go to 0. So we have to define what the statement "1/2, 1/3, 1/4,... goes to 0" means, before we can attempt to prove that it's true.

This is the standard definition: "1/n goes to 0" means that "for every positive real number ε, there's a positive integer N, such that for all integers n such that n≥N, we have |1/n| < ε". With this definition in place, it's quite easy to prove that "1/n goes to 0" is a true statement. What I want you to see here, is that we chose this definition to make sure that this statement would be true. The first mathematicians who thought about how to define the limit of a sequence might have briefly considered definitions that make the statement "1/n goes to 0" false, but they would have dismissed those definitions as irrelevant, because they fail to capture the idea of a limit that they already understood on an intuitive level.

So the real reason why 1/n goes to 0 is that we wanted it to!.​

Edit: My posts in this old thread might be useful too.

 

[Studiot]

Hello James,

Just as there is more than one possible route from A to B, there is more than one posasible sequence of presentation (development) of analysis in maths.

It is best to follow one or another and not mix them up as something that is stated or proved in one presentation may arrive later in another.

I would be therefore be helpful if you could indicate your course eg you text and what you have already covered. Particularly have you covered open and closed sets or intervals and bounded v unbounded sets or intervals?

Modern treaments introduce epsilon delta quite early.
Here is an extract older treatment that does not introduce it till later.

go well

 

[I like Serena]

Right, yes. I just think it might be more intuitive to think of it as "as x approaches a, f(x) approaches L" as opposed to the reverse, "as f(x) approaches L, x approaches a". It follows that we should define delta before we do epsilon. But it works either way, right? If we say the limit of 2x as x approaches 2 is 4, then epsilon = 2(delta) and delta = epsilon / 2; it doesn't matter which one we define first.

I'm sorry, I'm having a hard time understanding this. Do you think you could rephrase it?

Let's consider an example: the heavy step function.
This function is -1 if x < 0 and +1 if x > 0.

So following your reasoning, we let x approach zero, and see what function does and pick an appropriate epsilon.
Let's say delta = 0.1. Now I can (always) pick epsilon = 2.
And indeed, for an x within this delta the function is within epsilon.
I think you can see how this will not work, don't you?

Now we reverse it, as it is supposed to be, in accordance with the definition of a limit.
We pick an epsilon = 0.1. Now however I pick delta, the function will never be within epsilon.
This is what we expect since the heavy step function has no defined limit for x = 0.

 

[SteveL27]

Why does my calculus textbook have me first pick a value for epsilon and then determine delta from that? Shouldn't it be the other way around? x is the independent variable, after all.

"No matter how close x gets to a there will always be a corresponding f(x)-value" sounds more intuitive than "no matter how close f(x) gets to L there will always be a corresponding x-value". You need the x first to determine the f(x)!

To constrain f(x) to be close to L, we need to constrain x to be close to a. So you tell me how close you want f(x) to be to L; and I'll tell you how close x needs to be to a. You give me epsilon, I'll give you delta.

That's the intuition behind why we choose eps first. For every epsilon, there is a delta.

There's another magic trick being done though. The definition says, FOR ALL epsilon > 0. There's a couple of thousands of years of intellectual wrangling with infinitesimal quantities finessed by the universal quantifier.

The brilliance of the eps/delta idea is that instead of talking about "infinitely small" quantities that we can't really formalize, what we do instead is first develop a rigorous theory of the real numbers, then say that FOR ALL epsilon > 0 etc. Philosophically, there's quite a bit of magic going in in that "for all."

[Yes I know that infinitesimals have been formalized in modern math, but that's a side issue to this discussion]

Hope some of this helps. I do commend you for making a serious effort to understand the meaning of the definition of limit. You are very much doing the right thing in terms of how to learn math.

 

[JamesGold]

This is my attempt at patching together everything I've learned so far:

Less formal definition: No matter how close we want to make f(x) to L there exists a x that can get it there.

More formal: If f(x) lies within epsilon from L, where epsilon can be as large or small as we like, there will be a corresponding x within delta from a. This definition is two steps ahead of the less formal definition. We have defined just how close we want f(x) to be to L (within epsilon) and we have shown how close x must be to a (delta) to get f(x) where we want it to be. The trick is to find just how large delta must be for any given epsilon, no matter how large or small. This could take the form of an equation, such as epsilon = 1/2(delta). This equation effectively says that for any epsilon value we enter, no matter how large or small, there will always be a delta value half its size. It's not the "half its size" part that matters, it's the "there will always be a delta" part that matters.

Most formal:

If

[URL]http://www.math.ucdavis.edu/~kouba/CalcOneDIRECTORY/preciselimdirectory/img5.gif[/URL]

then

[URL]http://www.math.ucdavis.edu/~kouba/CalcOneDIRECTORY/preciselimdirectory/img6.gif[/URL]

All these symbols can be interpreted as "If x lies between a and delta (a.k.a. the number that x must lie within for f(x) to lie within epsilon), then f(x) lies between L epsilon." That almost seems like a no-brainer. Also, if we plug numbers into this definition, it magically pops out a relationship between epsilon and delta. How, exactly? The other thing that is causing me confusion is that the less formal and most formal definitions seem to be approaching the matter from different angles. The less formal definition talks about f(x) first and the most formal definition talks about x first. Can someone help me reconcile the two definitions? My brain is starting to malfunction after two days sitting against the grinding wheel. All this work to understand a proof that I probably won't even encounter ever again.

I would be therefore be helpful if you could indicate your course eg you text and what you have already covered. Particularly have you covered open and closed sets or intervals and bounded v unbounded sets or intervals?

Hey Studiot, I'm in a Calculus I college class. We're using the 6th edition of the Stewart textbook and we just learned the epsilon-delta definition yesterday. I don't think we've covered the stuff you mentioned, but I could be wrong.

 

[I like Serena]

Most formal would be:

$\forall \epsilon > 0 \quad \exists \delta > 0 \text{, such that }$
$$\forall x \text{ with } 0 < |x-a| < \delta: |f(x) - L| < \epsilon$$

By now I don't really know what else to explain.
It would just be more words.

I would recommend trying a number of examples of which you have a solution.
Try to do it in the "most formal" way.
I think after a while you'll get the hang of it and develop a feel what it's about.

 

[Fredrik]

The main difference between the actual definition and the "less formal definition" is that we don't just want f(x) to "get there". We also want it to "stay there". The "less formal definition" tells us e.g. that sin(1/x)→1 as x→0. This isn't what we want. We want the limit of sin(1/x) as x→0 to be undefined, not to equal every number in the interval [-1,1].

 

[Studiot]

Stewart huh?

He has written some very good stuff about calculus, though it is not liked by all.
He actually has several textbooks, but as far as I can tell, they are all variations of a common approach.
His approach is basically the same as in the extracts I posted so they are relevant. How did you get on with them?
I hoped the outline in the first might be helpful.

In the version of Stewart I have (Single Variable Calculus) he covers intervals and manipulation of inequalities on page 4.

The main difference between the actual definition and the "less formal definition" is that we don't just want f(x) to "get there". We also want it to "stay there".

Notice in the post by ILS that the inequalities are strict. This means 'greater than' rather than 'greater than or equal' to.

The upshot of that is noted in the first definition on p52 of the second extract I posted.

The limit point itself is excluded from the set/interval under consideration.

 

[SteveL27]

Most formal:

If

[URL]http://www.math.ucdavis.edu/~kouba/CalcOneDIRECTORY/preciselimdirectory/img5.gif[/URL]

then

[URL]http://www.math.ucdavis.edu/~kouba/CalcOneDIRECTORY/preciselimdirectory/img6.gif[/URL]

You are missing the most important point. The definition of limit says that

* For all epsilon > 0, you can find a delta such that the above holds.

If you leave that part out, you are missing the essence of the definition.

 

[Fredrik]

All this work to understand a proof that I probably won't even encounter ever again.

You know that what we've been discussing so far isn't a proof, right?

(It looks like you just typed the wrong word because you were tired or something, but you did make a similar mistake earlier. Just to be on the safe side: The definition proves exactly nothing. It tells you what mathematicians mean when they say "f(x)→y as x→a". Nothing more, nothing less).