Mech_LS24
- 148
- 16
- TL;DR Summary
- I can't figure out how to setup a equation for the angle position of a slider crank mechanism. As the mechanism is driven by an angular velocity, the angle (theta) changes constantly. Theta is the angle which rotates the crank. I would like find the angle α (alpha) during the rotation of crank.
Hi!
I would like to know how I could define an equation for α with given the two lengths of the rods and angle theta (θ). I sketched the situation below, the problem arises when the rod Lab has pasts the 180 degrees.
Like to hear.
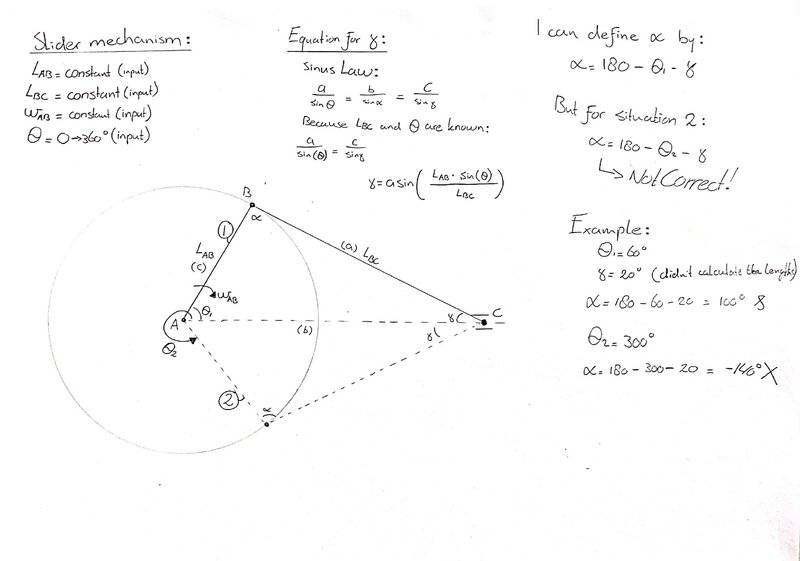
I would like to know how I could define an equation for α with given the two lengths of the rods and angle theta (θ). I sketched the situation below, the problem arises when the rod Lab has pasts the 180 degrees.
Like to hear.
