Equations of Motion for Pulley System with Belt and Spring Constants

In summary, the conversation is about drawing a 'free body diagram' of two pulleys connected by a belt to derive the 'equations of motion'. The issue being discussed is whether the 'tight' and 'slack' sides should be modeled in the same way and if this will affect the problem when considering the spring constants (K). The equations being used are Hooke's Law and Newton's 2nd Law, with variables such as force, mass, acceleration, displacement, and angular displacement. The solution attempts to use these equations to derive the 'k' spring constant from the 'E' Young's modulus.
  • #1
toolpusher123
64
0
Equations of Motion, help...

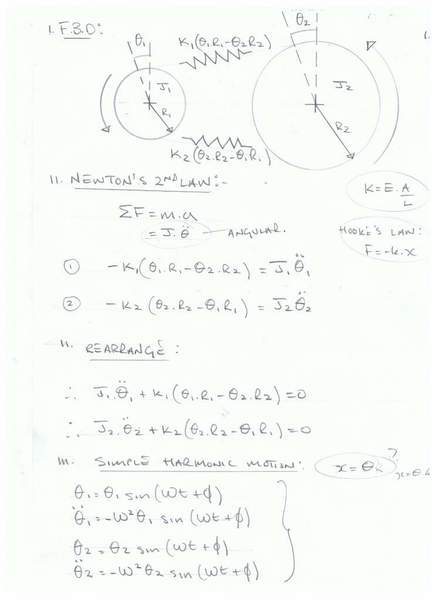
Homework Statement



I'm attempting to draw a 'free body diagram' of 2 pulley's connected by a belt (open configuration), and hence derive the 'equations of motion'.
The issue I'm having is in regard to, the 'tight' & 'slack' sides & wheather they should be modeled in exactly the same way? Will the difference enter the problem when I put the spring constants in (K)?


Homework Equations



Hooke's Law: F=-K.x
Newton's 2nd Law: ∑F=m.a ∴ T=J.θ"
F=Force
m=mass, J= mass moment of inertia
a=acceleration, θ"=angular acceleration,
x=displacement, θ=angular displacement
k=spring constant


The Attempt at a Solution



J1*θ"1+K1(θ1*r1-θ2*r2)=0
J2*θ"2+K2(θ2*r2-θ1*r1)=0

I've attached a drawing, it makes interpretation much easier, thanks...
Pulley's & Springs_1.jpg


Pulley's & Springs_2.jpg

 
Physics news on Phys.org
  • #2
Also, would this be the right equation to derive 'k', spring constant from 'E' Young's modulus, K=E*A/L (area/length)?
 

FAQ: Equations of Motion for Pulley System with Belt and Spring Constants

What are the three equations of motion?

The three equations of motion are:

  • First Equation: v = u + at, where v is the final velocity, u is the initial velocity, a is the acceleration, and t is the time
  • Second Equation: s = ut + 1/2at^2, where s is the displacement
  • Third Equation: v^2 = u^2 + 2as, which is derived from the first two equations

How are the equations of motion derived?

The equations of motion are derived from the fundamental laws of motion developed by Sir Isaac Newton. The first law states that an object will remain at rest or in uniform motion unless acted upon by an external force. The second law states that the force applied to an object is directly proportional to its mass and acceleration. The third law states that for every action, there is an equal and opposite reaction. By using these laws and basic calculus, the equations of motion can be derived.

What is the significance of the equations of motion?

The equations of motion are significant because they allow us to mathematically describe the motion of objects. They are used in many fields of science and engineering, such as mechanics, physics, and aerospace. They also help us understand the relationship between displacement, velocity, acceleration, and time.

Can the equations of motion be used for all types of motion?

No, the equations of motion are only applicable for motion in a straight line with constant acceleration. They cannot be used for motion with changing direction or non-uniform acceleration. In these cases, calculus and other mathematical techniques must be used to describe the motion.

How can the equations of motion be applied in real-life situations?

The equations of motion can be applied in real-life situations to predict the motion of objects and to design systems that involve motion. For example, engineers use these equations to design roller coasters, cars, and airplanes. They can also be used in sports to analyze the motion of athletes, and in astronomy to calculate the movement of planets and other celestial bodies.

Similar threads

Creating a One Shaft Model for a Geared System w/ Inertia
Replies
1
Views
2K
Engineering The value of "k" for spring constant, is the book answer incorrect?
Replies
3
Views
1K
How to get transfer function (freq. domain) from Newton's 2nd Law Eqn
Replies
2
Views
3K
Equations of motion for disk and spring system
Replies
1
Views
3K
How Do You Calculate Spring Properties for Effective Landing Gear Design?
Replies
10
Views
2K
Equations of Motion w/ spring scale
Replies
2
Views
2K
Equation of Motion of a System of Springs (System Dynamics)
Replies
1
Views
2K
Kinematics Belt and Pulley Problem
2
Replies
40
Views
7K
Statics Problem involving blocks, rope, pulleys and springs....
Replies
2
Views
3K
Absolute motion analysis of pulley-spring system
Replies
10
Views
635

Hot Threads

Recent Insights

Back
Top