Equations of Motion for Torsional Inverted Pendulum on a moving cart

[hamiltoncusin]

TL;DR Summary

Equations of Motion for Torsional Inverted Pendulum on a moving cart using Lagrangian

Hello
I am trying to figure out the equations of motion for the following scenario: a mass m is held upright on by a massless rod mounted on a cart that is being pulled by a linear force. The caveat is that the rod is mounted on the cart via a torsional spring which causes a restorative torque as the rod swinges. Hopefully the image below can illustrate the situation

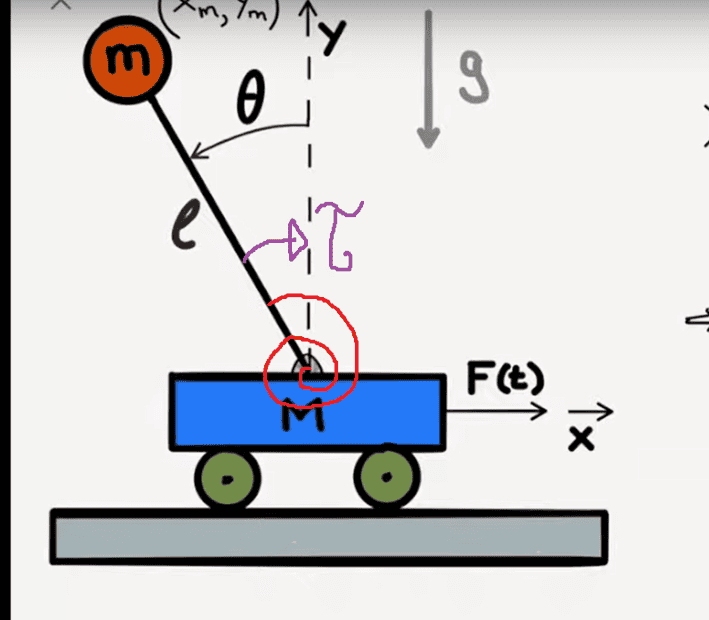
I think I figure out the equations of motion using Newton's equations and the balance of forces, however, I believe it will be easier to use Lagrangian equations. I found the video on the link below (that's where the drawing above comes from) which explains really well how to obtain the Lagrangian equations for a system without the torsional spring. My questions is then:

- I know I need to include the torsion string potential energy term in the Lagrangian, but do I need to include any generalized force related to Theta, as the video shows for x?

Here is the video:

Thanks everyone for the help

 

[Mishal0488]

I may be mistaking your question to some extent, however you do not need to model generalized force to the theta component, unless you are actually applying a force to the rod, in which case the loading becomes a little more complicated. Your virtual angle needs to be converted into an actual displacement. This implies you would need to multiply the virtual angle by the total length of your rod.

 

[Orodruin]

There is no external force being applied to the theta coordinate. So no.