Equilibrium of a Rod Problem: Find Minimum Distance x

[burnst14]

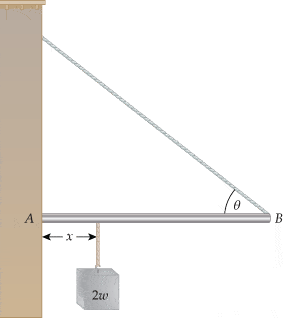
Problem Statement: As shown in the figure below, a uniform beam is supported by a cable at one end and the force of friction at the other end. The cable makes an angle of θ = 30°, the length of the beam is L = 1.50 m, the coefficient of static friction between the wall and the beam is μs = 0.420, and the weight of the beam is represented by w. Determine the minimum distance x from point A at which an additional weight 2w (twice the weight of the rod) can be hung without causing the rod to slip at point A.

Relevant Equations:
Ff = μN
Ʃτ = 0
ƩF = 0

Solution Attempt:
I'm not really sure where to start. Does Fn (normal force) come from the weight of the rod multiplied by cos(30) due to the angle of the cable holding it against the wall? And once I find Fn, I multiply that by μ, giving the upward force of friction. Then, placing the rotational axis at the end of the rod that the cable is attached to (because it's not moving, I can place the axis anywehere) and set Fn equal to the weight of the object being attached multiplied by the distance from the wall. Would that work?

 

[voko]

You just need to go about the problem systematically.

Write down the equation for x-components of the forces.

Write down the one for y-components.

Then for moments/torques.

You are going to have a bunch of known and unknown forces in those equations. Of those, the normal force at A and friction at A will be related with the coefficient of friction.

 

[burnst14]

Okay so:

ƩFy: F_f + F_Bsin30-2w-w = 0
ƩFx: F_N - F_Bcos30 = 0
Ʃτ: -w(0.75) - 2wx_m + F_Bsin30(1.5)

Is that correct?

 

[voko]

Assuming the third formula ends with " = 0 ", that looks good.

 

[burnst14]

Alright so all of that is correct, but now what? We don't have most of those variables and the only ones we can solve for are F_F/F_N. How do we relate w to F_N? Everything has to be in terms of "w" as it stands.

What am I missing?

 

[voko]

Observer that once you relate friction and the normal force, the first to equations can be solved, giving you all the forces in the system. But you still have the third one to satisfy.

 

[burnst14]

So rewriting ƩF_y we get:

μF_N - 2w - w = -F_Bsin30 → -(μF_N - 2w - w) = F_Bsin30

And rewriting ƩF_x we get:

F_N = F_Bsin30

We set these equal which gives:

-(μF_N - 2w - w) = F_N

then

-μF_N + 2w + w = F_N

then

(-0.420)F_N + 2w + w = F_N

then

What? Nothing cancels so we can't solve for anything.

 

[voko]

And rewriting ƩF_x we get:

F_N = F_Bsin30

It was F_Bcos 30 originally.

then

(-0.420)F_N + 2w + w = F_N

then

What? Nothing cancels so we can't solve for anything.

How so? Assuming it is correct (which it is not), this is the same as 3w = 1.420 F_N. Surely this can be solved for F_N.

 

[Gank]

Is the answer 0.9275m?

 

[burnst14]

Oh yeah, so it really doesn't work then.

How so? Assuming it is correct (which it is not), this is the same as 3w = 1.420 F_N. Surely this can be solved for F_N.

Now I feel really dumb. How do you solve that for anything, but an answer in terms of w?

But back to the problem, I'm at a loss of what to even try. You say relate F_N and μ, but I can't.

If I try setting it equal with F_N rather than F_B...

I get:
F_Bcos30 = (F_Bsin30 - 3w) / -μ

Does cos30/sin30 cancel somehow that I'm unaware of? That would help with one of the variables. Actually that would help everything! Waitwaitwaitwaitwait...

[F_Bcos30] / [F_Bsin30] = -3w/ -μ

substitute in for μ:

[F_Bcos30] / [F_Bsin30] = -3w/ -0.420

Cancel F_B and multiply by -0.420:

-0.420(sin30/cos30) = -3w

Divide by -3:

(0.420/3)(sin30/cos30) = w

RIGHT?

 

[burnst14]

Is the answer 0.9275m?

I don't have the answers. It's a Web Assign, if you'v heard of it. Basically online physics homework that allows for 5 solution attempts.

 

[Gank]

The way I did mine was:

We need to find the Fb in terms of w then we can eliminate it from the final answer as all the terms in the torques will have w. So I found 0.42Fn=Ff and that Fn=Fbcos30. From there i found Ff in terms of Fb and then put this into Fy to find Fb in terms of w. From here i substituted this into the torque equation and simplified

 

[Gank]

@voko ?

 

[Gank]

How many more answer attempts do you have?

 

[Gank]

Is there any help available on this thread?

 

[voko]

F_Bcos30 = (F_Bsin30 - 3w) / -μ

Does cos30/sin30 cancel somehow that I'm unaware of? That would help with one of the variables. Actually that would help everything! Waitwaitwaitwaitwait...

[F_Bcos30] / [F_Bsin30] = -3w/ -μ

I think you need to brush up on your algebra skills. When you have $a F_B = (b F_B + c)/d$, you should have no problem doing this: $$

a F_B = (b/d) F_B + (c/d)

\\

a F_B - (b/d) F_B = c/d

\\

(a - b/d)F_B = c/d

\\

F_B = \frac {c/d} {a - b/d}

\\

F_B = \frac {c} {ad - b}
$$

 

[Gank]

Burnst14 got any answers?

 

[voko]

The way I did mine was:

We need to find the Fb in terms of w then we can eliminate it from the final answer as all the terms in the torques will have w. So I found 0.42Fn=Ff and that Fn=Fbcos30. From there i found Ff in terms of Fb and then put this into Fy to find Fb in terms of w. From here i substituted this into the torque equation and simplified

This approach should work.

 

[Gank]

Cheers Voko. Sorry for being quite brash - I get really into problem solving

 

[voko]

The way I did mine was:

We need to find the Fb in terms of w then we can eliminate it from the final answer as all the terms in the torques will have w. So I found 0.42Fn=Ff and that Fn=Fbcos30. From there i found Ff in terms of Fb and then put this into Fy to find Fb in terms of w. From here i substituted this into the torque equation and simplified

There is also another approach, somewhat more complex but "more correct". Note that by letting $F_f = \mu F_n$ we assume that the friction is maxed out. But we do not really know if it is going to be maxed out at a minimum or at a maximum of $x$.

So the more correct approach would involve not letting $F_f = \mu F_n$ just yet, and instead solve the system of three equations in three variables $F_B, \ F_f, \ F_n$ and one parameter $x$. Then find the range of $x$ where $F_f \le \mu F_n$. You could try that.

 

[burnst14]

I think you need to brush up on your algebra skills. When you have $a F_B = (b F_B + c)/d$, you should have no problem doing this: $$

a F_B = (b/d) F_B + (c/d)

\\

a F_B - (b/d) F_B = c/d

\\

(a - b/d)F_B = c/d

\\

F_B = \frac {c/d} {a - b/d}

\\

F_B = \frac {c} {ad - b}
$$

Yes I could do that, but in this case "c" is another variable, "w". So my solution would be in terms of w like I said. Wouldn't it?

 

[voko]

Yes I could do that, but in this case "c" is another variable, "w". So my solution would be in terms of w like I said. Wouldn't it?

Yes, you will end up with $F_B$ in terms of $w$. Which is what you want, because the torque equation is also in terms of $w$, so you could expect it will cancel out.

 

[burnst14]

OOOOOOOOOOOHHHHHHHHHHHH!

Dang it. I was so focused on solving for a number that I completely forgot about the possibility of substitution and solving it as a system. Wow. Sorry. Okay.

 

[burnst14]

Okay I'm going to bed, I'll work on this in the morning and post what I got and let you know if I got it correct. Thanks for the patience.

 

[burnst14]

If absinθ = c
does (asinθ)b = c?

 

[burnst14]

Nevermind. I just proved it does.

 

[burnst14]

Alright I got it wrong. Here's what I did:

Using that algebra step, I got:

F_B = 1.171w

Then I substituted that in for F_B in:

Ʃτ: -w(0.75) - 2wx_m + F_Bsin30(1.5)

Giving:

-w(0.75) - 2wx_m + (1.171w)sin30(1.5) = 0

Combine like terms and multiply out:

0.13w = 2wx

Divide over:

x = 0.065m

This is wrong according to Web Assign. It seems too small, but who am I to say so. So where did I stray?

 

[voko]

I obtain $F_B = \frac {3w} {\mu \cos \theta + \sin \theta} \approx 3.473 w$.

 

[burnst14]

Alright I got the right answer using your value. I'm not sure how you got it though. That seems to be new to me.

[cos30-(sin30/-μ)]F_B = 3w/-μ

F_B = [(-3w/-μ)/(cos30-(sin30/-μ)]

F_B = (-3w/-μ)[(1/cos30)-(-μ/sin30)

F_B = (3w/μ)(sin30+μcos30/sin30cos30)

F_B = (3wsin30+3wμcos30)/(μsin30cos30)

Where do you go from there?

 

[burnst14]

Also what tool are you using to write your equations? It's much more clearly written.

 

[voko]

Alright I got the right answer using your value. I'm not sure how you got it though. That seems to be new to me.

[cos30-(sin30/-μ)]F_B = 3w/-μ

F_B = [(-3w/-μ)/(cos30-(sin30/-μ)]

This is almost exactly the equation I got. Just multiply the numerator and denominator by $\mu$.

F_B = (-3w/-μ)[(1/cos30)-(-μ/sin30)

This is wrong. You cannot go from $\frac {a} {b + c}$ to $a(\frac 1 b + \frac 1 c)$, these are not equal.

As for the tool, click the Quote button on my message, you will see some code there. That code is LaTeX. When you are in (advanced) reply screen, there is a $\sum$ button, which you can use to find codes for whatever math symbol you need. Or you can just search for a LaTex reference online.

 

[burnst14]

Alright thank you. Oh okay. I'll use that next time. Thanks for all the help. This forum is great.