- #1
connor8771
- 11
- 3
- Homework Statement
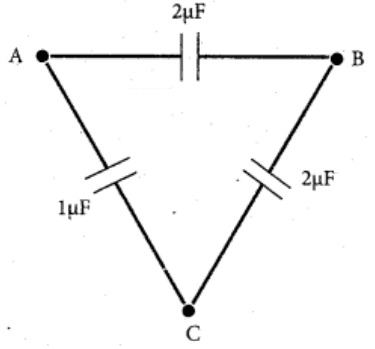
- There are three capacitors connected in a triangular network as shown in the picture. What is the equivalent capacitance across terminals a and c?
- Relevant Equations
- C=q/delta(V)
Hi, I'm struggling with this question. I feel like I don't even know where to begin. It seems to be a relatively simple calculation, but would the effective capacitance between A and C not just be 1 microFarad? Obviously that can't be the correct answer because such a simple observation wouldn't justify a question, but I can't figure out why it would be anything different. I understand how capacitance works but can't seem to even get a handle on what this question wants of me. Thanks in advance for any help.