PandaKitten
- 11
- 1
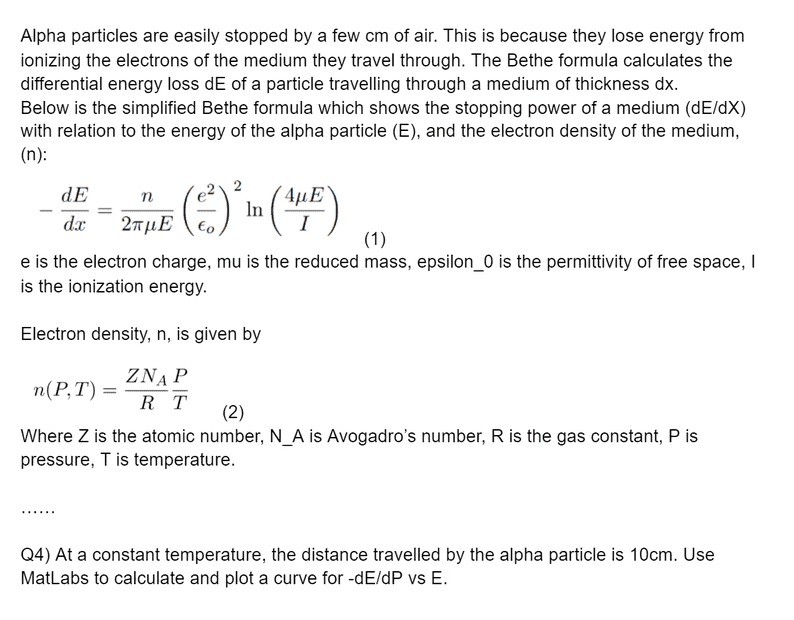
- Homework Statement
- Full question is below
- Relevant Equations
- Bethe's formula
The question is below. I tried reasoning that because x is constant, E is also constant however that gives me values in the range of 10^51. Then I tried to use numpy's ivp_solve function to solve the differential equation however I wasn't able to get that working either. Apparently I'm meant to use Taylor's expansion to estimate E however I'm not sure how I would do that.