Even with a whimsical mathematical usage, solutions are obtained!

[Z-10-46]

TL;DR Summary

Even with a whimsical mathematical usage, coherent solutions are obtained!

Hello everyone,

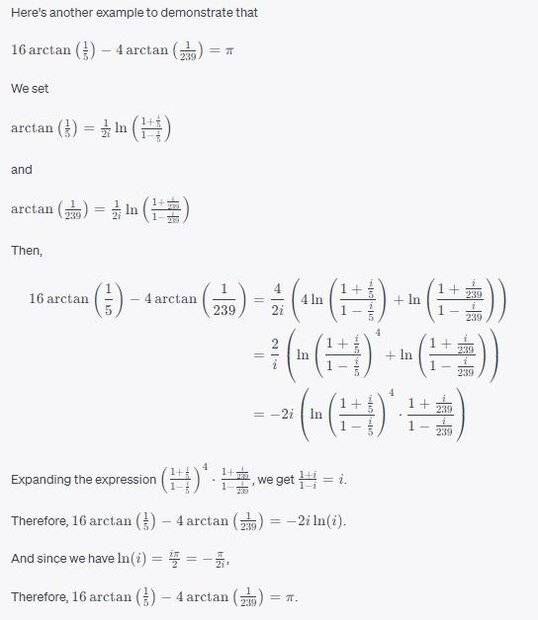
Here, we observe that the familiar properties of the real logarithm hold true for the complex logarithm in these examples.

So why does a whimsical mathematical use of real logarithm properties yield coherent solutions even in the case of complex logarithm?

 

[FactChecker]

As long as you are dealing with analytic functions, the real function is just a restriction of the complex analytic function. Two analytic functions can only be identical on the real line if they are identical.

 

[fresh_42]

As long as you are dealing with analytic functions, the real function is just a restriction of the complex analytic function. Two analytic functions can only be identical on the real line if they are identical.

The term analytical is very interesting. It is reserved for functions that have an expression as a series. And that was how mathematicians regarded all functions for a long time, as series.

 

[Svein]

The term analytical is very interesting. It is reserved for functions that have an expression as a series. And that was how mathematicians regarded all functions for a long time, as series.

When I studied complex analysis, "analytical" and "holomorphic" were assumed to mean more or less the same thing.

 

[fresh_42]

When I studied complex analysis, "analytical" and "holomorphic" were assumed to mean more or less the same thing.

Isn't that still the case?

 

[Svein]

Isn't that still the case?

I certainly hope so. But the definition used to be "functions that satisfy the Cauchy-Riemann equations".