- #1
chwala
Gold Member
- 2,756
- 390
- Homework Statement
- Kindly see the attached....
- Relevant Equations
- exact differential equations
I am looking at this and i would like some clarity...
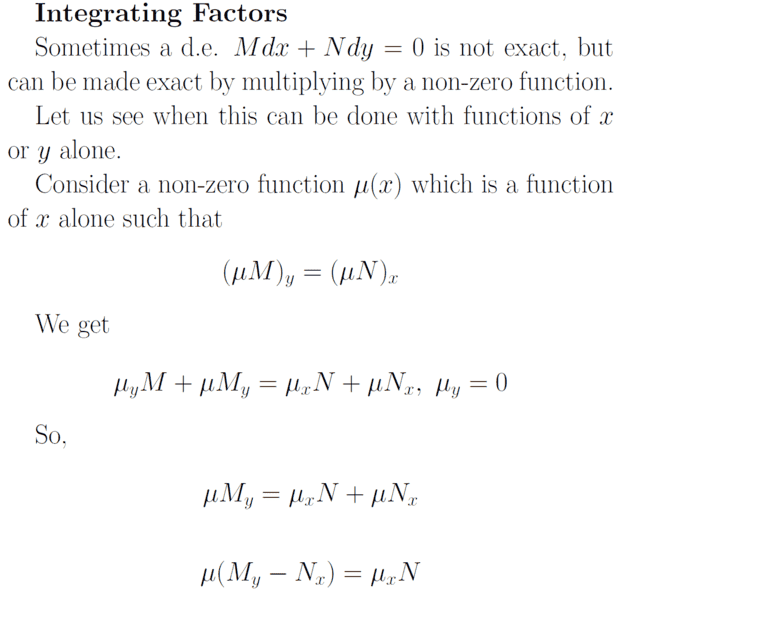 at the step where "he let" ##μ_y##=0" Could we also use the approach, ##μ_x##=0"?
at the step where "he let" ##μ_y##=0" Could we also use the approach, ##μ_x##=0"?
so that we now have,
##μ_y##M=μ(##N_{x} -M_{y})##... and so on, is this also correct?
so that we now have,
##μ_y##M=μ(##N_{x} -M_{y})##... and so on, is this also correct?
Last edited:
 ...##c## the constant in your post is simply equal to ##1##...ok cheers mate
...##c## the constant in your post is simply equal to ##1##...ok cheers mate