Example - Bland - Right Noetherian but not Left Noetherian

[Math Amateur]

I am reading Paul E. Bland's book, "Rings and Their Modules".

I am focused on Section 4.2: Noetherian and Artinian Modules and need some help to fully understand Example 4 on page 108 ... ...

Example 4 reads as follows:

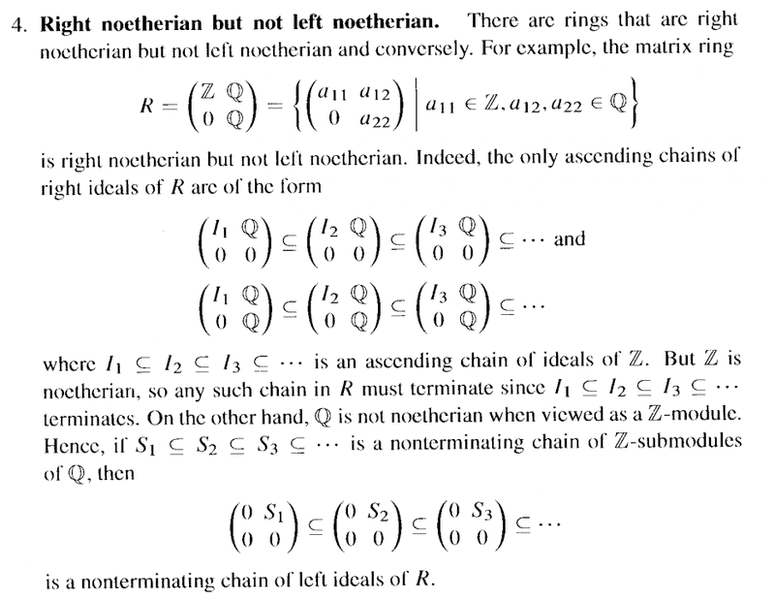
In the above text, I do not understand the proof that $R$ is not left Noetherian ... I hope someone can clearly explain the way the proof works ...To try to clarify my lack of understanding of the proof ... Bland, in the above text writes ... ..." ... ... On the other hand, $\mathbb{Q}$ is not Noetherian when viewed as a $\mathbb{Z}$-module.

Hence if $S_1 \subseteq S_2 \subseteq S_3 \subseteq$ ... ... is a non-terminating chain of $\mathbb{Z}$-modules of $\mathbb{Q}$, then$\begin{pmatrix} 0 & S_1 \\ 0 & 0 \end{pmatrix} \subseteq \begin{pmatrix} 0 & S_2 \\ 0 & 0 \end{pmatrix} \subseteq \begin{pmatrix} 0 & S_3 \\ 0 & 0 \end{pmatrix} \subseteq$ ... ... is a non-terminating chain of left ideals of $R$ ... ... "
My questions are as follows:What is the justification for regarding $\mathbb{Q}$ as a $\mathbb{Z}$-module ... indeed, couldn't we have done this while trying to prove/justify that $R$ was right Noetherian and ended up with a non-terminating chain of right ideals of $R$ ...Why is $\mathbb{Q}$ not Noetherian when viewed as a $\mathbb{Z}$-module ... ... ?Can someone please simply and clearly explain the proof of $R$ not being left Noetherian ... ... ?
Hope someone can help ...

Peter

 

[andrewkirk]

What is the justification for regarding $\mathbb{Q}$ as a $\mathbb{Z}$-module

It is easy to show that $\mathbb{Q}$ can be regarded as a $\mathbb{Z}$-module. All that is needed is to show that it satisfies the four module axioms.

Why is $\mathbb{Q}$ not Noetherian when viewed as a $\mathbb{Z}$-module ... ... ?

Because there exist non-terminating ascending chains of ideals, for instance the chain whose $k$th element is $2^{-k}\mathbb Z$.

 

[fresh_42]

What is the justification for regarding $\mathbb{Q}$ as a $\mathbb{Z}$-module

Hi Peter.

We consider $R$-left-modules $S_i$ of the form $\begin{bmatrix}0 & S_i \\ 0 & 0 \end{bmatrix}$
We simply choose them as an arbitrary chain $S_1 \subseteq S_2 \subseteq S_3 \subseteq \dots$ in $\mathbb{Q}$

Now all we are allowed to do, is multiply them with elements of $R$ from the left. Let's do it:
$$ \begin{bmatrix}\mathbb{Z} & \mathbb{Q} \\ 0 &\mathbb{Q}\end{bmatrix}\,\cdot\,\begin{bmatrix}0 & S_i \\ 0 & 0 \end{bmatrix}=\begin{bmatrix}0 & \mathbb{Z} \cdot S_i \\ 0 & 0 \end{bmatrix} $$
Therefore the ascending chain condition of the $S_i$ as left $\mathbb{Z}$-modules comes into play.

... indeed, couldn't we have done this while trying to prove/justify that $R$ was right Noetherian and ended up with a non-terminating chain of right ideals of $R$ ...

Do the multiplication from the right and see what happens.

Why is $\mathbb{Q}$ not Noetherian when viewed as a $\mathbb{Z}$-module ... ... ?

The short answer is Proposition 5.33. in Joseph J. Rotman's book: Advanced Modern Algebra.
The long one is to define $S_i := \mathbb{Z} \cdot \frac{1}{2^i}$ for $i \in \mathbb{N}$.

 

[Math Amateur]

Thanks for the help fresh_42 and Andrew ... help is much appreciated ...

... BUT ... still somewhat uneasy and perplexed by this example ...

One of the points I am uneasy about is that if we "view" $\mathbb{Q}$ as a $\mathbb{Z}$-module it is not Noetherian ... but if we "view" $\mathbb{Q}$ as a field then it is Noetherian ... ? ... can you explain how this makes sense ...

Maybe when thinking of $\mathbb{Q}$ maybe I should be thinking of $\mathbb{Z} \cdot \mathbb{Q}$ as the elements of the $\mathbb{Q}$ as a $\mathbb{Z}$-module would presumably be of the form $n \cdot \frac{a}{b}$ where $n \in \mathbb{Z}$ and $\frac{a}{b} \in \mathbb{Q}$ ... but ... then (?) on the other hand (?) ... ... $n \cdot \frac{a}{b}$ just gives us another "fraction" ... that is another member of $\mathbb{Q}$ ... ... there goes my idea that $\mathbb{Z} \cdot \mathbb{Q}$ would at least be something different from $\mathbb{Q}$ ... so the difference between Noetherian and non-Noetherian would not just depend weirdly on the view we take of $\mathbb{Q}$ ...

Hope you can clarify this issue for me ...

Peter

 

[fresh_42]

Thanks for the help fresh_42 and Andrew ... help is much appreciated ...

... BUT ... still somewhat uneasy and perplexed by this example ...

One of the points I am uneasy about is that if we "view" $\mathbb(Q)$ as a $\mathbb(Z)$-module it is not Noetherian ... but if we "view" $\mathbb(Q)$ as a field then it is Noetherian ... ? ... can you explain how this makes sense ...

Maybe when thinking of $\mathbb(Q)$ maybe I should be thinking of $\mathbb(Z) \cdot \mathbb(Q)$ as the elements of the $\mathbb(Q)$ as a $\mathbb(Z)$-module would presumably be of the form $n \cdot \frac{a}{b}$ ... but ... then (?) on the other hand (?) ... ... $n \cdot \frac{a}{b}$ just gives us another "fraction" ... that is another member of $\mathbb(Q)$ ... ...

Hope you can clarify this issue for me ...

Peter

We consider $\begin{bmatrix}0 & S_i \\ 0 & 0\end{bmatrix}$ as $R = \begin{bmatrix} \mathbb{Z} & \mathbb{Q} \\ 0 & \mathbb{Q}\end{bmatrix}$ left modules.

But multiplication from left means we have $\mathbb{Z} \cdot S_i \subseteq S_i \subseteq \mathbb{Q}$. So the $S_i \subseteq \mathbb{Q}$ are left $\mathbb{Z}$-modules by their construction. The requirement $s_i \cdot r \in \begin{bmatrix}0 & S_i \\ 0 & 0\end{bmatrix} \; (s_i \in \begin{bmatrix}0 & S_i \\ 0 & 0\end{bmatrix} \;,\; r\in R)$ forces the fact that the $S_i$ have to be left $\mathbb{Z}$-modules.

And yes, $n \cdot \frac{a}{b} \in \mathbb{Q}$. So? That's the $\mathbb{Z}$-module property of the $S_i$ which is inherited by the $R$-module property of the $S_i$-matrices $\begin{bmatrix}0 & S_i \\ 0 & 0\end{bmatrix}$.

The point is that we get an ascending chain of $R$-modules that does not end:
$$ \begin{bmatrix} 0 & \mathbb{Z} \cdot 2^{-1} \\ 0 & 0 \end{bmatrix} \subsetneq \begin{bmatrix} 0 & \mathbb{Z} \cdot 2^{-2} \\ 0 & 0 \end{bmatrix} \subsetneq \begin{bmatrix} 0 & \mathbb{Z} \cdot 2^{-3} \\ 0 & 0 \end{bmatrix} \subsetneq \ldots \subsetneq \begin{bmatrix} \mathbb{Z} & \mathbb{Q} \\ 0 & \mathbb{Q} \end{bmatrix} =: R $$
We define them in this way. All in $R \,$! And one such chain is sufficient, but any other prime will do as well.

Edit: If we replace all $\mathbb{Z}$ by $\mathbb{Q}$ then the example breaks down as all inclusions become equalities. But then we would talk about another ring.

 

[Math Amateur]

Thanks so much for the further assistance ... most helpful!

I think I'm closer to understanding things now ... but still reflecting ...

Thanks again ...

Peter

 

[fresh_42]

Correction to post #5: It has to be $r\,\cdot\,s_i \in \begin{bmatrix}0 & S_i \\ 0 & 0\end{bmatrix}$ since we consider left modules here. It doesn't work for right modules.
Sorry.

 

[andrewkirk]

Any commutative ring $R$ can be considered as either a $R$-module $R_R$ or a $\mathbb Z$-module $R_{\mathbb Z}$. In the former, the scalar product $rm$ where $r$ is in $R$ as ring and $m$ is in $R$ as module, is the module element corresponding to the ring element $rm$ (calculated using ring multiplication). In the latter, the scalar product $nm$ with $n\in\mathbb Z$ means $m+m+...+m$ with $n$ terms.

$R_{\mathbb Z}$ in general has a richer set of submodules than $R_R$. In fact if $R$ is a field then the only submodules of $R_R$ are {0} and $R_R$. Can you figure out why?

 

[Math Amateur]

Any commutative ring $R$ can be considered as either a $R$-module $R_R$ or a $\mathbb Z$-module $R_{\mathbb Z}$. In the former, the scalar product $rm$ where $r$ is in $R$ as ring and $m$ is in $R$ as module, is the module element corresponding to the ring element $rm$ (calculated using ring multiplication). In the latter, the scalar product $nm$ with $n\in\mathbb Z$ means $m+m+...+m$ with $n$ terms.

$R_{\mathbb Z}$ in general has a richer set of submodules than $R_R$. In fact if $R$ is a field then the only submodules of $R_R$ are {0} and $R_R$. Can you figure out why?

Andrew ... you ask ... " ... ... In fact if $R$ is a field then the only submodules of $R_R$ are {0} and $R_R$. Can you figure out why? "

Basically that is because if an ideal contains a unit then it is necessarily equal to the whole ring ... and all elements of a field, except 0, are units ...

Peter

 

[fresh_42]

Andrew ... you ask ... " ... ... In fact if $R$ is a field then the only submodules of $R_R$ are {0} and $R_R$. Can you figure out why? "

Basically that is because if an ideal contains a unit then it is necessarily equal to the whole ring ... and all elements of a field, except 0, are units ...

Peter

This is also the reason, why the example above, regarded as right modules leads to a different result.
The multiplication $s_i\cdot r$ becomes $S_i \cdot \mathbb{Q}$.