Ben-tanglement
- 6
- 0
- TL;DR Summary
- The Bohm interpretation of a Mach–Zehnder interferometer in "particle mode" (1 beam splitter) swaps paths taken to D1 & D2, relative to the standard QM interpretation. An asymmetric beam splitter predicts different results for the 2 interpretations
Background
I was reading a paper, Delayed Choice Experiments and the Bohm Approach by Basil Hiley and Robert Callaghan. The Wheeler's Delayed Choice experiment was explained in a way that was very easy to understand. An interesting point in this paper is that when a Mach–Zehnder interferometer is in "particle mode" with 1 beam splitter, the Bohm interpretation says that the paths of the photons are swapped compared to the SQM (Standard Quantum Mechanics) interpretation.
See Figure 2 in the linked paper. The particle, a photon in this case, enters the apparatus from the lower left, and hits the only beam splitter, BS1, and is either reflected towards mirror M1 along Channel 1, or transmitted towards mirror M2 along Channel 2.
Hiley & Callaghan, section 3.1, Interferometer with BS2 removed:
The above description of the paths is the same as described in the Wikipedia on Wheeler's Delayed Choice Experiment. See the figure in the "Simple interferometer" section in the "open" position with one beam splitter. Using the terminology in Figure 2 of Hiley & Callaghan: If the photon is detected in D1 then the photon is said to have gone down channel 1 with mirror M1. If the photon is detected in D2 then the photon is said to have gone down channel 2 with mirror M2.
In the Bohm interpretation, the photon goes down either Channel 1 or Channel 2 (no superpositions), but the quantum potential goes equally down both channels. The region I2 (Figure 2), is of particular interest to this analysis. The quantum potential (pilot wave ripples?) traveling down both channels will interfere with each other. See Figure 3 for the Bohm trajectories within region I2, and Figure 5 for the overall Bohm trajectories.
The bold in the paragraph above is my emphasis. The conclusion is that the paths taken by photons are swapped in the Bohm interpretation compared to Standard Quantum Mechanics.
Experiment
Could there be any way to alter the Mach–Zehnder interferometer to distinguish between the two interpretations? Perhaps if the one beam splitter was non-symmetrical, let's say reflecting 52% of the time and transmitting 48% of the time (52-48) rather than 50-50, differences may emerge for the results predicted by the two interpretations.
Predictions are illustrated here with a Mach–Zehnder interferometer in "particle mode" with a non-symmetrical beam splitter:
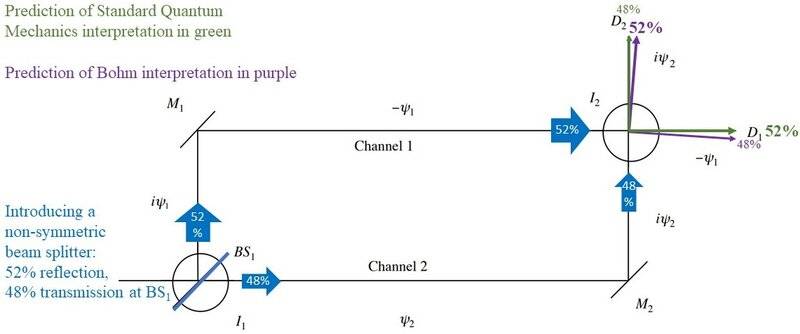
This is a modified version of Figure 2 from the paper.
Blue highlights my modification to the interferometer.
Green highlights the predictions of the Standard Quantum Mechanics interpretation.
Purple highlights the predictions of the Bohm interpretation.
In the Standard Quantum Mechanics interpretation, the 52% of photons reflected at BS1 should reflect off M1 and arrive at detector D1. The 48% of photons transmitted at BS1 should reflect off M2 and arrive at detector D2.
In the Bohm interpretation, the 52% of photons reflected at BS1, traveling with a stronger quantum potential, should reflect off M1, enter region I2, 'bounce off' the weaker quantum potential arriving from the lower path, then head towards D2 at an angle bent slightly towards D1. The 48% of photons transmitted at BS1, traveling with a weaker quantum potential, should reflect off M2, enter region I2, 'bounce off' the stronger quantum potential arriving from the upper path, then head towards D1 at an angle bent slightly towards M2.
If one could gradually increase the reflectivity of BS1 from 50% to 100%, the number of photons in Channel 1 would gradually increase from 50% to 100%, and would exit region I2 at an angle that initially points at D2 but gradually shifts towards pointing at D1. The number of photons in Channel 2 would gradually decrease from 50% to 0%, and would exit region I2 at an angle that initially points at D1 but gradually shits towards pointing back to M2.
I was reading a paper, Delayed Choice Experiments and the Bohm Approach by Basil Hiley and Robert Callaghan. The Wheeler's Delayed Choice experiment was explained in a way that was very easy to understand. An interesting point in this paper is that when a Mach–Zehnder interferometer is in "particle mode" with 1 beam splitter, the Bohm interpretation says that the paths of the photons are swapped compared to the SQM (Standard Quantum Mechanics) interpretation.
See Figure 2 in the linked paper. The particle, a photon in this case, enters the apparatus from the lower left, and hits the only beam splitter, BS1, and is either reflected towards mirror M1 along Channel 1, or transmitted towards mirror M2 along Channel 2.
Hiley & Callaghan, section 3.1, Interferometer with BS2 removed:
Let us begin by first quickly recalling the SQM treatment of the delayed choice experiment. When BS2 is removed...If BS1 is a 50/50 beam splitter, then each particle entering the interferometer will have a 50% chance of firing one of the detectors. This means that the device acts as a particle detector, because the particle will either take path 1, BS1M1D1, trigging the detector D1. Or it will travel down path 2, BS1M2D2, triggering detector D2.
The above description of the paths is the same as described in the Wikipedia on Wheeler's Delayed Choice Experiment. See the figure in the "Simple interferometer" section in the "open" position with one beam splitter. Using the terminology in Figure 2 of Hiley & Callaghan: If the photon is detected in D1 then the photon is said to have gone down channel 1 with mirror M1. If the photon is detected in D2 then the photon is said to have gone down channel 2 with mirror M2.
Now let us turn to consider how the BI [Bohm interpretation] analyses this experiment. Here we must construct an ensemble of trajectories, each individual trajectory corresponding to the possible initial values of position of the particle within the incident wave packet. One set of trajectories will follow the upper arm of the apparatus, while the others follow the lower arm.
In the Bohm interpretation, the photon goes down either Channel 1 or Channel 2 (no superpositions), but the quantum potential goes equally down both channels. The region I2 (Figure 2), is of particular interest to this analysis. The quantum potential (pilot wave ripples?) traveling down both channels will interfere with each other. See Figure 3 for the Bohm trajectories within region I2, and Figure 5 for the overall Bohm trajectories.
Here the wave packets from each channel overlap and there will be a region of interference because the two wave packets are coherent...The particles following the trajectories then ‘bounce off’ this potential as shown in figure 3 so that the particles in channel 1 end up triggering D2, while the trajectories in channel 2 end up triggering D1.
The bold in the paragraph above is my emphasis. The conclusion is that the paths taken by photons are swapped in the Bohm interpretation compared to Standard Quantum Mechanics.
Experiment
Could there be any way to alter the Mach–Zehnder interferometer to distinguish between the two interpretations? Perhaps if the one beam splitter was non-symmetrical, let's say reflecting 52% of the time and transmitting 48% of the time (52-48) rather than 50-50, differences may emerge for the results predicted by the two interpretations.
Predictions are illustrated here with a Mach–Zehnder interferometer in "particle mode" with a non-symmetrical beam splitter:
This is a modified version of Figure 2 from the paper.
Blue highlights my modification to the interferometer.
Green highlights the predictions of the Standard Quantum Mechanics interpretation.
Purple highlights the predictions of the Bohm interpretation.
In the Standard Quantum Mechanics interpretation, the 52% of photons reflected at BS1 should reflect off M1 and arrive at detector D1. The 48% of photons transmitted at BS1 should reflect off M2 and arrive at detector D2.
In the Bohm interpretation, the 52% of photons reflected at BS1, traveling with a stronger quantum potential, should reflect off M1, enter region I2, 'bounce off' the weaker quantum potential arriving from the lower path, then head towards D2 at an angle bent slightly towards D1. The 48% of photons transmitted at BS1, traveling with a weaker quantum potential, should reflect off M2, enter region I2, 'bounce off' the stronger quantum potential arriving from the upper path, then head towards D1 at an angle bent slightly towards M2.
If one could gradually increase the reflectivity of BS1 from 50% to 100%, the number of photons in Channel 1 would gradually increase from 50% to 100%, and would exit region I2 at an angle that initially points at D2 but gradually shifts towards pointing at D1. The number of photons in Channel 2 would gradually decrease from 50% to 0%, and would exit region I2 at an angle that initially points at D1 but gradually shits towards pointing back to M2.