- #1
Richard Spiteri
- 38
- 10
I am still intrigued by the neutral buoyancy of a body and have come up with some other questions. As previously encouraged, I attempted to answer my own questions (this is not home work, it is just me trying to wake up my brain in my retirement).
Fig 1 has no question but builds on what @kuruman and others showed me; Fig 2 and Fig 3 build on my understanding with some questions that I tried to answer myself
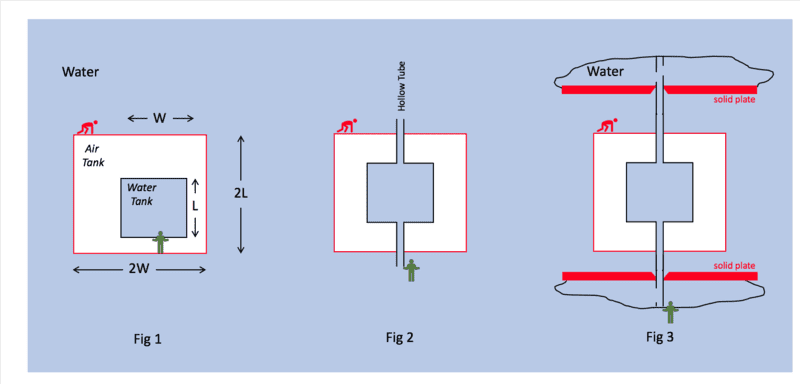

Fig 1 has no question but builds on what @kuruman and others showed me; Fig 2 and Fig 3 build on my understanding with some questions that I tried to answer myself
Last edited: